Home /
Expert Answers /
Advanced Math /
without-multiplying-matrices-find-bases-for-the-row-and-column-spaces-of-a-a-left-be-pa820
(Solved): Without multiplying matrices, find bases for the row and column spaces of \( A \) : \[ A=\left[\be ...
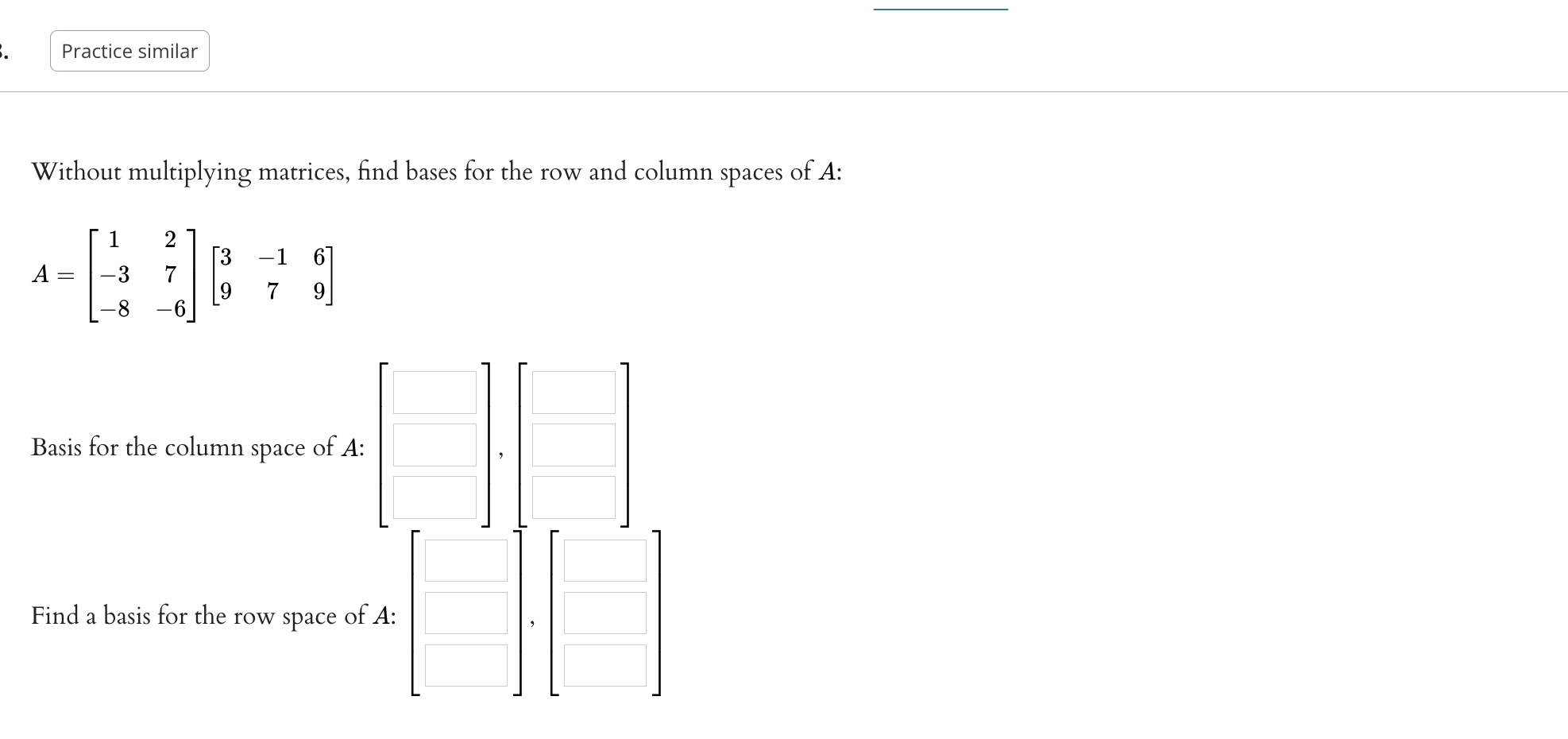
Without multiplying matrices, find bases for the row and column spaces of \( A \) : \[ A=\left[\begin{array}{cc} 1 & 2 \\ -3 & 7 \\ -8 & -6 \end{array}\right]\left[\begin{array}{ccc} 3 & -1 & 6 \\ 9 & 7 & 9 \end{array}\right] \] Basis for the column space of \( A \) : Find a basis for the row space of \( A \) :
Expert Answer
Let for given matrix A Maximum possible rank of (A) =2 Because we know rank(AB) <=rank(A) and rank(AB) <=r