(Solved): Using the hydrostatic equation, derive an expression for the pressure at the ...

???????

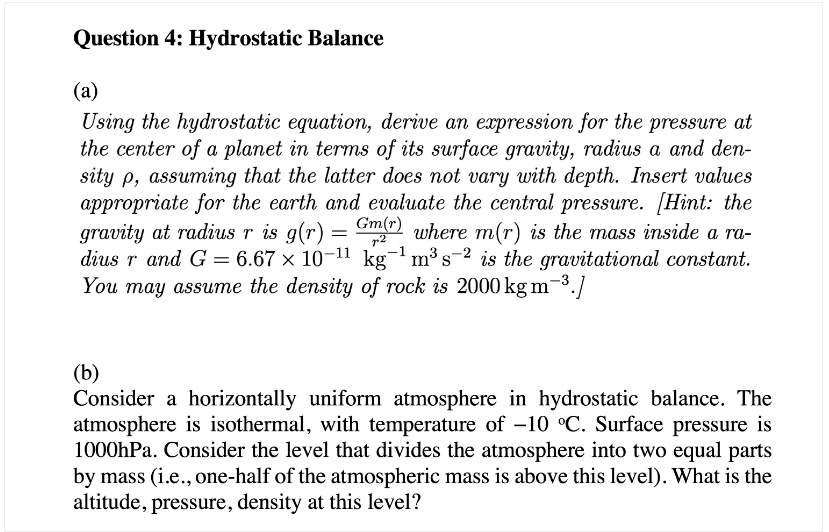
Using the hydrostatic equation, derive an expression for the pressure at the center of a planet in terms of its surface gravity, radius a and density $$?$$, assuming that the latter does not vary with depth. Insert values appropriate for the earth and evaluate the central pressure. [Hint: the gravity at radius $$r$$ is $$g(r)=\frac{Gm(r)}{r^2}$$ where $$m(r)$$ is the mass inside a radius $$r$$ and $$G=6.67\times 10^{?11}{\mathrm{kg}}^{r^2}{\mathrm{m}}^3{\mathrm{s}}^{?2}$$ is the gravitational constant. You may assume the density of rock is $$2000\mathrm{kg}{\mathrm{m}}^{?3}$$.] (b) Consider a horizontally uniform atmosphere in hydrostatic balance. The atmosphere is isothermal, with temperature of $$?10^{?}\mathrm{C}$$. Surface pressure is $$1000\mathrm{hPa}$$. Consider the level that divides the atmosphere into two equal parts by mass (i.e., one-half of the atmospheric mass is above this level). What is the altitude, pressure, density at this level?