(Solved): Use the superposition method to derive the equations of deflection for the plate shown in Fig 34 and ...

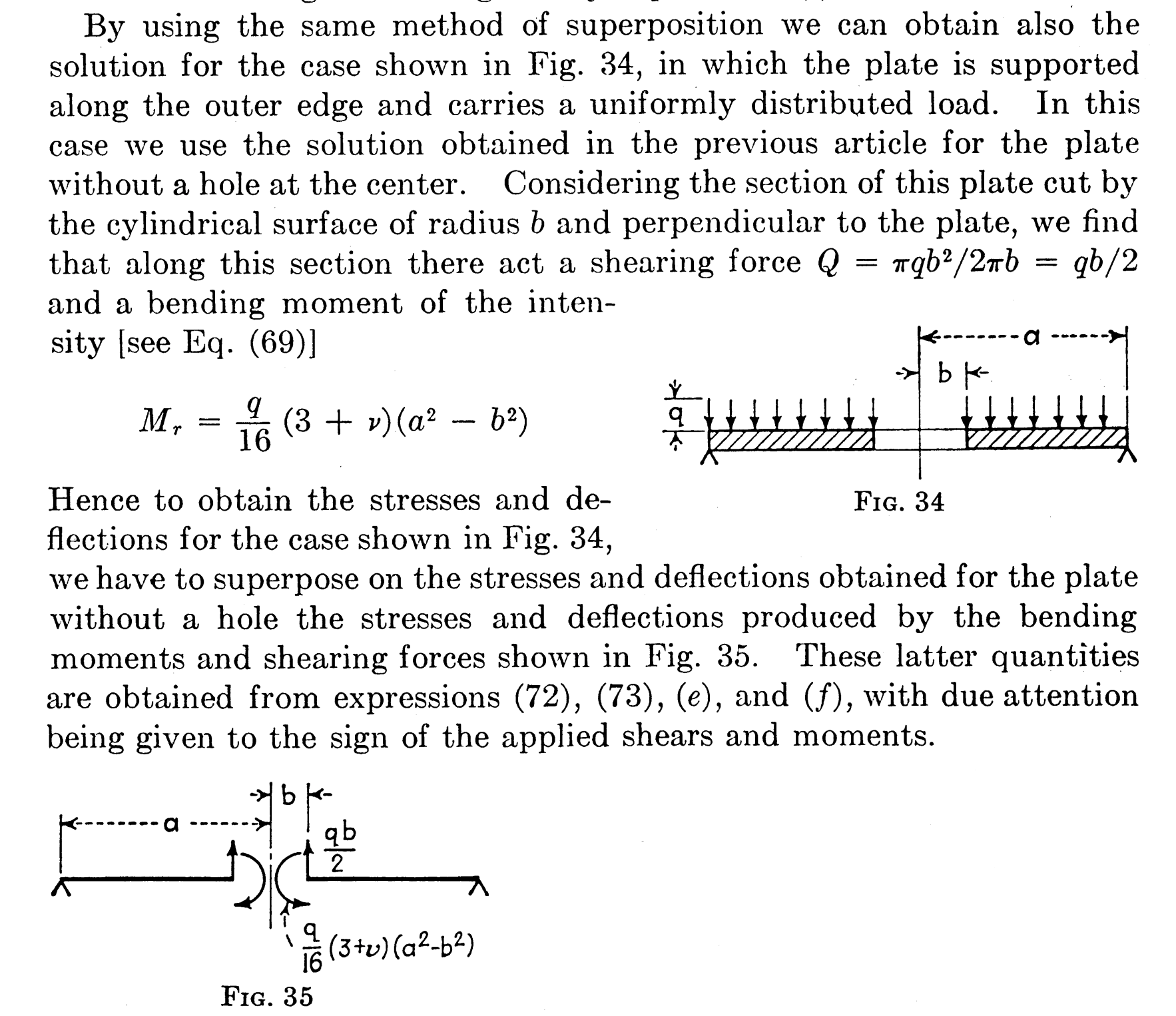
By using the same method of superposition we can obtain also the solution for the case shown in Fig. 34, in which the plate is supported along the outer edge and carries a uniformly distributed load. In this case we use the solution obtained in the previous article for the plate without a hole at the center. Considering the section of this plate cut by the cylindrical surface of radius \\( b \\) and perpendicular to the plate, we find that along this section there act a shearing force \\( Q=\\pi q b^{2} / 2 \\pi b=q b / 2 \\) and a bending moment of the intensity [see Eq. (69)] \\[ M_{r}=\\frac{q}{16}(3+\\nu)\\left(a^{2}-b^{2}\\right) \\] Hence to obtain the stresses and deflections for the case shown in Fig. 34, we have to superpose on the stresses and deflections obtained for the plate without a hole the stresses and deflections produced by the bending moments and shearing forces shown in Fig. 35. These latter quantities are obtained from expressions (72), (73), \\( (e) \\), and \\( (f) \\), with due attention being given to the sign of the applied shears and moments. HIG. 30