Home /
Expert Answers /
Calculus /
use-the-identity-grad-times-gradf-0-and-stokes-39-theorem-to-show-that-the-circulations-of-the-follo-pa826
(Solved): Use the identity grad\times gradf=0 and Stokes' Theorem to show that the circulations of the followi ...
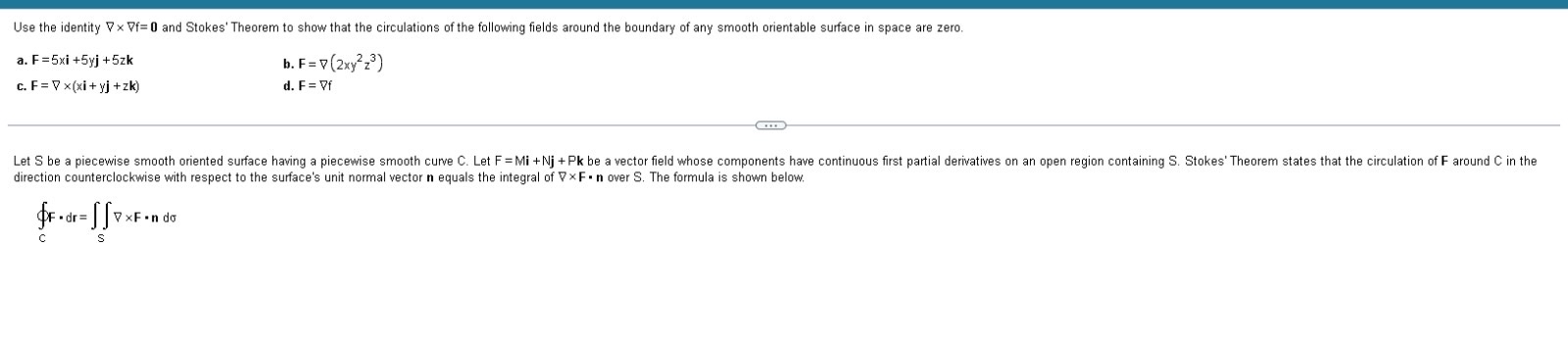
Use the identity
grad\times gradf=0and Stokes' Theorem to show that the circulations of the following fields around the boundary of any smooth orientable surface in space are zero. a.
F=5\xi +5yj+5zkb.
F=grad(2xy^(2)z^(3))c.
F=grad\times (\xi +yj+zk)d.
F=gradfdirection counterclockwise with respect to the surface's unit normal vector
nequals the integral of
grad\times F*nover S . The formula is shown below.
o\int_C F*dr=∬_(S)grad\times F*nd\sigma