(Solved): Transform the given initial value problem into an algebraic equation for \( Y=\mathcal{L}\{y\} \) ...

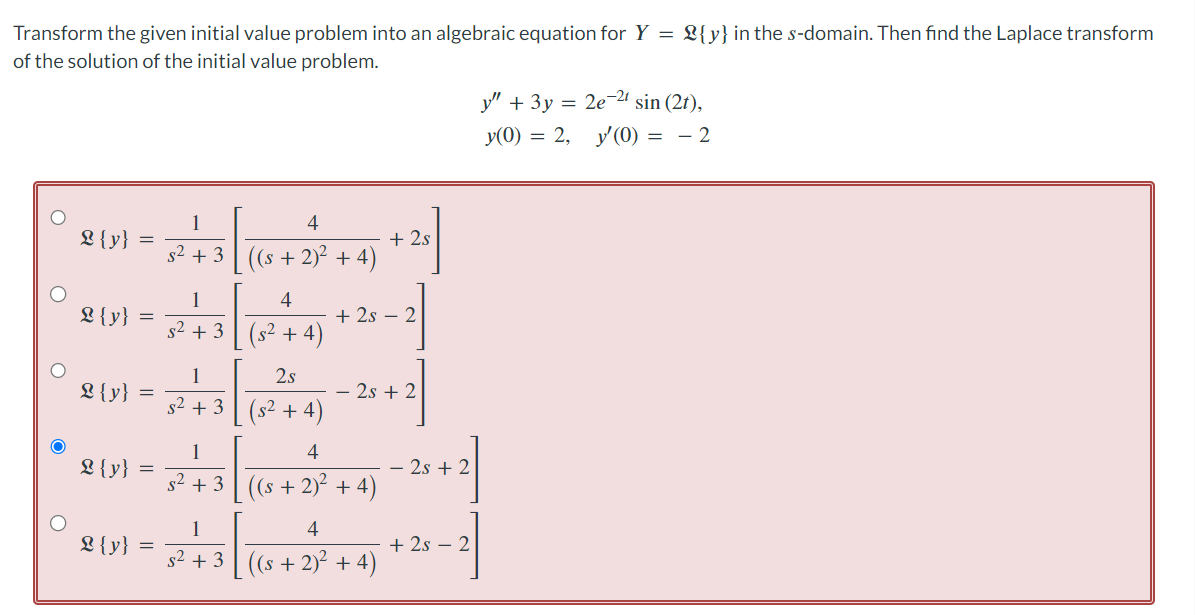
Transform the given initial value problem into an algebraic equation for \( Y=\mathcal{L}\{y\} \) in the \( s \)-domain. Then find the Laplace transform of the solution of the initial value problem. \[ \begin{array}{l} y^{\prime \prime}+3 y=2 e^{-2 t} \sin (2 t), \\ y(0)=2, \quad y^{\prime}(0)=-2 \end{array} \] \[ \begin{array}{l} \mathfrak{L}\{y\}=\frac{1}{s^{2}+3}\left[\frac{4}{\left((s+2)^{2}+4\right)}+2 s\right] \\ \mathfrak{L}\{y\}=\frac{1}{s^{2}+3}\left[\frac{4}{\left(s^{2}+4\right)}+2 s-2\right] \\ \mathfrak{L}\{y\}=\frac{1}{s^{2}+3}\left[\frac{2 s}{\left(s^{2}+4\right)}-2 s+2\right] \\ \mathfrak{Q}\{y\}=\frac{1}{s^{2}+3}\left[\frac{4}{\left((s+2)^{2}+4\right)}-2 s+2\right] \\ \mathfrak{L}\{y\}=\frac{1}{s^{2}+3}\left[\frac{4}{\left((s+2)^{2}+4\right)}+2 s-2\right] \end{array} \]