(Solved): This problem will illustrate the divergence theorem by corrputing the outward flux of the vector fi ...

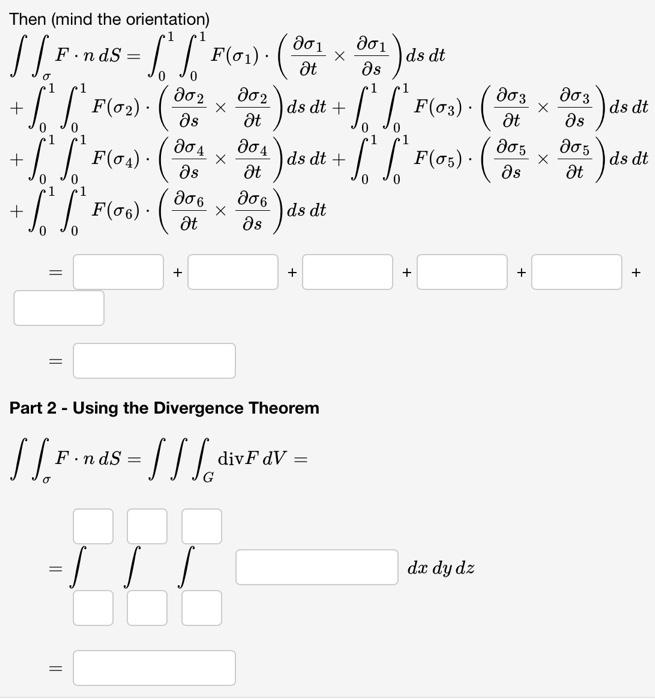
This problem will illustrate the divergence theorem by corrputing the outward flux of the vector field \( \mathbf{F}(x, y, z)=1 x \mathbf{i}+3 \mathbf{j}+4 z \mathrm{k} \) across the boundary of the right rectangular prism: \( -2 \leq x \leq 4,-2 \leq y \leq 3,-2 \leq z \leq 4 \) oriented outwards using a surface integral and a triple integral over the solid bounded by rectangular prism. Note: The vectors in this field point outwards from the origin, so we would expect the flux across each face of the prism to be positive. Part 1 - Using a Surface Integral First we parameterize the six faces using \( 0 \leq s \leq 1 \) and \( 0 \leq t \leq 1 \) : The face with \( z=-2: \sigma_{1}=\left(x_{1}(s), y_{1}(t), z_{1}(s, t)\right) \) \[ \begin{array}{l} x_{1}(s)= \\ y_{1}(t)= \\ z_{1}(s, t)=-2 \end{array} \] The face with \( z=4: \sigma_{2}=\left(x_{2}(s), y_{2}(t), z_{2}(s, t)\right) \) \[ \begin{array}{l} x_{2}(s)= \\ y_{2}(t)= \\ z_{2}(s, t)=4 \end{array} \] The face with \( \left.x=-2: \sigma_{3}=x_{3}(s, t), y_{3}(s), z_{3}(t)\right) \) \[ \begin{array}{l} x_{3}(s, t)=-2 \\ y_{3}(s)= \\ z_{3}(t)= \end{array} \] The face with \( \mathrm{x}=4: \sigma_{4}=\left(x_{4}(s, t), y_{4}(s), z_{4}(t)\right) \) \[ \begin{array}{l} x_{4}(s, t)=4 \\ y_{4}(s)= \\ z_{4}(t)= \end{array} \] The face with \( \mathrm{y}=-2: \sigma_{s}=\left(x_{5}(s), y_{s}(s, t), z_{5}(t)\right) \) \( x_{5}(s)= \) \( y_{5}(s, t)=-2 \) \( z_{5}(t)= \) The face with \( y=3: \sigma_{6}=\left(x_{6}(s), y_{0}(s, t), z_{6}(t)\right) \) \( x_{6}(s)= \) \( y_{6}(s, t)=3 \) \( z_{6}(t)= \) Then (mind the orientation) \[ \begin{array}{l} \iint_{\sigma_{0}} F \cdot n d S=\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{1}\right) \cdot\left(\frac{\partial \sigma_{1}}{\partial t} \times \frac{\partial \sigma_{1}}{\partial s}\right) d s d t \\ +\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{2}\right) \cdot\left(\frac{\partial \sigma_{2}}{\partial s} \times \frac{\partial \sigma_{2}}{\partial t}\right) d s d t+\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{3}\right) \cdot\left(\frac{\partial \sigma_{3}}{\partial t} \times \frac{\partial \sigma_{3}}{\partial s}\right) d s d t \\ +\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{4}\right) \cdot\left(\frac{\partial \sigma_{4}}{\partial s} \times \frac{\partial \sigma_{4}}{\partial t}\right) d s d t+\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{5}\right) \cdot\left(\frac{\partial \sigma_{5}}{\partial s} \times \frac{\partial \sigma_{5}}{\partial t}\right) d s d t \\ +\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{6}\right) \cdot\left(\frac{\partial \sigma_{6}}{\partial t} \times \frac{\partial \sigma_{6}}{\partial s}\right) d s d t \end{array} \] Then (mind the orientation) \[ \begin{array}{l} \iint_{\sigma} F \cdot n d S=\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{1}\right) \cdot\left(\frac{\partial \sigma_{1}}{\partial t} \times \frac{\partial \sigma_{1}}{\partial s}\right) d s d t \\ +\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{2}\right) \cdot\left(\frac{\partial \sigma_{2}}{\partial s} \times \frac{\partial \sigma_{2}}{\partial t}\right) d s d t+\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{3}\right) \cdot\left(\frac{\partial \sigma_{3}}{\partial t} \times \frac{\partial \sigma_{3}}{\partial s}\right) d s d \\ +\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{4}\right) \cdot\left(\frac{\partial \sigma_{4}}{\partial s} \times \frac{\partial \sigma_{4}}{\partial t}\right) d s d t+\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{5}\right) \cdot\left(\frac{\partial \sigma_{5}}{\partial s} \times \frac{\partial \sigma_{5}}{\partial t}\right) d s d \\ +\int_{0}^{1} \int_{0}^{1} F\left(\sigma_{6}\right) \cdot\left(\frac{\partial \sigma_{6}}{\partial t} \times \frac{\partial \sigma_{6}}{\partial s}\right) d s d t \end{array} \] Part 2 - Using the Divergence Theorem \[ \iint_{\sigma} F \cdot n d S=\iiint_{G} \operatorname{div} F d V= \] \[ =\iiint d x d y d z \]