Home /
Expert Answers /
Advanced Math /
this-is-discrete-math-nbsp-for-number-4-add-justification-10-let-a-n-pa420
(Solved): this is discrete math for number 4 add justification 10. Let \( a_{n} ...
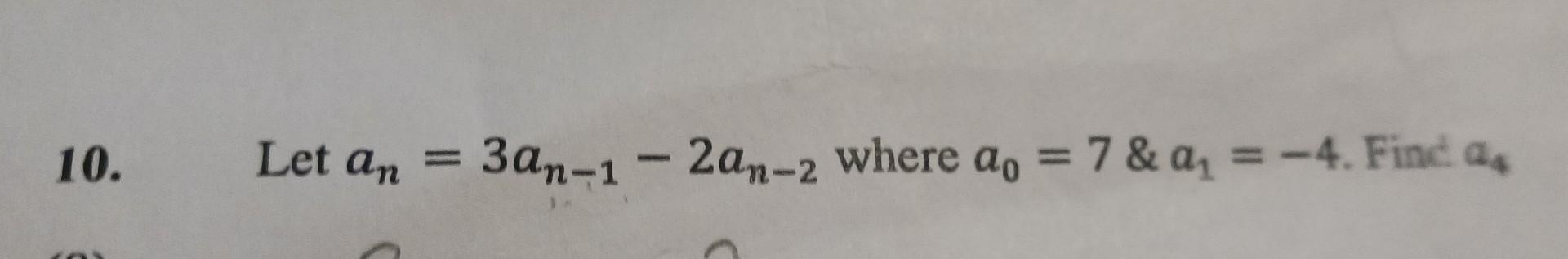
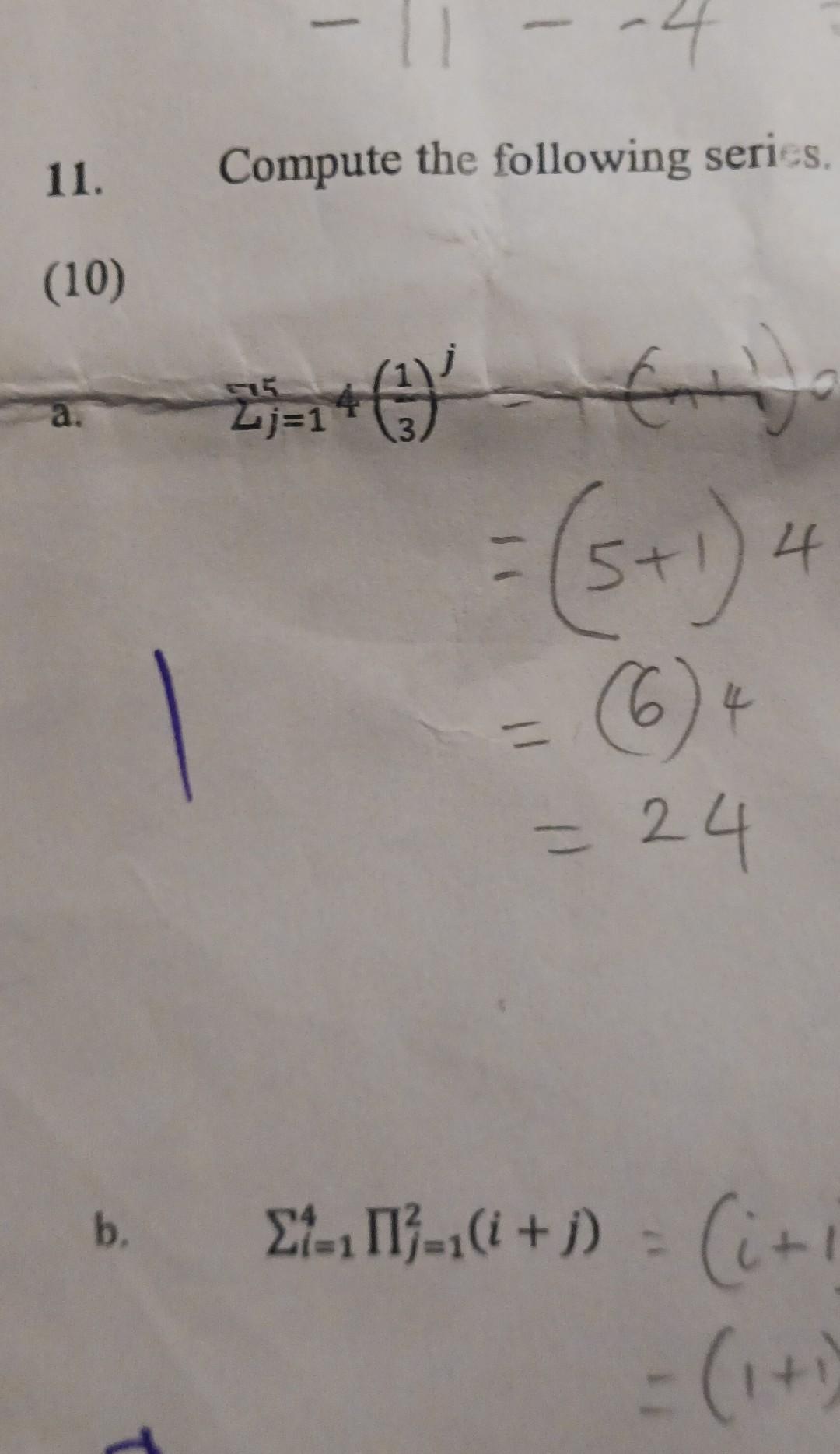
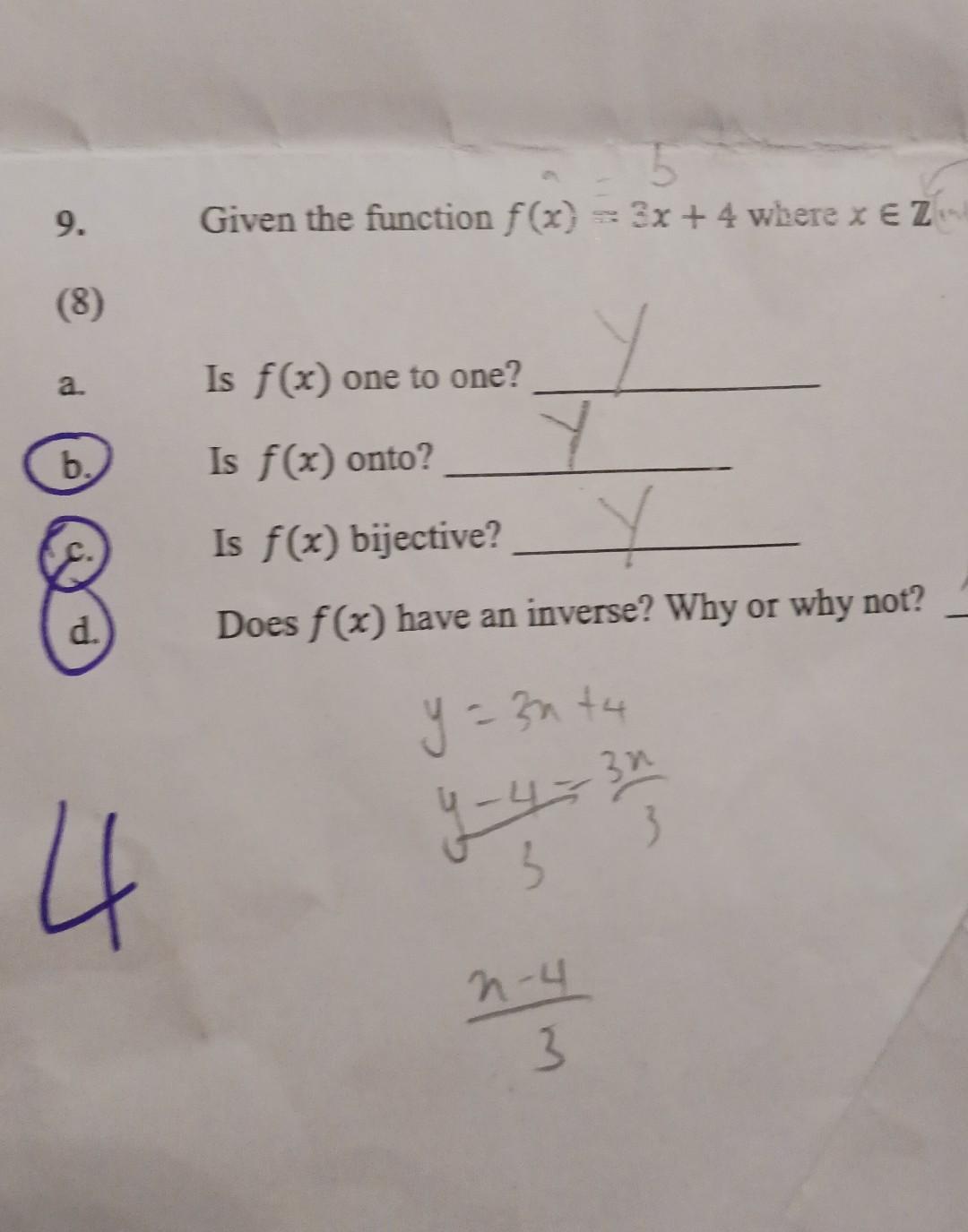
this is discrete math
![4. Use logical equivalences to simplify.
(8)
Steps
\[
\neg(p \wedge \neg q) \wedge(\neg q \rightarrow p)
\]](https://media.cheggcdn.com/study/8f5/8f56fb57-fea2-43b3-ab8c-41e9b004a212/image.jpg)
for number 4 add justification
10. Let \( a_{n}=3 a_{n-1}-2 a_{n-2} \) where \( a_{0}=7 \& a_{1}=-4 \). Find \( a_{4} \)
11. Compute the following series, (10) b. \( \quad \sum_{i=1}^{4} \Pi_{j=1}^{2}(i+j)=(i+1 \)
9. Given the function \( f(x)=3 x+4 \) where \( x \in \mathbb{Z} \) (8) a. Is \( f(x) \) one to one? b. Is \( f(x) \) onto? (c.) Is \( f(x) \) bijective? d. Does \( f(x) \) have an inverse? Why or why not?
4. Use logical equivalences to simplify. (8) Steps \[ \neg(p \wedge \neg q) \wedge(\neg q \rightarrow p) \]
Expert Answer
10. Given relation is: an=3an?1?2an?2 And, a0=7anda1=?4. We need to find the term a4. So, put n=2: a2=3a1?2a0=3×(?4)?2×7 =?12?14=?26 put n=3: a3=3a2?2