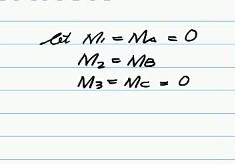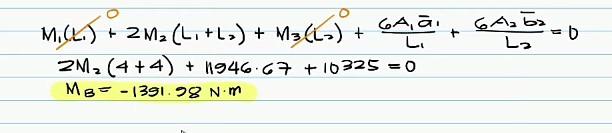Home /
Expert Answers /
Civil Engineering /
theory-of-structures-solve-the-problem-using-3-moment-equation-solve-the-step-by-step-pls-show-me-pa463
(Solved): theory of structures solve the problem using 3 moment equation solve the step by step pls show me ...
theory of structures solve the problem using 3 moment equation solve the step by step pls show me
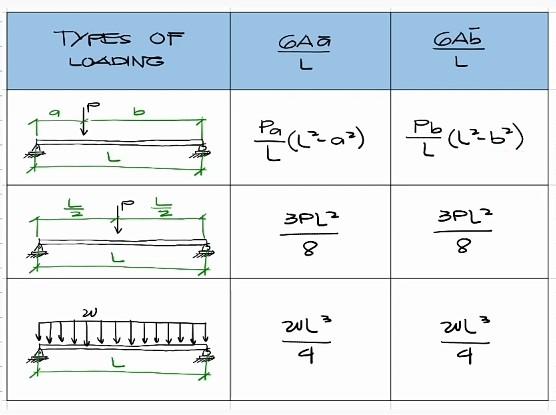
this is your guide and formula
solve the reaction support but same answer the question
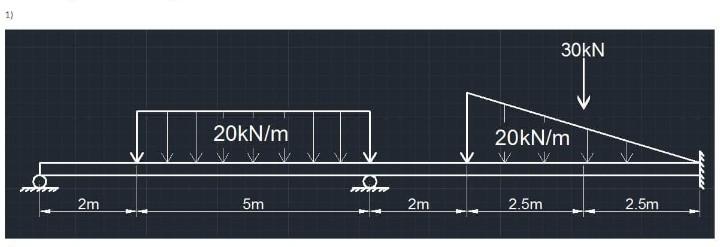
1) 2m 20kN/m 5m 2m 30KN 20kN/m 2.5m 2.5m www.
ANSWERS: 1) RA-23.8833 RB-114.408 RC-41.7087 Mc-56.45
TYPES OF LOADING b ? 20 I GAG GAL L ?(20) ?(46) ?(202) 3PL2 3PL2 8 8 ?? 4 3 L3
TYPES OF LOADING ? all 20 8 A5 L 60 7wL³ 0 Sweat 32 Gab L 7w1³ 60 83 20 ??? 32
TYPES OF LOADING Y L-X d a 20 • TILLF 7 b M a 20 minuma GAa L [6²(2l²-6³) - a² (2L²-a²)] ?S 3(x) ((²-x²) dx L - M (30²-L³) (?*- =7) (x²) xPP [~_Y (~) (²-x²) dx D
GAb 4 [d² (2L² ?²) - c² (22²= c)] je 3 (L-x) [(²-(L-Af Idx + M (36²-L²) [e(x-1) ?z7] (x-7) ×pa ??? L [y(L-x) [L²- (L-x)²] dx Pa ((²a²) Pb((²6²) b X P dx F 20 y P= ydx h
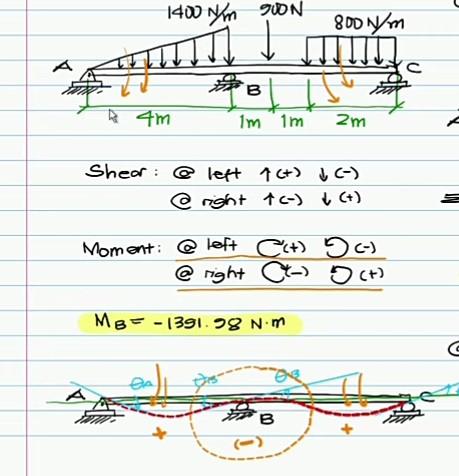
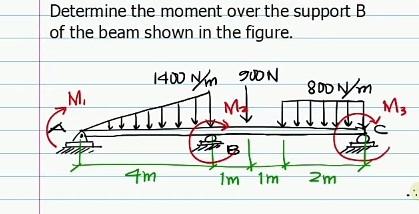
Determine the moment over the support B of the beam shown in the figure. 1400 N/M SOON m 800 N/m M I made M3 B 4m 1 1m 2m
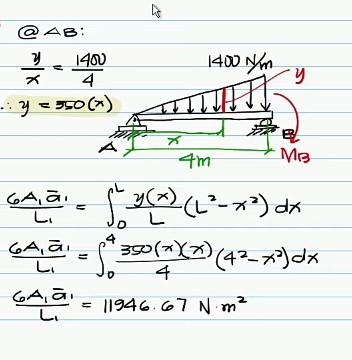
@B: ? 1400 ? 4 · : Y = 350 (x) A 4m MB GA, al 00:= 102(x) (12-13) dx ? L 4 GA, a 350 (xXx) (42_x2) dx = ? D 4 GA' = 11946.67 Nim2 ? 1400 ~m ?? X y
@BC: 900N 800 N/m MB mis I. y = 800 ? B em im 2m 4 ????? = ?² Y (L-X) [L ³² - (L-x)²] dx L? 2 - 2ND (3) (4²-3³) ??* (4~?) 6A? ba La + (800 (4-x) [4²-(4-x)²] dx = 10325 N·m²
let Mr = M? = 0 M? - Me M3 Mc = 0
M) + 2M (L) + Ms) + Aa + 6 2M? (4+4) + 11946.67 +10325 =0 Me= - 1391 92 N + 6 6 = b ??
A 1400 N/m 900N ? 300 800 N/m TIT ? 15 ôm 1m 4m 2m Shear @ left 1 (+) ? (-) @right tc-) & (+) Moment: @ left C'(+) 9 (-) @right - 0 (+) MB-1391.98 N.m G
@AB: 4m MB Av VB? MB=0C Av (4) - 1400 (4) (4/?) +1391 98 O E A = 2452.005 N 1 YB. = 347.595 N ? VB? 1400 N/m R?3 @BC 980 B Ms Im In VB? MB=O -Rc(4) Rc = VB? = 1 ZFV-0 ? (+) RB 1770.99 N ?
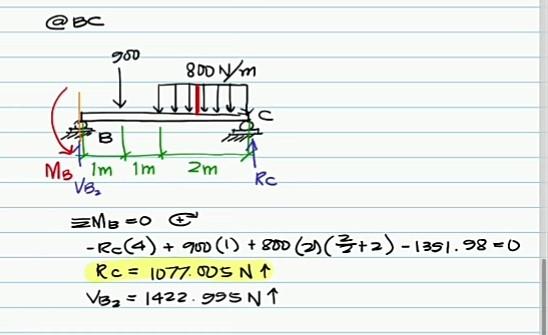
@BC 980 ? 800 N/m ? 2m Rc MB=0 G -Rc (4) + 900 (1) + 800 (2) (3+2) - 1351.98=0 Rc = 1077.005 N ? VB? = 1422.995 ?? B Me 1m 1m VB?


![TYPES OF
LOADING Y
L-X
d
a
20
• TILLF
7
b
M
a
20
minuma
GAa
L
[6²(2l²-6³) - a² (2L²-a²)]
?S 3(x) ((²-x²) dx
L
- M
(30²-L³)
(?](https://media.cheggcdn.com/study/d9c/d9cbb0d6-c6c8-4302-b0d2-7ffe48e560bc/image)
![GAb
4 [d² (2L² ?²) - c² (22²= c)]
je 3 (L-x) [(²-(L-Af Idx
+ M (36²-L²)
[e(x-1) ?z7] (x-7) ×pa
???
L
[y(L-x) [L²- (L-x)²] dx](https://media.cheggcdn.com/study/49c/49c3a011-b29e-4ed9-a8df-e4058cefc43b/image)
![@BC:
900N
800 N/m
MB
mis I.
y = 800
?
B
em im 2m
4
?????
= ?² Y (L-X) [L ³² - (L-x)²] dx
L?
2
- 2ND (3) (4²-3³)
??* (4~?)
6A?](https://media.cheggcdn.com/study/d57/d57eddf4-66c9-49cb-8bf7-cdd42e7044b4/image)