(Solved): The surface of a hill is modeled by the equation z = = (60 - 3x - 5y) m shown in the figure. I ...

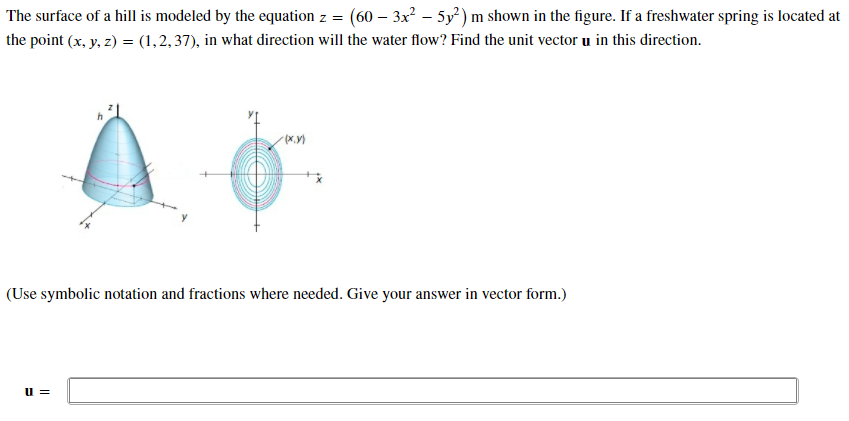
The surface of a hill is modeled by the equation z = = (60 - 3x² - 5y²) m shown in the figure. If a freshwater spring is located at the point (x, y, z) = (1,2,37), in what direction will the water flow? Find the unit vector u in this direction. (x,y) (Use symbolic notation and fractions where needed. Give your answer in vector form.) u= Find the directional derivative Du f(x, y) of the function f(x, y) = 8xy² + 7x² at the point (-1,2) and in the direction ??3. u = i + V?³j. (Use symbolic notation and fractions where needed.) Du f(-1,2)= Find the directional derivative Du f(x, y) of the function f(x, y) = 6xy + 3x² at the point (0, 3) and in the direction ( = (Express numbers in exact form. 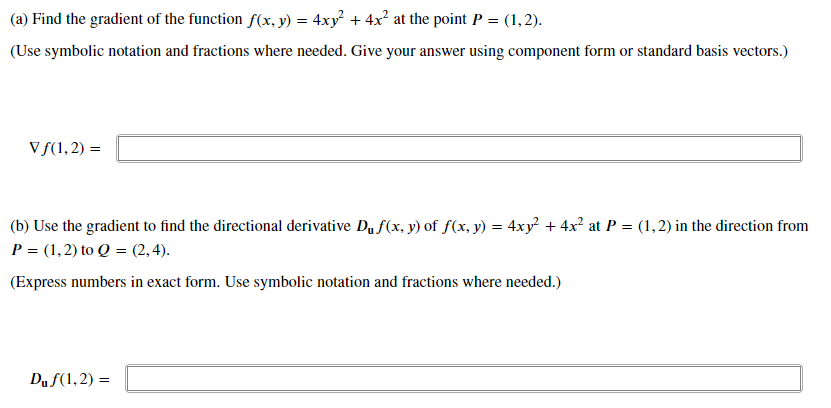Use symbolic notation and fractions where needed.) Du f(0, 3) = 4x (a) Find the gradient of the function f(x, y) = 4xy² + 4x² at the point P = (1,2). (Use symbolic notation and fractions where needed. Give your answer using component form or standard basis vectors.) Vƒ(1,2)= (b) Use the gradient to find the directional derivative Du f(x, y) of f(x, y) = 4xy² + 4x² at P = (1,2) in the direction from P = (1,2) to Q = (2,4). (Express numbers in exact form. 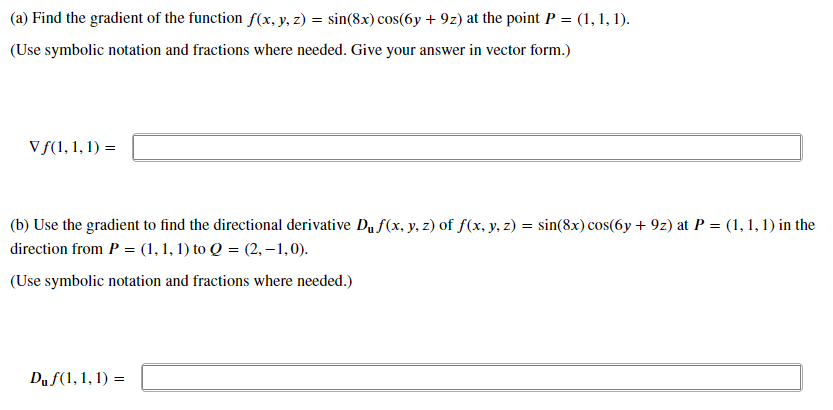Use symbolic notation and fractions where needed.) Du f(1,2)= (a) Find the gradient of the function f(x, y, z) = sin(8x) cos(6y +9z) at the point P = (1, 1, 1). (Use symbolic notation and fractions where needed. Give your answer in vector form.) Vf(1, 1, 1) = (b) Use the gradient to find the directional derivative Du f(x, y, z) of f(x, y, z) = sin(8x) cos(6y + 9z) at P = (1, 1, 1) in the direction from P = (1, 1, 1) to Q = (2,?1,0). (Use symbolic notation and fractions where needed.) Du f(1, 1, 1) =