Home /
Expert Answers /
Calculus /
the-quadric-surfaces-4-y-x-2-and-6-z-x-2-y-2-intersect-in-a-curve-parametrize-t-pa145
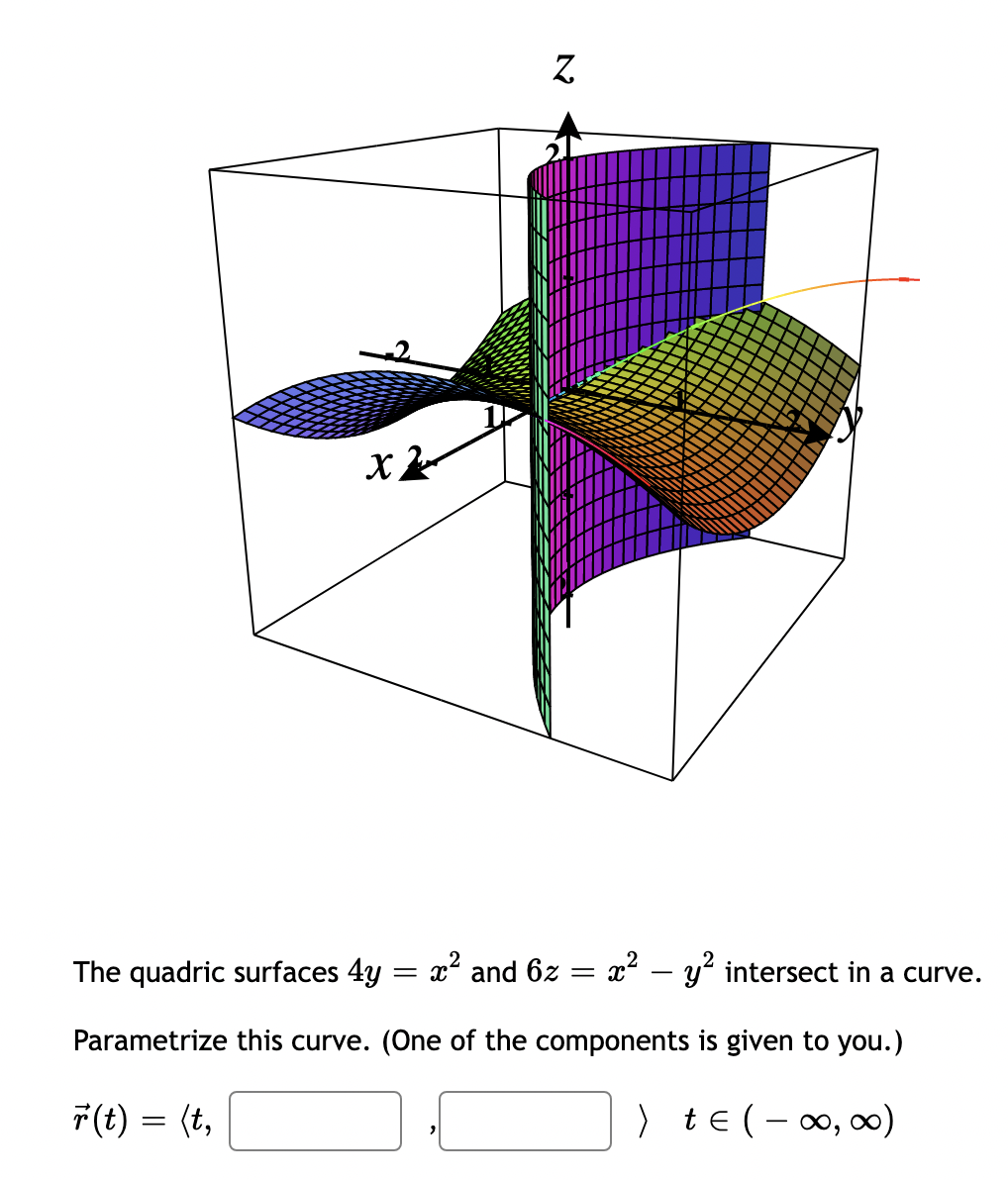
(Solved): The quadric surfaces \( 4 y=x^{2} \) and \( 6 z=x^{2}-y^{2} \) intersect in a curve. Parametrize t ...
The quadric surfaces \( 4 y=x^{2} \) and \( 6 z=x^{2}-y^{2} \) intersect in a curve. Parametrize this curve. (One of the components is given to you.) \[ \vec{r}(t)=\langle t, \quad, \quad\rangle t \in(-\infty, \infty) \]
Expert Answer
given quadric surfaces 4y=x2and6z=x