(Solved): The Poisson distribution is a discrete distribution that expresses the probability of a fixed number ...
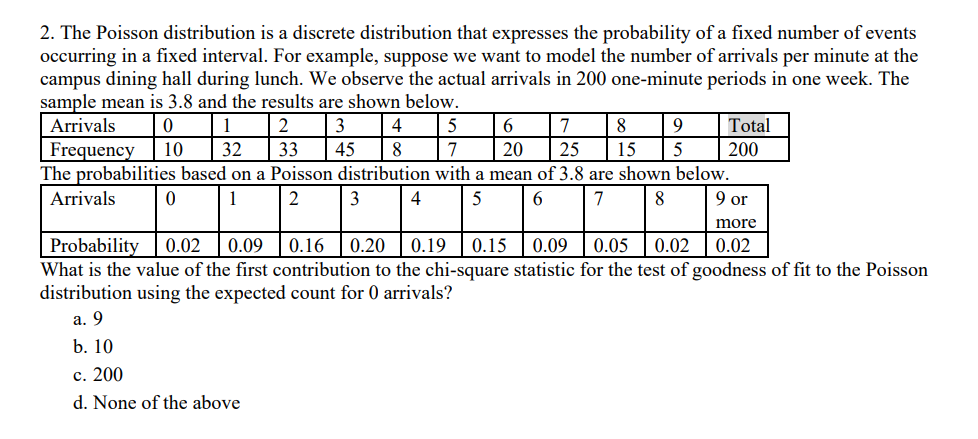
The Poisson distribution is a discrete distribution that expresses the probability of a fixed number of events occurring in a fixed interval. For example, suppose we want to model the number of arrivals per minute at the campus dining hall during lunch. We observe the actual arrivals in 200 one-minute periods in one week. The sample mean is 3.8 and the results are shown below. Arrivals 0 1 2 3 4 5 6 7 8 9 Total Frequency 10 32 33 45 8 7 20 25 15 5 200 The probabilities based on a Poisson distribution with a mean of 3.8 are shown below. Arrivals 0 1 2 3 4 5 6 7 8 9 or more Probability 0.02 0.09 0.16 0.20 0.19 0.15 0.09 0.05 0.02 0.02 What is the value of the first contribution to the chi-square statistic for the test of goodness of fit to the Poisson distribution using the expected count for 0 arrivals?
a.9
b.10
c.200
d. None of the above
Expert Answer
Solution: We need to calculate the chi square test statistic contribution fo