(Solved): The Maclaurin series expansion for \( \cos x \) is \[ \cos x=1-\frac{x^{2}}{2}+\frac{x^{4}}{4 !}-\f ...

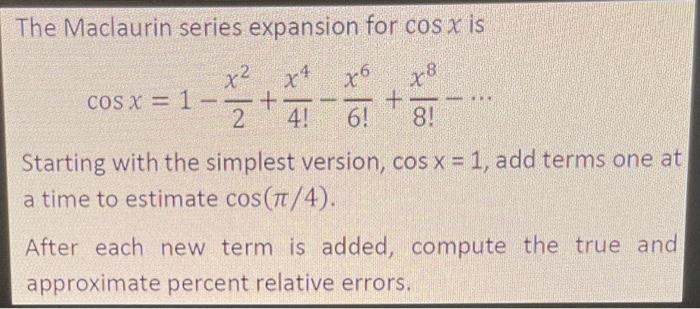
The Maclaurin series expansion for \( \cos x \) is \[ \cos x=1-\frac{x^{2}}{2}+\frac{x^{4}}{4 !}-\frac{x^{6}}{6 !}+\frac{x^{8}}{8 !}-\cdots \] Starting with the simplest version, \( \cos x=1 \), add terms one at a time to estimate \( \cos (\pi / 4) \). After each new term is added, compute the true and approximate percent relative errors.