(Solved): The following table contains the number of successes and failures for three categories of a variable ...

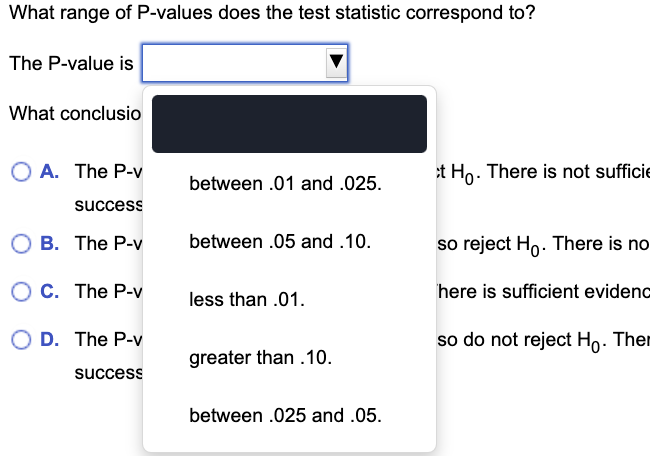
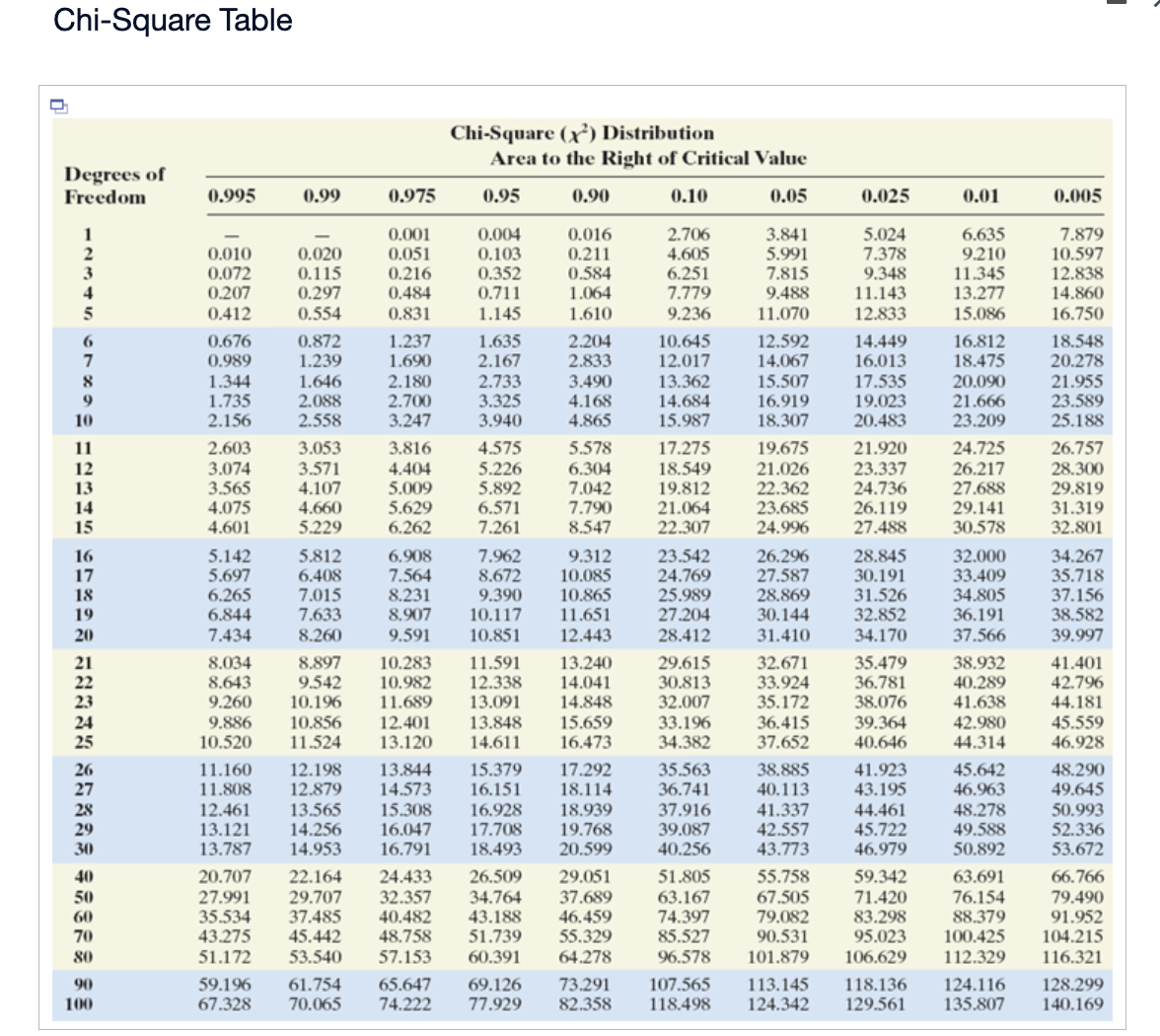
The following table contains the number of successes and failures for three categories of a variable. Test whether the proportions are equal for each category at the \\( \\alpha=0.01 \\) level of significance. Click the icon to view the Chi-Square table of critical values. State the hypotheses. Choose the correct answer below. A. \\( \\mathrm{H}_{0} \\) : The categories of the variable and success and failure are dependent. \\( \\mathrm{H}_{1} \\) : The categories of the variable and success and failure are independent. B. \\( H_{0}: \\mu_{1}=E_{1} \\) and \\( \\mu_{2}=E_{2} \\) and \\( \\mu_{3}=E_{3} \\) \\( \\mathrm{H}_{1} \\) : At least one mean is different from what is expected. C. \\( \\mathrm{H}_{0} \\) : The categories of the variable and success and failure are independent. \\( \\mathrm{H}_{1} \\) : The categories of the variable and success and failure are dependent. D. \\( H_{0}: p_{1}=p_{2}=p_{3} \\) \\( \\mathrm{H}_{1} \\) : At least one of the proportions is different from the others. Compute the value of the chi-square test statistic. \\[ \\chi_{0}^{2}=\\quad \\text { (Round to three decimal places as needed.) } \\]\r\nWhat range of \\( \\mathrm{P} \\)-values does the test statistic correspond to? he P-value is What conclusio A. The P-v success B. The P-v C. The P-v D. The P-v success between .01 and .025 . between .05 and .10 . less than 001 . greater than 10 . :t \\( \\mathrm{H}_{0} \\). There is not suffici so reject \\( \\mathrm{H}_{0} \\). There is no here is sufficient evidenc so do not reject \\( \\mathrm{H}_{0} \\). The between .025 and .05 .\r\nChi-Square Table