(Solved): The clamped-free cylindrical pressure vessel shown below is subjected to a combined internal press ...

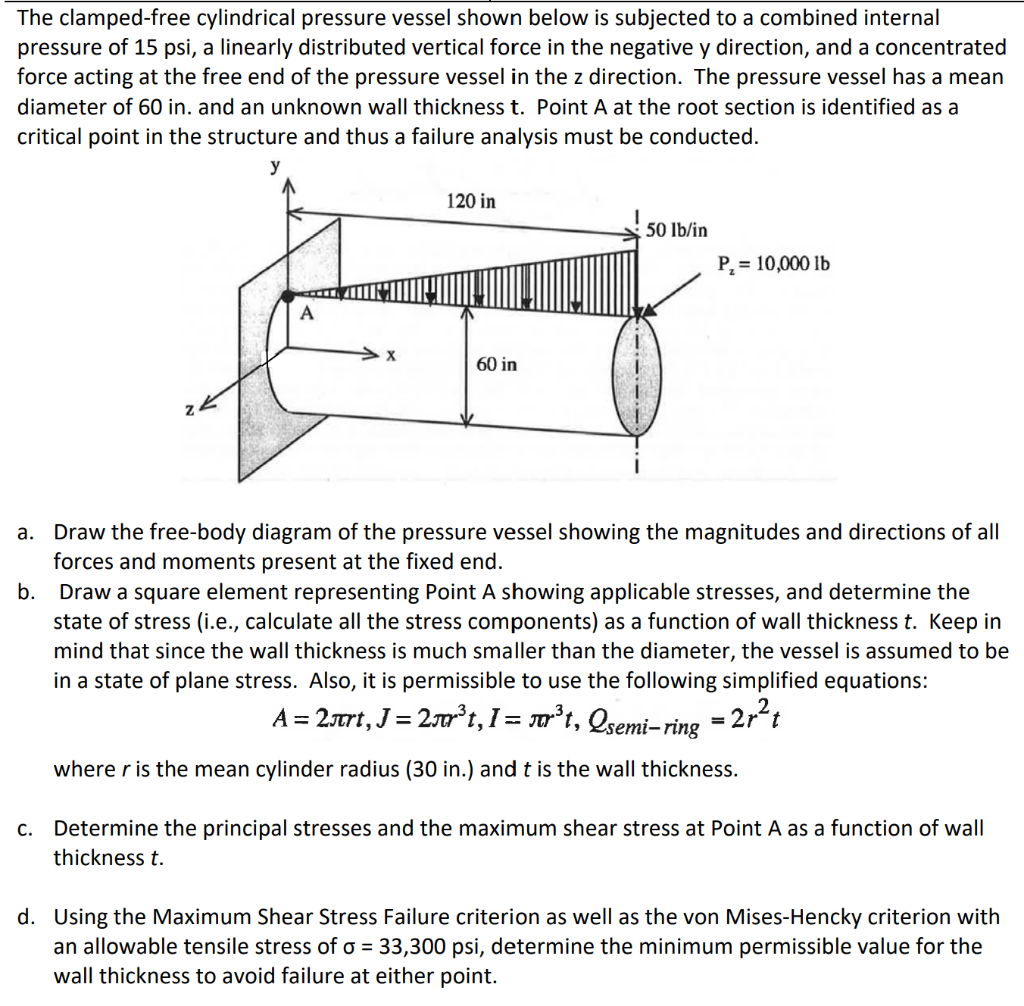
The clamped-free cylindrical pressure vessel shown below is subjected to a combined internal pressure of 15 psi, a linearly distributed vertical force in the negative y direction, and a concentrated force acting at the free end of the pressure vessel in the \( z \) direction. The pressure vessel has a mean diameter of 60 in. and an unknown wall thickness \( t \). Point \( A \) at the root section is identified as a critical point in the structure and thus a failure analysis must be conducted. a. Draw the free-body diagram of the pressure vessel showing the magnitudes and directions of all forces and moments present at the fixed end. b. Draw a square element representing Point A showing applicable stresses, and determine the state of stress (i.e., calculate all the stress components) as a function of wall thickness \( t \). Keep in mind that since the wall thickness is much smaller than the diameter, the vessel is assumed to be in a state of plane stress. Also, it is permissible to use the following simplified equations: \[ A=2 \pi r t, J=2 \pi r^{3} t, I=\pi r^{3} t, Q_{\text {semi-ring }}=2 r^{2} t \] where \( r \) is the mean cylinder radius ( 30 in.) and \( t \) is the wall thickness. c. Determine the principal stresses and the maximum shear stress at Point \( \mathrm{A} \) as a function of wall thickness \( t \). d. Using the Maximum Shear Stress Failure criterion as well as the von Mises-Hencky criterion with an allowable tensile stress of \( \sigma=33,300 \) psi, determine the minimum permissible value for the wall thickness to avoid failure at either point.