(Solved): Stokes Theorem A unitorm magnetic field \( \mathbf{B} \) has constant strength \( b \) teslas in the ...

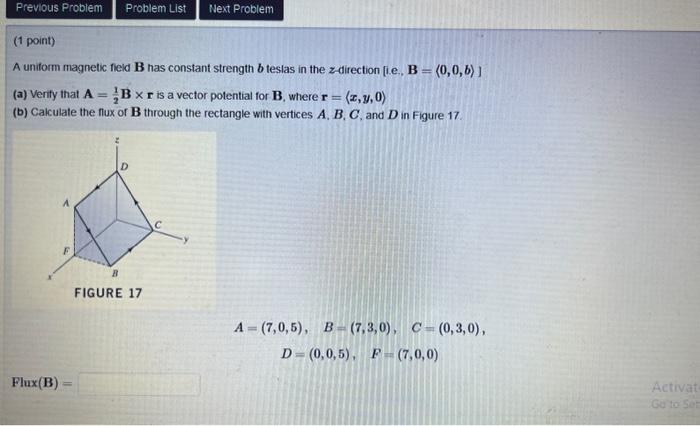
A unitorm magnetic field \( \mathbf{B} \) has constant strength \( b \) teslas in the \( z \)-direction \( [i . e, \mathbf{B}=\langle 0,0, b\rangle] \) (a) Verify that \( \mathbf{A}=\frac{1}{2} \mathbf{B} \times \mathbf{r} \) is a vector potential for \( \mathbf{B} \), where \( \mathbf{r}=\langle x, y, 0\rangle \) (b) Calculate the fux of \( \mathbf{B} \) through the rectangle with vertices \( A, B, C \), and \( D \) in Figure 17. \[ \begin{array}{c} A=(7,0,5), \quad B=(7,3,0), \quad C=(0,3,0), \\ D=(0,0,5), \quad F=(7,0,0) \end{array} \]