(Solved): Set the number of flips per sample to 30 , and choose variable 19. Click Reveal P to see the popula ...

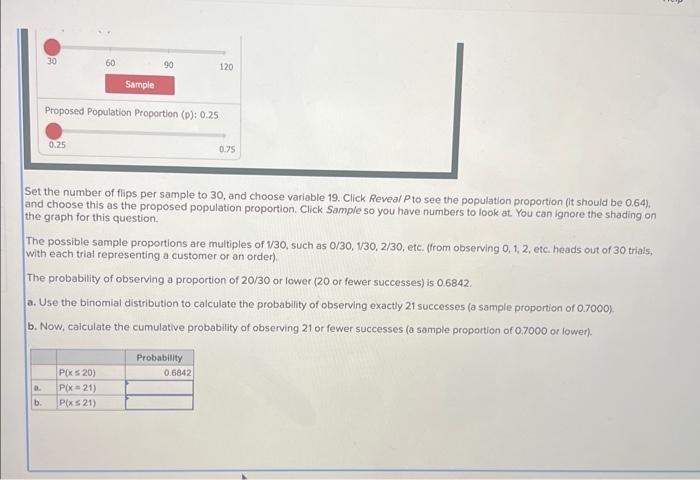
Set the number of flips per sample to 30 , and choose variable 19. Click Reveal P to see the population proportion (it should be \( 0.64 \) ). and choose this as the proposed population proportion. Click Sample so you have numbers to look at You can ignore the shading on the graph for this question. The possible sample proportions are multiples of \( 1 / 30 \), such as \( 0 / 30,1 / 30,2 / 30 \), etc. (from observing \( 0,1,2 \), etc. heads out of 30 trials, with each trial representing a customer or an order), The probability of observing a proportion of \( 20 / 30 \) or lower ( 20 or fewer successes) is \( 0.6842 \). a. Use the binomial distribution to calculate the probability of observing exactly 21 successes (a sample proportion of \( 0.7000 \) ). b. Now, calculate the cumulative probability of observing 21 or fewer successes (a sample proportion of \( 0.7000 \) or lower).