(Solved): Required information A chemical reaction is run 12 times, and the temperature \( x_{i}\left(\right. ...

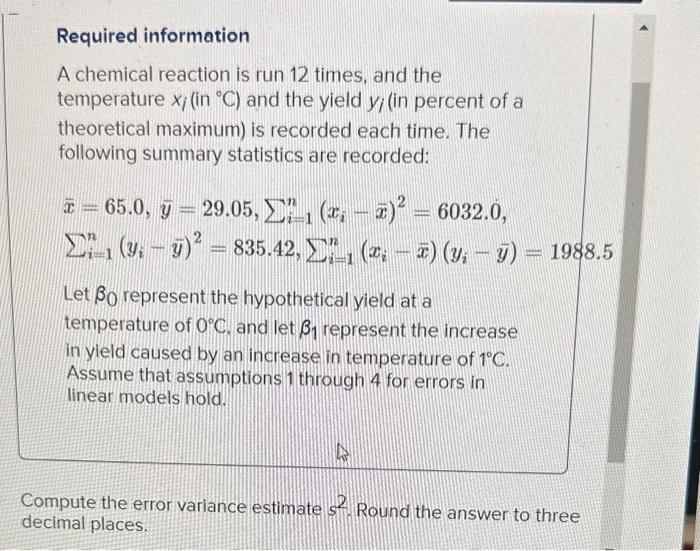
Required information A chemical reaction is run 12 times, and the temperature \( x_{i}\left(\right. \) in \( ^{\circ} \mathrm{C} \) ) and the yield \( y_{i} \) (in percent of a theoretical maximum) is recorded each time. The following summary statistics are recorded: \[ \begin{array}{l} \bar{x}=65.0, \bar{y}=29.05, \sum_{i=1}^{n}\left(x_{i}-\bar{x}\right)^{2}=6032.0 \\ \sum_{i=1}^{n}\left(y_{i}-\bar{y}\right)^{2}=835.42, \sum_{i=1}^{n}\left(x_{i}-\bar{x}\right)\left(y_{i}-\bar{y}\right)=1988.5 \end{array} \] Let \( \beta_{0} \) represent the hypothetical yield at a temperature of \( 0^{\circ} \mathrm{C} \). and let \( \beta_{1} \) represent the increase in yield caused by an increase in temperature of \( 1^{\circ} \mathrm{C} \). Assume that assumptions 1 through 4 for errors in linear models hold. Compute the error variance estimate \( s^{2} \). Round the answer to three decimal places.