(Solved): Questions 1. The LTI system is described by its difference equation below. \[ y(n)=3 / 4 y(n-1)-1 ...

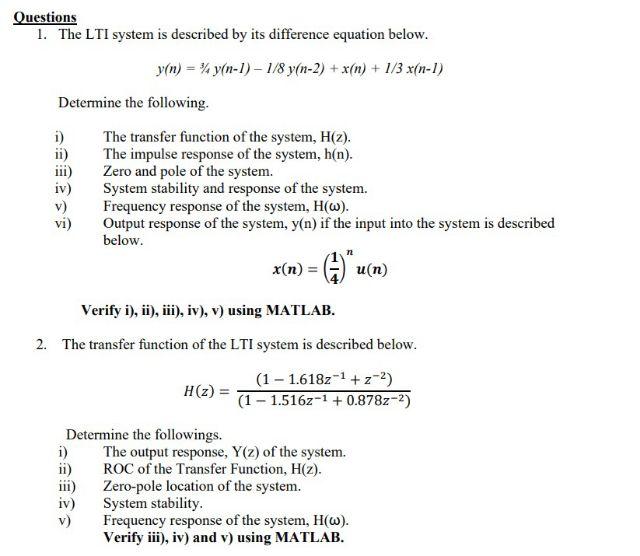
Questions 1. The LTI system is described by its difference equation below. \[ y(n)=3 / 4 y(n-1)-1 / 8 y(n-2)+x(n)+1 / 3 x(n-1) \] Determine the following. i) The transfer function of the system, \( \mathrm{H}(\mathrm{z}) \). ii) The impulse response of the system, h(n). iii) Zero and pole of the system. iv) System stability and response of the system. v) Frequency response of the system, \( \mathrm{H}(\omega) \). vi) Output response of the system, \( y(n) \) if the input into the system is described below. \[ x(n)=\left(\frac{1}{4}\right)^{n} u(n) \] Verify i), ii), iii), iv), v) using MATLAB. 2. The transfer function of the LTI system is described below. \[ H(z)=\frac{\left(1-1.618 z^{-1}+z^{-2}\right)}{\left(1-1.516 z^{-1}+0.878 z^{-2}\right)} \] Determine the followings. i) The output response, \( Y(z) \) of the system. ii) ROC of the Transfer Function, H(z). iii) Zero-pole location of the system. iv) System stability. v) Frequency response of the system, \( \mathrm{H}(\omega) \). Verify iii), iv) and v) using MATLAB.