(Solved): Q3) A silicon detector has the geometry shown in the figure. Light illuminates the central part un ...

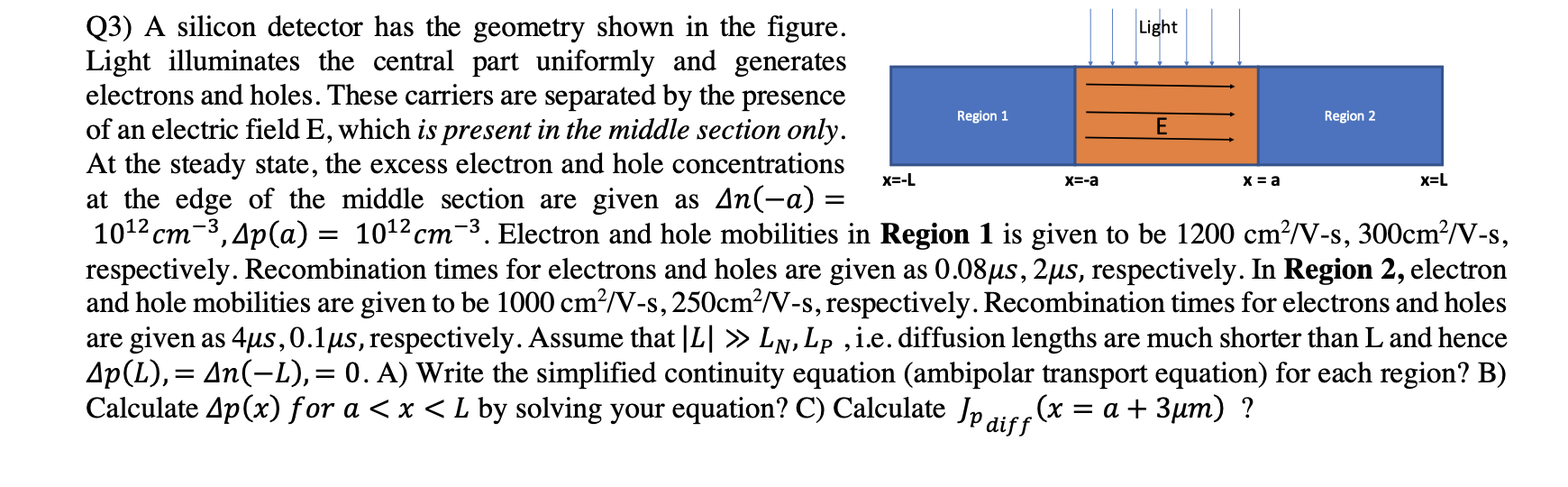
Q3) A silicon detector has the geometry shown in the figure. Light illuminates the central part uniformly and generates electrons and holes. These carriers are separated by the presence of an electric field \( \mathrm{E} \), which is present in the middle section only. At the steady state, the excess electron and hole concentrations at the edge of the middle section are given as \( \Delta n(-a)= \) \( 10^{12} \mathrm{~cm}^{-3}, \Delta p(a)=10^{12} \mathrm{~cm}^{-3} \). Electron and hole mobilities in Region 1 is given to be \( 1200 \mathrm{~cm}^{2} / \mathrm{V}-\mathrm{s}, 300 \mathrm{~cm}^{2} / \mathrm{V}-\mathrm{s} \), respectively. Recombination times for electrons and holes are given as \( 0.08 \mu s, 2 \mu s \), respectively. In Region 2, electron and hole mobilities are given to be \( 1000 \mathrm{~cm}^{2} / \mathrm{V}-\mathrm{s}, 250 \mathrm{~cm}^{2} / \mathrm{V}-\mathrm{s} \), respectively. Recombination times for electrons and holes are given as \( 4 \mu s, 0.1 \mu s \), respectively. Assume that \( |L| \gg L_{N}, L_{P} \), i.e. diffusion lengths are much shorter than \( L \) and hence \( \Delta p(L),=\Delta n(-L),=0 \). A) Write the simplified continuity equation (ambipolar transport equation) for each region? B) Calculate \( \Delta p(x) \) for \( a