(Solved): Problem \( 4.3 \) (7 points). Consider the Euclidean dot product. Let \( S \) be a subspace of \( ...

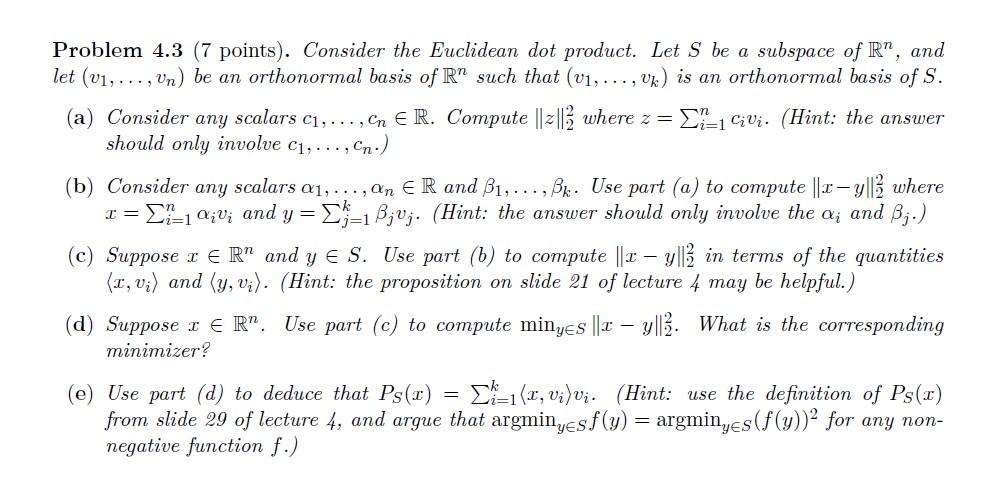
Problem \( 4.3 \) (7 points). Consider the Euclidean dot product. Let \( S \) be a subspace of \( \mathbb{R}^{n} \), and let \( \left(v_{1}, \ldots, v_{n}\right) \) be an orthonormal basis of \( \mathbb{R}^{n} \) such that \( \left(v_{1}, \ldots, v_{k}\right) \) is an orthonormal basis of \( S \). (a) Consider any scalars \( c_{1}, \ldots, c_{n} \in \mathbb{R} \). Compute \( \|z\|_{2}^{2} \) where \( z=\sum_{i=1}^{n} c_{i} v_{i} \). (Hint: the answer should only involve \( c_{1}, \ldots, c_{n} \).) (b) Consider any scalars \( \alpha_{1}, \ldots, \alpha_{n} \in \mathbb{R} \) and \( \beta_{1}, \ldots, \beta_{k} \). Use part (a) to compute \( \|x-y\|_{2}^{2} \) where \( x=\sum_{i=1}^{n} \alpha_{i} v_{i} \) and \( y=\sum_{j=1}^{k} \beta_{j} v_{j} \). (Hint: the answer should only involve the \( \alpha_{i} \) and \( \beta_{j} \).) (c) Suppose \( x \in \mathbb{R}^{n} \) and \( y \in S \). Use part (b) to compute \( \|x-y\|_{2}^{2} \) in terms of the quantities \( \left\langle x, v_{i}\right\rangle \) and \( \left\langle y, v_{i}\right\rangle \). (Hint: the proposition on slide 21 of lecture 4 may be helpful.) (d) Suppose \( x \in \mathbb{R}^{n} \). Use part (c) to compute \( \min _{y \in S}\|x-y\|_{2}^{2} \). What is the corresponding minimizer? (e) Use part (d) to deduce that \( P_{S}(x)=\sum_{i=1}^{k}\left\langle x, v_{i}\right\rangle v_{i} \). (Hint: use the definition of \( P_{S}(x) \) from slide 29 of lecture 4, and argue that \( \operatorname{argmin}_{y \in S} f(y)=\operatorname{argmin}_{y \in S}(f(y))^{2} \) for any nonnegative function \( f \). (f) Use part (e) to deduce that \( P_{S} \) is a linear map. (g) Use part (e) to conclude that \( V V^{T} \) is the canonical matrix associated to \( P_{S} \).