(Solved): Problem 3 (Control Chart Practice) Q3-1. A manufacturer of components for automobile transmissions ...

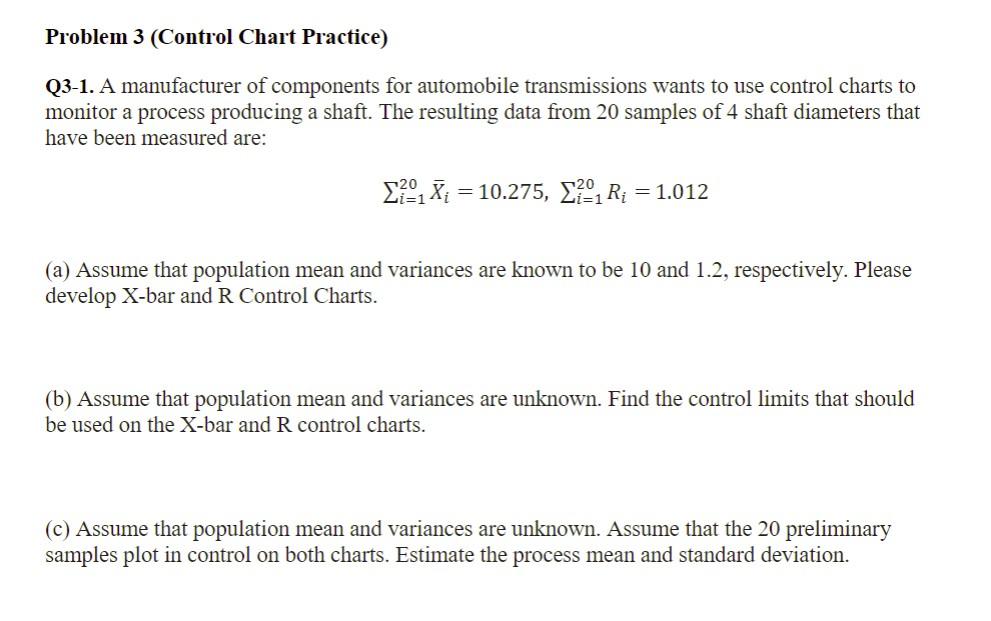
Problem 3 (Control Chart Practice) Q3-1. A manufacturer of components for automobile transmissions wants to use control charts to monitor a process producing a shaft. The resulting data from 20 samples of 4 shaft diameters that have been measured are: \[ \sum_{i=1}^{20} \bar{X}_{i}=10.275, \sum_{i=1}^{20} R_{i}=1.012 \] (a) Assume that population mean and variances are known to be 10 and 1.2, respectively. Please develop X-bar and \( \mathrm{R} \) Control Charts. (b) Assume that population mean and variances are unknown. Find the control limits that should be used on the X-bar and \( \mathrm{R} \) control charts. (c) Assume that population mean and variances are unknown. Assume that the 20 preliminary samples plot in control on both charts. Estimate the process mean and standard deviation. Q3-2. Samples of \( \mathrm{n}=4 \) items are taken from a process at regular intervals. A normally distributed quality characteristic is measured and \( \bar{X} \) and \( S \) values are calculated for each sample. After 50 groups have been analyzed, we have \[ \sum_{i=1}^{50} \bar{X}_{i}=1,000, \sum_{i=1}^{50} S_{i}=72 \] (a) Assume that population mean and standard deviation are known to be 21 and \( 1.5 \), respectively. Please develop X-bar and S Control Charts. (b) Assume that population mean and standard deviation are unknown. Find the control limits that should be used on the X-bar and S Control charts. (c)* Assume that population mean and standard deviation are unknown. Assuming also that if an item exceeds the upper specification limit it can be reworked, and if it is below the lower specification limit it must be scrapped, what percentage scrap and rework is the process now producing?