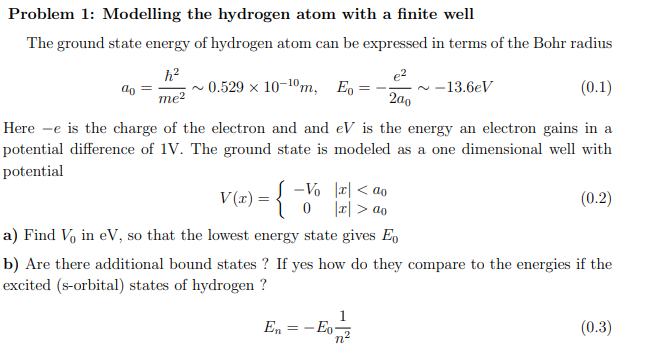Home /
Expert Answers /
Advanced Physics /
problem-1-modelling-the-hydrogen-atom-with-a-finite-well-the-ground-state-energy-of-hydrogen-atom-c-pa609
(Solved): Problem 1: Modelling the hydrogen atom with a finite well The ground state energy of hydrogen atom c ...
Problem 1: Modelling the hydrogen atom with a finite well
The ground state energy of hydrogen atom can be expressed in terms of the Bohr radius
a_(0)=(h^(2))/(me^(2))∼0.529\times 10^(-10)m,E_(0)=-(e^(2))/(2a_(0))∼-13.6eV
Here -e is the charge of the electron and and eV is the energy an electron gains in a
potential difference of 1 V . The ground state is modeled as a one dimensional well with
potential
V(x)={(-V_(0),|x|a_(0)):}
aV_(0) in eV , so that the lowest energy state gives E_(0)
bE_(n)=-E_(0)(1)/(n^(2))