(Solved): Problem 1: 1,3,5-Hexatriene \( (H) \) is known to convert to three different dimers, \( A, B \), a ...

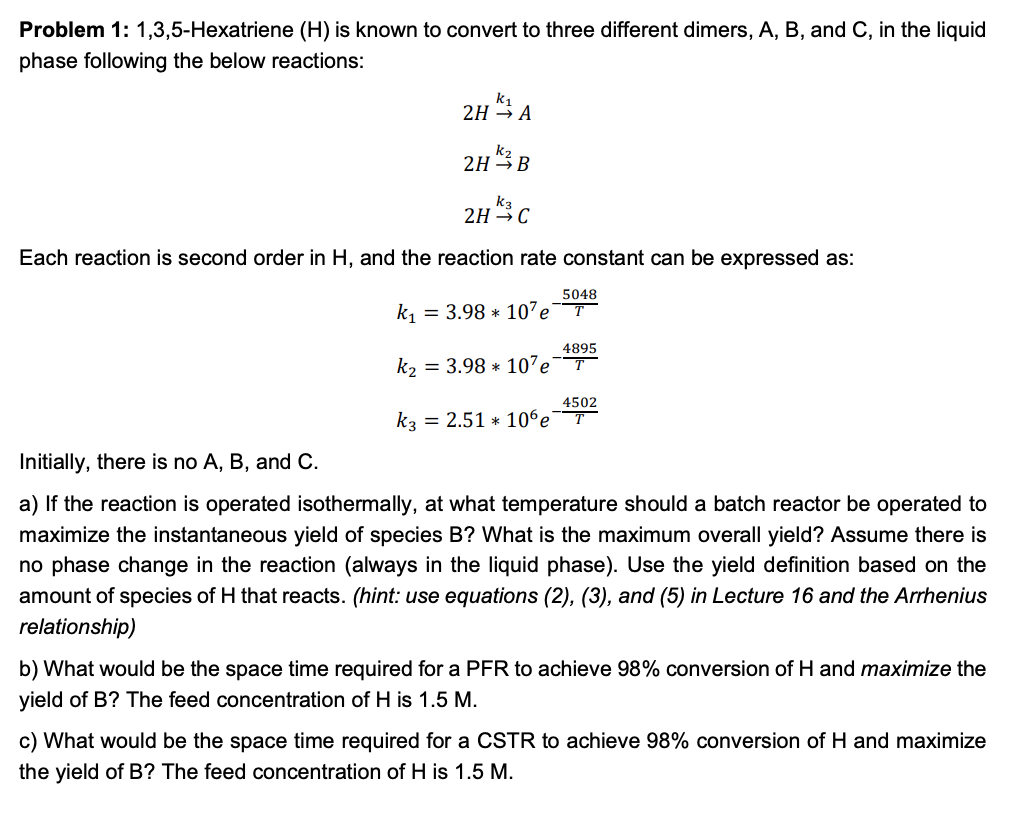
Problem 1: 1,3,5-Hexatriene \( (H) \) is known to convert to three different dimers, \( A, B \), and \( C \), in the liquid phase following the below reactions: \[ \begin{array}{l} 2 H \stackrel{k_{1}}{\rightarrow} A \\ 2 H \stackrel{k_{2}}{\rightarrow} B \\ 2 H \stackrel{k_{3}}{\rightarrow} C \end{array} \] Each reaction is second order in \( \mathrm{H} \), and the reaction rate constant can be expressed as: \[ \begin{array}{l} k_{1}=3.98 * 10^{7} e^{-\frac{5048}{T}} \\ k_{2}=3.98 * 10^{7} e^{-\frac{4895}{T}} \\ k_{3}=2.51 * 10^{6} e^{-\frac{4502}{T}} \end{array} \] Initially, there is no \( A, B \), and \( C \). a) If the reaction is operated isothermally, at what temperature should a batch reactor be operated to maximize the instantaneous yield of species B? What is the maximum overall yield? Assume there is no phase change in the reaction (always in the liquid phase). Use the yield definition based on the amount of species of \( \mathrm{H} \) that reacts. (hint: use equations (2), (3), and (5) in Lecture 16 and the Arrhenius relationship) b) What would be the space time required for a PFR to achieve \( 98 \% \) conversion of \( \mathrm{H} \) and maximize the yield of \( B \) ? The feed concentration of \( \mathrm{H} \) is \( 1.5 \mathrm{M} \). c) What would be the space time required for a CSTR to achieve \( 98 \% \) conversion of \( \mathrm{H} \) and maximize the yield of \( B \) ? The feed concentration of \( \mathrm{H} \) is \( 1.5 \mathrm{M} \).