(Solved): PLEASE SOLVE QUESTION B The Figure shows an existing \( 1.0 \mathrm{~km} \) long pipe that su ...

PLEASE SOLVE QUESTION B

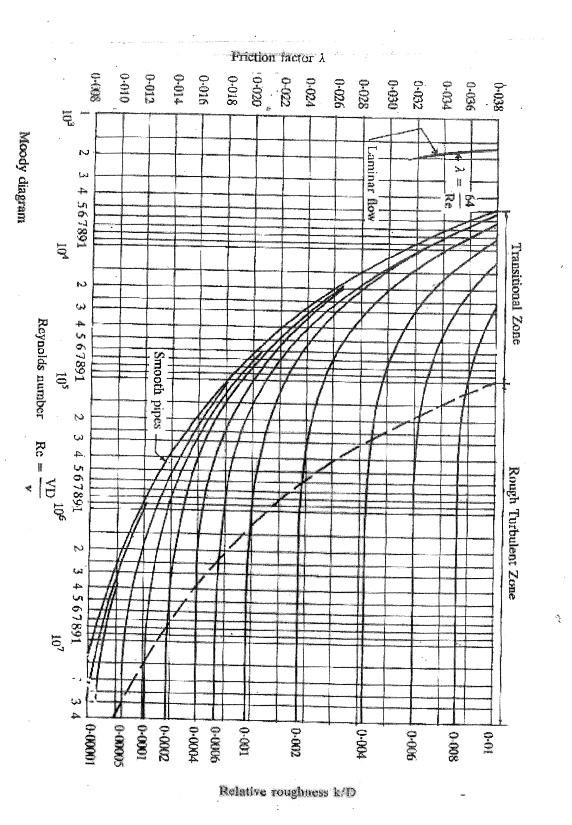
The Figure shows an existing \( 1.0 \mathrm{~km} \) long pipe that supplies water from a zone to a service reservoir. The pipe is \( 100 \mathrm{~mm} \) in diameter and the estimated effective wall roughness \( \mathrm{k}_{\mathrm{s}} \) is \( 0.60 \mathrm{~mm} \). a) Given a difference in water levels of \( 16.77 \mathrm{~m} \) between the two reservoirs, determine the flow rate in the pipe. Assume a kinematic viscosity \( v \) of \( 1.0 \times 10^{-6} \mathrm{~m}^{2} \mathrm{~s}^{-1} \) and ignore local losses. b) A new pipe is proposed to supplement the existing one for half the length \( (500 \mathrm{~m}) \) as shown in the Figure in order to allow a flow rate of 10 litres/per second from the zone to the service reservoir. Assuming the same water level difference of \( 16.77 \mathrm{~m} \mathrm{will} \) be retained, determine the required diameter of the new pipe. The effective wall roughness \( \mathrm{k}_{\mathrm{s}} \) of the new pipe is \( 0.40 \mathrm{~mm} \). The Moody chart is provided for your use and for any trial and error computation you need to do; assume a starting diameter of \( 100 \mathrm{~mm} \). The Moody chart is provided for your use and for any trial and error computation you need to do; assume a starting diameter of \( 100 \mathrm{~mm} \).