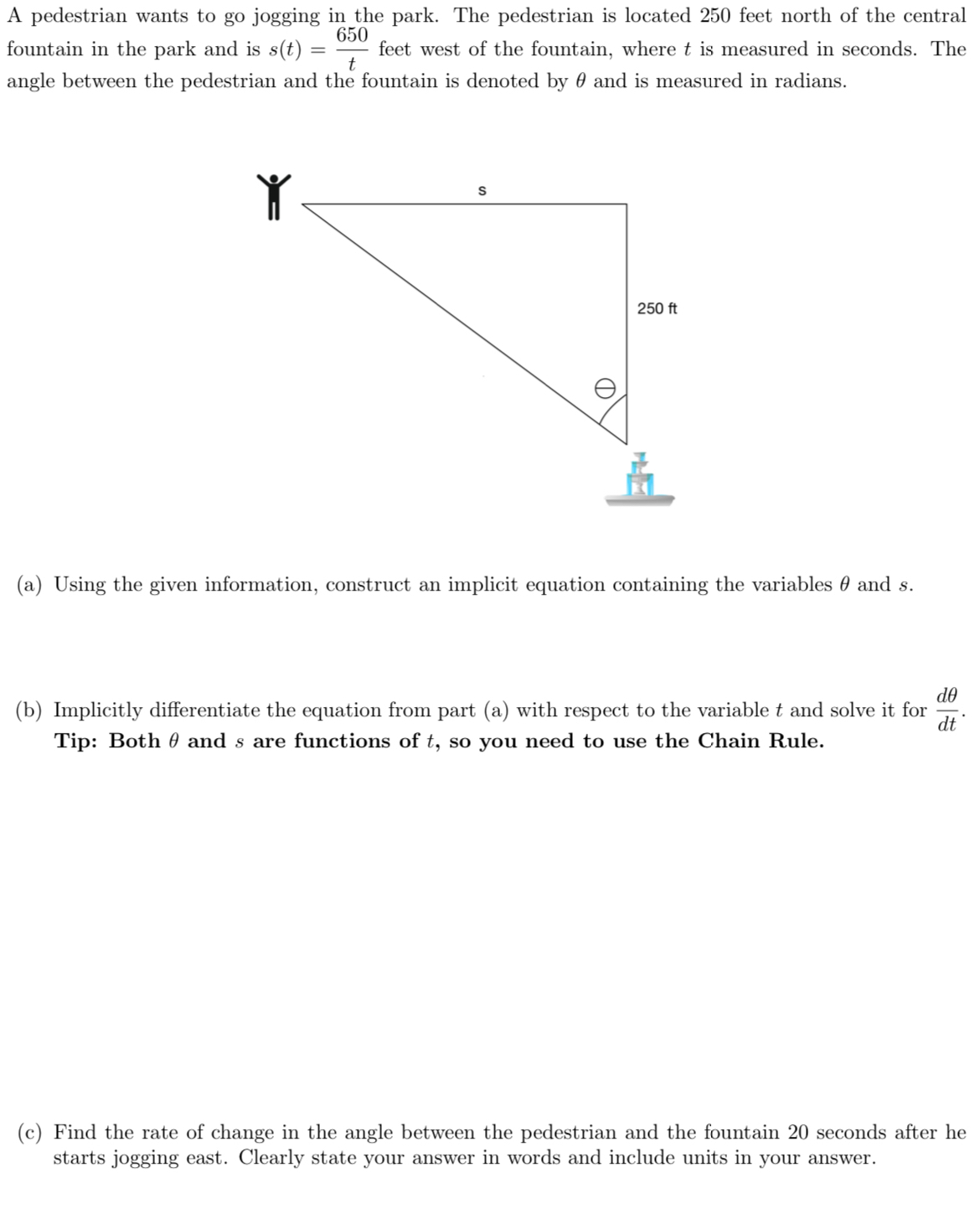
(Solved): Please answer every part! A pedestrian wants to go jogging in the park. The pedestrian is located 2 ...

Please answer every part! A pedestrian wants to go jogging in the park. The pedestrian is located 250 feet north of the central fountain in the park and is

feet west of the fountain, where

is measured in seconds. The angle between the pedestrian and the fountain is denoted by

and is measured in radians. (a) Using the given information, construct an implicit equation containing the variables

and

. (b) Implicitly differentiate the equation from part (a) with respect to the variable

and solve it for

. Tip: Both

and

are functions of

, so you need to use the Chain Rule. (c) Find the rate of change in the angle between the pedestrian and the fountain 20 seconds after he starts jogging east. Clearly state your answer in words and include units in your answer.