(Solved): PLEASE ANSWER ALL PARTS Axial loads are applied with rigid bearing plates to the solid cylindrical ...

PLEASE ANSWER ALL PARTS

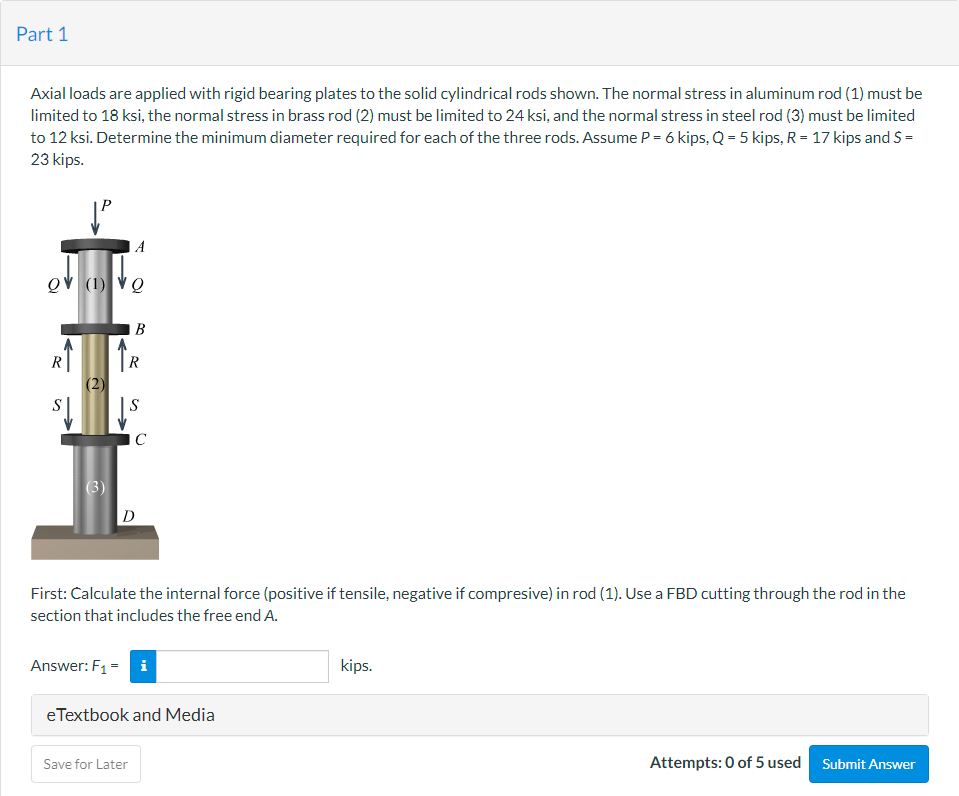
Axial loads are applied with rigid bearing plates to the solid cylindrical rods shown. The normal stress in aluminum rod (1) must be limited to \( 18 \mathrm{ksi} \), the normal stress in brass rod (2) must be limited to \( 24 \mathrm{ksi} \), and the normal stress in steel rod (3) must be limited to \( 12 \mathrm{ksi} \). Determine the minimum diameter required for each of the three rods. Assume \( P=6 \) kips, \( Q=5 \) kips, \( R=17 \mathrm{kips} \) and \( S= \) 23 kips. First: Calculate the internal force (positive if tensile, negative if compresive) in rod (1). Use a FBD cutting through the rod in the section that includes the free end \( A \). Answer: \( F_{1}= \) kips. eTextbook and Media Attempts: 0 of 5 used Calculate the internal force (positive if tensile, negative if compresive) in rod (2). Use a FBD cutting through the rod in the section that includes the free end \( A \). Answer: \( F_{2}= \) kips eTextbook and Media Attempts: 0 of 5 used Part 3 Calculate the internal force (positive if tensile, negative if compressive) in rod (3). Use a FBD cutting through the rod in the section that includes the free end \( A \). Answer: \( F_{3}= \) kips. eTextbook and Media Attempts: 0 of 5 used Part 4 Calculate the minimum required cross-sectional areas, and in turn, the minimum rod diameters using the rod internal force magnitudes and the allowable stresses for each rod material. Answers: The normal stress in aluminum rod (1) must be limited to \( 18 \mathrm{ksi} \); therefore, the minimum cross-sectional area required for rod (1) is in \( ^{2} \) and the minimum rod diameter is therefore in. The normal stress in brass rod (2) must be limited to \( 24 \mathrm{ksi} \); therefore, the minimum cross-sectional area required for rod (2) is in \( ^{2} \) and the minimum rod diameter is therefore in. The normal stress in steel rod (3) must be limited to \( 12 \mathrm{ksi} \); therefore, the minimum cross-sectional area required for rod (3) is in \( ^{2} \) and the minimum rod diameter is therefore in.