(Solved): part: c, d, e, f, and j please & show work!  (13\%) Problem 5: A ball is thrown upward from ...

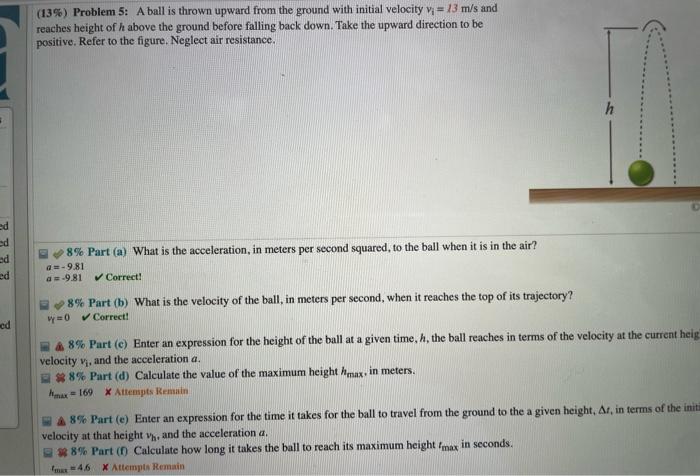
(13\%) Problem 5: A ball is thrown upward from the ground with initial velocity \( v_{i}=13 \mathrm{~m} / \mathrm{s} \) and reaches height of \( h \) above the ground before falling back down. Take the upward direction to be positive. Refer to the figure. Neglect air resistance. \( 8 \% \) Part (a) What is the acceleration, in meters per second squared, to the ball when it is in the air? \( a=-9.81 \) \( a=-9.81 \) Correct! \( 8 \% \) Part (b) What is the velocity of the ball, in meters per second, when it reaches the top of its trajectory? \( v_{\mathrm{r}}=0 \quad \vee \) Correct! A \( 8 \% \) Part (c) Enter an expression for the height of the ball at a given time, \( h \), the ball reaches in terms of the velocity at the current hei, velocity \( v_{\mathrm{i}} \), and the acceleration \( a \). \( \$ 8 \% \) Part (d) Calculate the value of the maximum height \( h_{\max } \), in meters. \( h_{\max }=169 \times \) Aitempts Kemain A \( 8 \% \) Part (e) Enter an expression for the time it takes for the ball to travel from the ground to the a given height, \( \Delta t \), in terms of the ini velocity at that height \( v_{\mathrm{h}} \), and the acceleration \( a \). \$1. \( 8 \% \) Part (f) Calculate how long it takes the ball to reach its maximum height \( t_{\max } \) in seconds. A \( 8 \% \) Part (c) Enter an expression for the height of the ball at a given time, \( h \), the ball reaches in terms of the velocity at the current heig velocity \( v_{1} \) and the acceleration \( a \). \( 138 \% \) Part (d) Calculate the value of the maximum height \( h_{\max } \), in meters. \( h_{\max }=169 \times \) Nttempts Resualis A \( 8 \% \) Part (e) Enter an expression for the time it takes for the ball to travel from the ground to the a given height, \( \Delta t \), in terms of the init velocity at that height \( v_{\mathrm{b}} \), and the acceleration \( a \). 4. \( 8 \% \) Part (f) Calculate how long it takes the ball to reach its maximum height \( t_{\max } \) in seconds. \( f_{\max }=4,6 \quad \mathrm{x} \) Aftempts Hemain A \( 8 \% \) Part \( (\mathrm{g}) \) What is the total displacement, in meters, of the ball going through its entire motion: traveling from the ground to the top back to the ground? \( 8 \% \) Part (h) Enter an expression for the total time of flight of the ball: the time from when it is launched to when it lands back on the this time in terms of \( y_{1} \) and \( a \). \( \Delta_{\text {ot }}=-2 \mathrm{v} / \mathrm{a} \quad \boldsymbol{u} \) Correct! \( 8 \% \) Part (i) Calculate the value of \( \Delta t_{\text {tot }} \) in seconds. \( \Delta f_{\text {oo }}=2.65 \quad \vee \) Correet! a \( 8 \% \) Part (j) Enter an expression for \( \Delta t_{\text {tox }} \) in terms of the variable \( t_{\max } \), the time of ascent from the ground to the top of the trajectory.