(Solved): PART (A) PART (B) The off-axis lamina shown in figure below is constrained without friction be ...

PART (A)

PART (B)

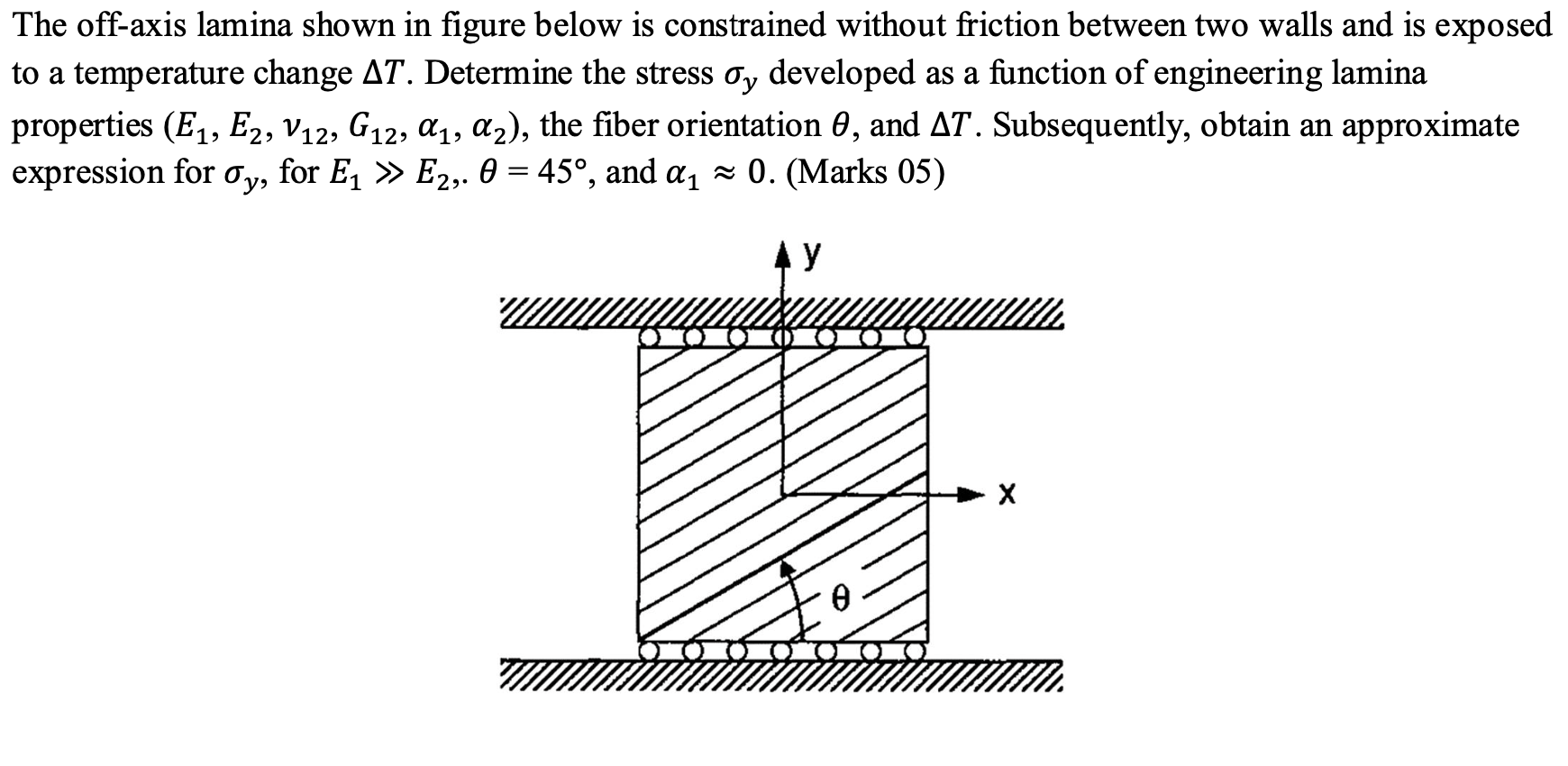
The off-axis lamina shown in figure below is constrained without friction between two walls and is exposed to a temperature change \( \Delta T \). Determine the stress \( \sigma_{y} \) developed as a function of engineering lamina properties \( \left(E_{1}, E_{2}, v_{12}, G_{12}, \alpha_{1}, \alpha_{2}\right) \), the fiber orientation \( \theta \), and \( \Delta T \). Subsequently, obtain an approximate expression for \( \sigma_{y} \), for \( E_{1} \gg E_{2}, . \theta=45^{\circ} \), and \( \alpha_{1} \approx 0 \). 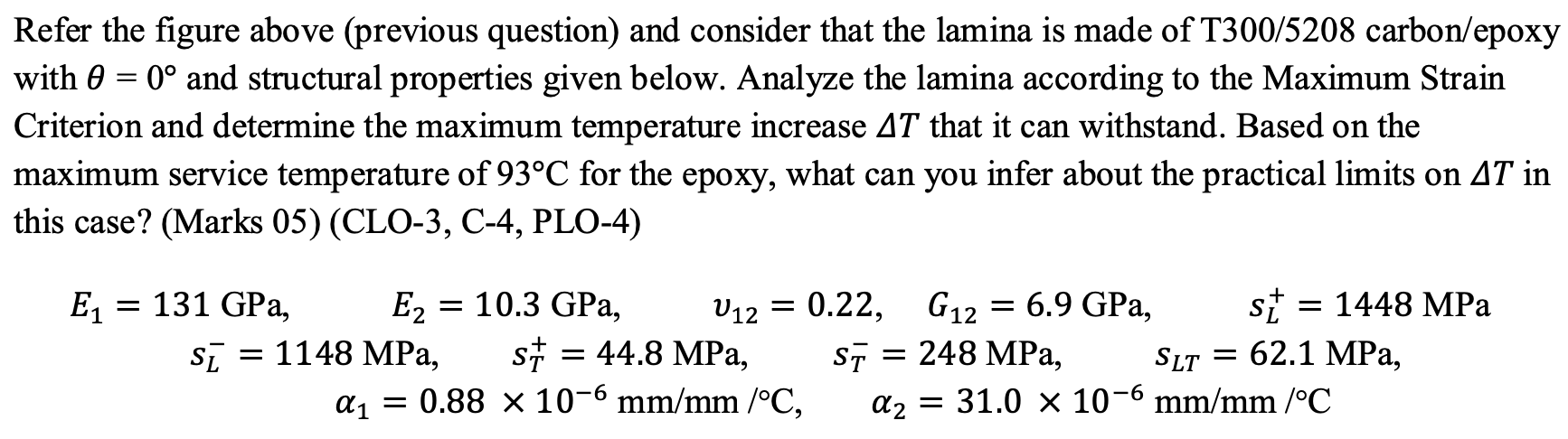(Marks 05) Refer the figure above (previous question) and consider that the lamina is made of \( \mathrm{T} 300 / 5208 \) carbon/epoxy with \( \theta=0^{\circ} \) and structural properties given below. Analyze the lamina according to the Maximum Strain Criterion and determine the maximum temperature increase \( \Delta T \) that it can withstand. Based on the maximum service temperature of \( 93^{\circ} \mathrm{C} \) for the epoxy, what can you infer about the practical limits on \( \Delta T \) in this case? (Marks 05) (CLO-3, C-4, PLO-4) \[ \begin{array}{c} E_{1}=131 \mathrm{GPa}, \quad E_{2}=10.3 \mathrm{GPa}, \quad v_{12}=0.22, \quad G_{12}=6.9 \mathrm{GPa}, \quad s_{L}^{+}=1448 \mathrm{MPa} \\ s_{L}^{-}=1148 \mathrm{MPa}, \quad s_{T}^{+}=44.8 \mathrm{MPa}, \quad s_{T}^{-}=248 \mathrm{MPa}, \quad s_{L T}=62.1 \mathrm{MPa}, \\ \alpha_{1}=0.88 \times 10^{-6} \mathrm{~mm} / \mathrm{mm} /{ }^{\circ} \mathrm{C}, \quad \alpha_{2}=31.0 \times 10^{-6} \mathrm{~mm} / \mathrm{mm} /{ }^{\circ} \mathrm{C} \end{array} \]