(Solved): PART 2: EARTHQUAKE EPICENTRE TRIANGULATION (30 MARKS) (Adapted from sciencecourseware.org.) In ord ...

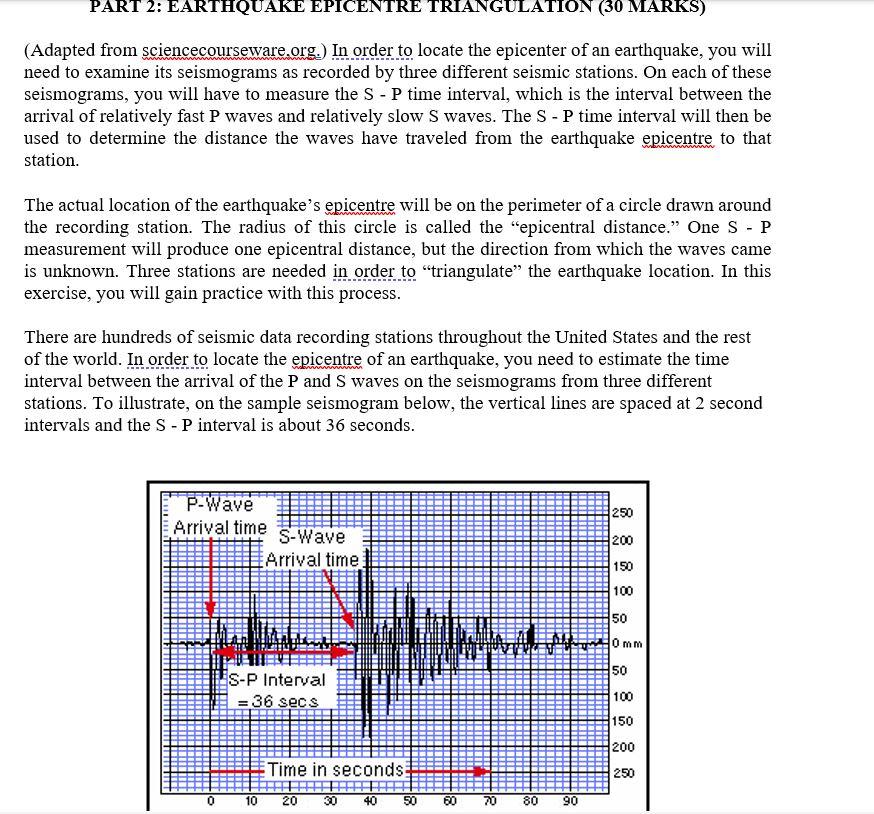
PART 2: EARTHQUAKE EPICENTRE TRIANGULATION (30 MARKS) (Adapted from sciencecourseware.org.) In order to locate the epicenter of an earthquake, you will need to examine its seismograms as recorded by three different seismic stations. On each of these seismograms, you will have to measure the S - P time interval, which is the interval between the arrival of relatively fast P waves and relatively slow S waves. The S - P time interval will then be used to determine the distance the waves have traveled from the earthquake epicentre to that station. The actual location of the earthquake's epicentre will be on the perimeter of a circle drawn around the recording station. The radius of this circle is called the "epicentral distance." One S - P measurement will produce one epicentral distance, but the direction from which the waves came is unknown. Three stations are needed in order to "triangulate" the earthquake location. In this exercise, you will gain practice with this process. There are hundreds of seismic data recording stations throughout the United States and the rest of the world. In order to locate the epicentre of an earthquake, you need to estimate the time interval between the arrival of the P and S waves on the seismograms from three different stations. To illustrate, on the sample seismogram below, the vertical lines are spaced at 2 second intervals and the S - P interval is about 36 seconds. 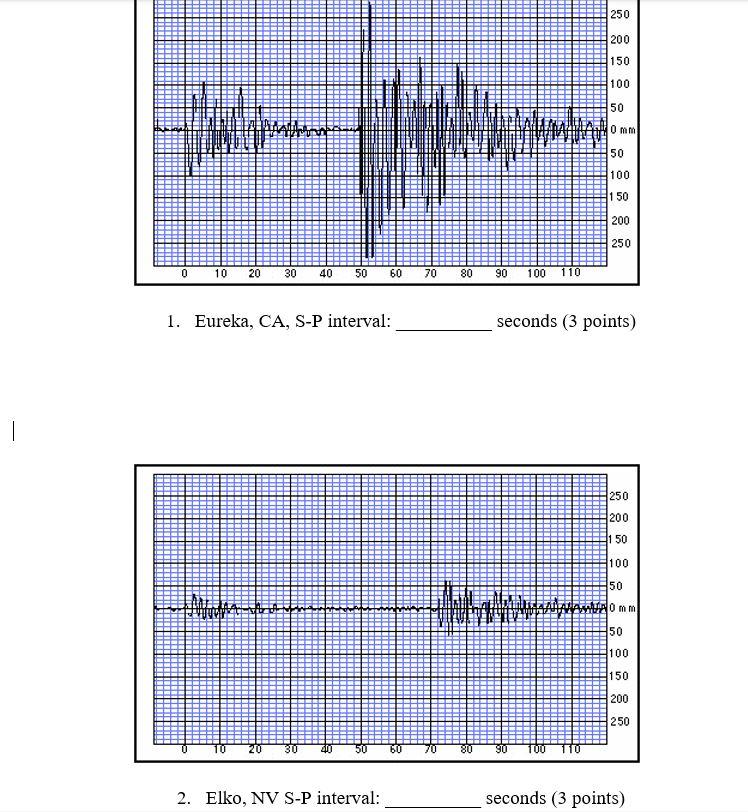P-Wave Arrival time 250 S-Wave Arrival time 0 S-P Interval = 36 secs 10 Time in seconds 20 30 40 50 60 70 80 90 8 8 8 8 200 150 100 50 0 mm 50 100 150 200 250 0 10 20 30 40 50 60 1. Eureka, CA, S-P interval: 20 2. 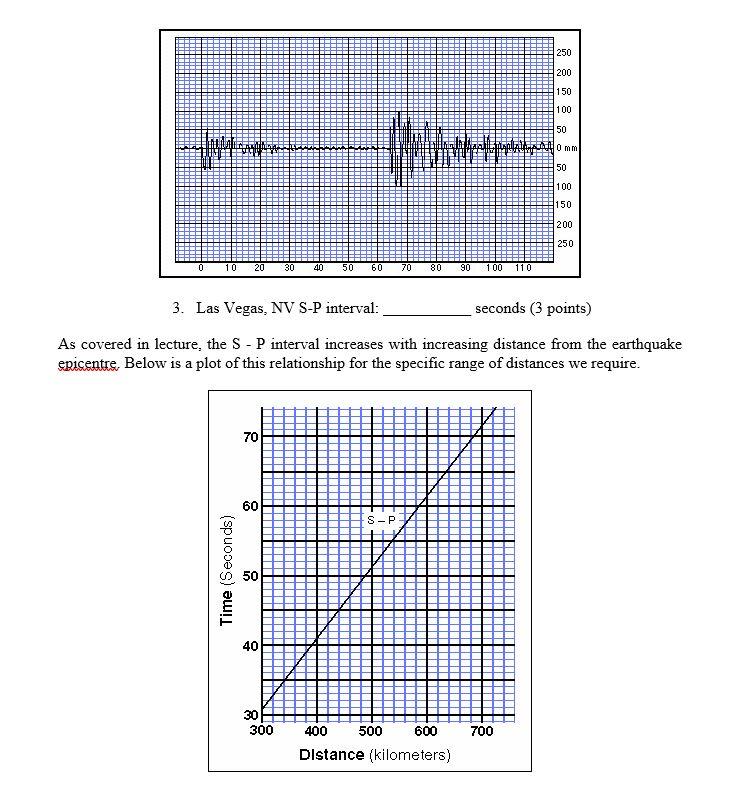Elko, NV S-P interval: 70 70 80 250 200 150 100 50 0 mm 50 100 1.50 200 250 90 100 110 seconds (3 points) 250 200 1 50 100 50 quavent om mm 50 100 150 200 250 seconds (3 points) 80 90 100 250 200 150 100 MA 50 0 mm - 50 100 150 200 250 0 10 20 30 40 50 60 70 80 90 100 110 3. Las Vegas, NV S-P interval: seconds (3 points) As covered in lecture, the S-P interval increases with increasing distance from the earthquake epicentre. Below is a plot of this relationship for the specific range of distances we require. 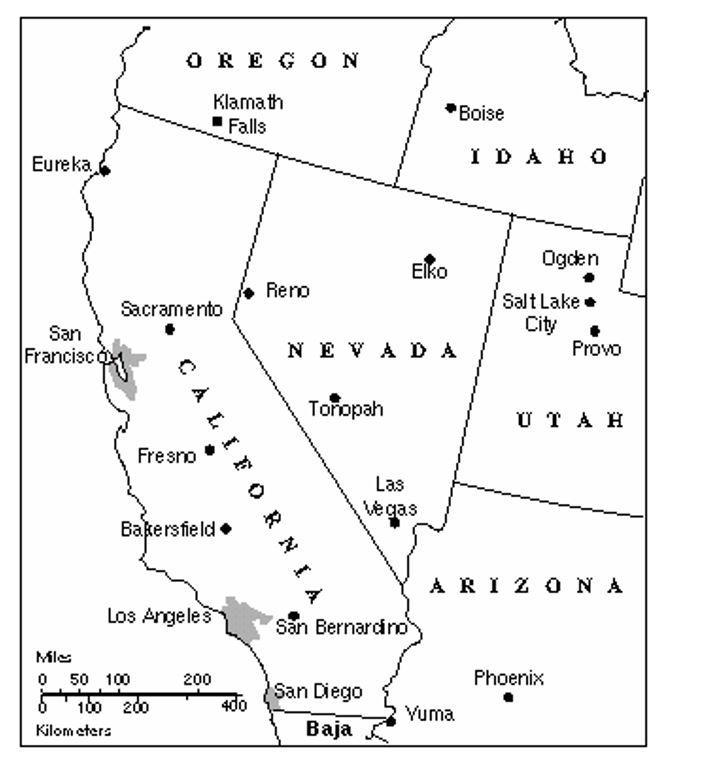70 60 S-P Time (Seconds) 50 40 30 300 400 500 600 Distance (kilometers) 700 Use the graph above and your previously-determined S - P intervals to obtain the epicentral distance from each seismograph station. Mark the corresponding points on the plot above. No additional explanation is required. 4. Epicentral distance from Eureka, CA: km (4 points) 5. Epicentral distance from Elko, NV: km (4 points) 6. Epicentral distance from Las Vegas, NV: km (4 points) 7. Using a compass or computer drawing tool, draw three circles on the map below. (No additional explanation is required.) Each circle should be centred at one of the seismograph stations used above. The radius of each circle should correspond to the epicentral distance measured from the seismograph station at the centre of the circle. Use the legend in the lower left of the map to convert geographic distance to distance on the page. (6 points) 8. Ideally, the three circles you drew on the map below should intersect at a point indicating the location of the epicentre. If your circles do not intersect at a point, it may be because of some inaccuracy in how you completed the previous steps. In the real world, inaccuracies in theoretical values of S P intervals often result in some uncertainty about the precise epicentre location, so don't dismay if your circles don't intersect precisely at a point! But you should at least see a location where the three circles are very close to intersecting at a single point, which will give you a good idea of the epicentre location. Mark on the map your best determination of the earthquake epicentre. No additional explanation is required. (3 points) Eureka San Francisco Sacramento OREGON Klamath Falls Milc 0 50 100 100 Kilometers Fresno Bakersfield Los Angeles 200 200 CALIFORNIA 400 Elko NEVADA Tonopah Reno *Boise Las Vegas San Bernardino San Diego Baja IDA HO Ogden Yuma Salt Lake City Provo UTAH ARIZONA Phoenix