(Solved): P1) The density of states \( (g(\varepsilon)) \) of an ideal hypothetical gas satisfies: \[ g(\vare ...

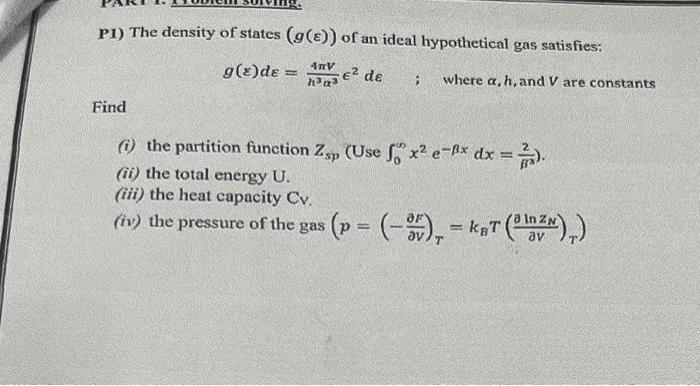
P1) The density of states \( (g(\varepsilon)) \) of an ideal hypothetical gas satisfies: \[ g(\varepsilon) d \varepsilon=\frac{4 \pi V}{h^{3} \alpha^{3}} \epsilon^{2} d \varepsilon \quad ; \quad \text { where } \alpha, h, \text { and } V \text { are constants } \] Find (i) the partition function \( Z_{s p} \) (Use \( \int_{0}^{\infty} x^{2} e^{-\beta x} d x=\frac{2}{\beta^{3}} \) ). (ii) the total energy \( U \). (iii) the heat capacity \( \mathrm{Cv} \). (iv) the pressure of the gas \( \left(p=\left(-\frac{\partial F}{\partial V}\right)_{T}=k_{B} T\left(\frac{\partial \ln z_{N}}{\partial V}\right)_{T}\right) \)