(Solved): Need Help For the function A whose graph is shown, state the following. (If the limit is infinite, e ...

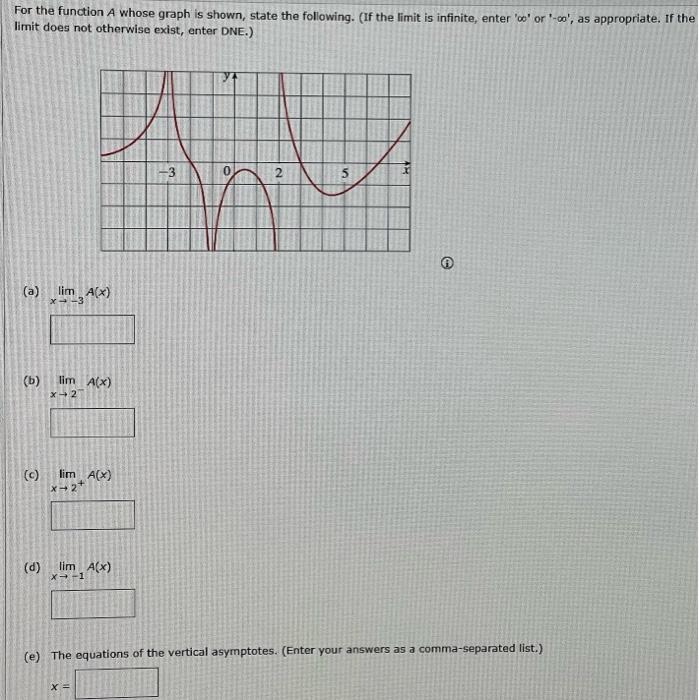
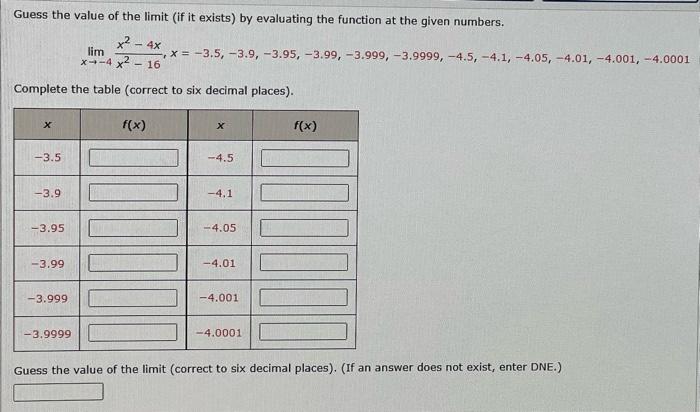
For the function A whose graph is shown, state the following. (If the limit is infinite, enter ' \( \infty \) ' or'- \( -\infty \) ', as appropriate. If the limit does not otherwise exist, enter DNE.) (a) \( \lim _{x \rightarrow-3} A(x) \) (b) \( \lim _{x \rightarrow 2^{-}} A(x) \) (c) \( \lim _{x \rightarrow 2^{+}} A(x) \) (d) \( \lim _{x \rightarrow-1} A(x) \) Guess the value of the limit (if it exists) by evaluating the function at the given numbers. \[ \lim _{x \rightarrow-4} \frac{x^{2}-4 x}{x^{2}-16}, x=-3.5,-3.9,-3.95,-3.99,-3.999,-3.9999,-4.5,-4.1,-4.05,-4.01,-4.001,-4.0001 \] Complete the table (correct to six decimal places). Guess the value of the limit (correct to six decimal places). (If an answer does not exist, enter DNE.)