(Solved):     4.2. Poisson probability distribution. The Poisson probability distribution is de ...

 

 

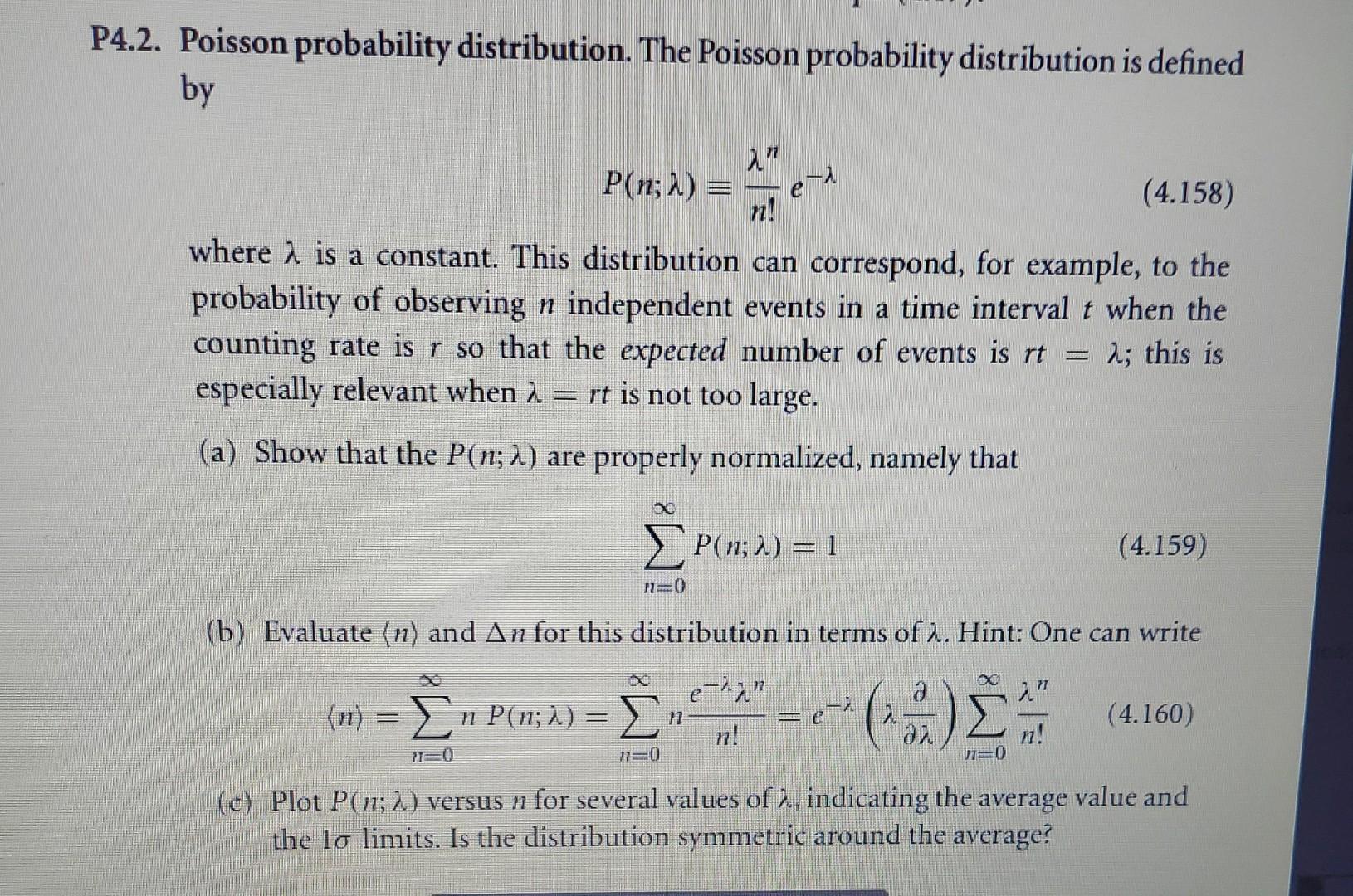
4.2. Poisson probability distribution. The Poisson probability distribution is defined by \[ P(n ; \lambda) \equiv \frac{\lambda^{n}}{n !} e^{-\lambda} \] where \( \lambda \) is a constant. This distribution can correspond, for example, to the probability of observing \( n \) independent events in a time interval \( t \) when the counting rate is \( r \) so that the expected number of events is \( r t=\lambda \); this is especially relevant when \( \lambda=r t \) is not too large. (a) Show that the \( P(n ; \lambda) \) are properly normalized, namely that \[ \sum_{n=0}^{\infty} P(n ; \lambda)=1 \] (b) Evaluate \( \langle n\rangle \) and \( \Delta n \) for this distribution in terms of \( \lambda \). Hint: One can write \[ \langle n\rangle=\sum_{n=0}^{\infty} n P(n ; \lambda)=\sum_{n=0}^{\infty} n \frac{e^{-\lambda} \cdot \lambda^{n}}{n !}=e^{-\lambda}\left(\lambda \frac{\partial}{\partial \lambda}\right) \sum_{n=0}^{\infty} \frac{\lambda^{n}}{n !} \] (c) Plot \( P(n ; \lambda) \) versus \( n \) for several values of \( \lambda \), indicating the average value and the \( 1 \sigma \) limits. Is the distribution symmetric around the average? (d) Assume that a book is 600 pages long and has a total of 1200 typographical errors. What is the average number of errors per page? What is the probability that a single page has no errors? How many pages would be expected to have less than 3 errors? What is the probability that a single page has 4 or more errors?