(Solved): Let Arg(z) be the principal argument function and let log(z) be the principal logarithm. 1. Use the ...

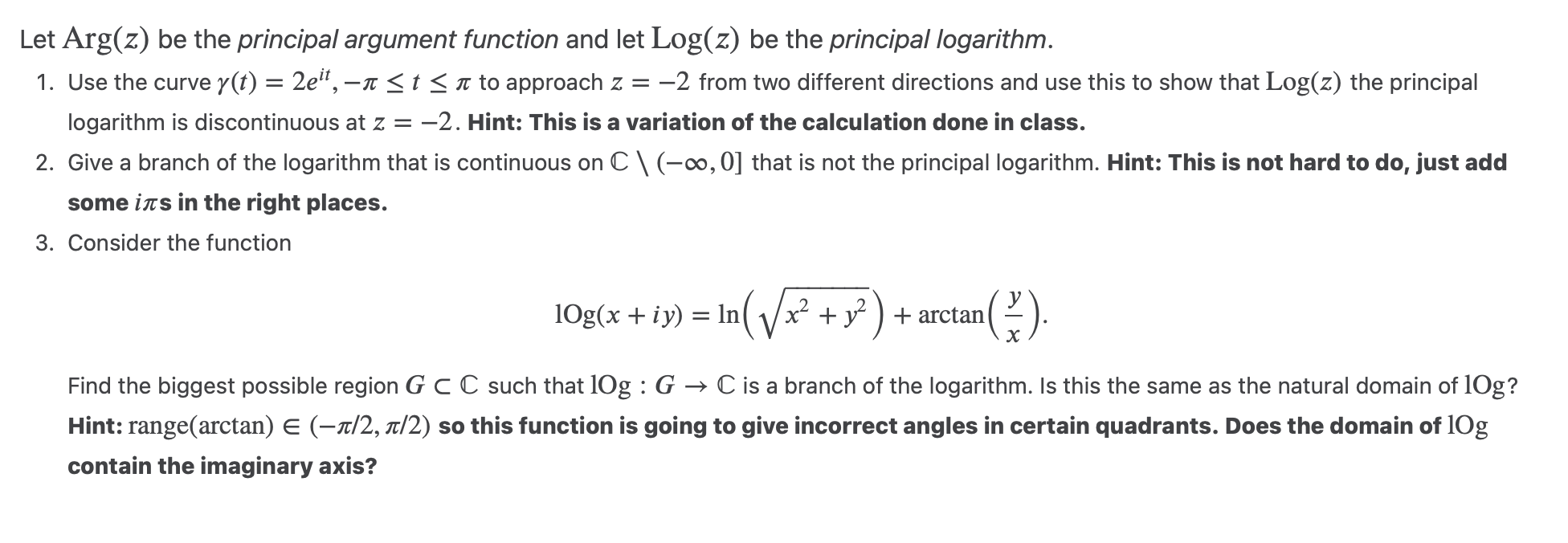
Let $\mathrm{Arg}(z)$ be the principal argument function and let $\log (z)$ be the principal logarithm. 1. Use the curve $\gamma (t)=2e^{it},-\pi \le t\le \pi$ to approach $z=-2$ from two different directions and use this to show that $\log (z)$ the principal logarithm is discontinuous at $z=-2$. Hint: This is a variation of the calculation done in class. 2. Give a branch of the logarithm that is continuous on $\mathbb{C}\backslash (-\infty ,0]$ that is not the principal logarithm. Hint: This is not hard to do, just add some $i\pi s$ in the right places. 3. Consider the function $\log (x+iy)=\ln \left(\sqrt{x^2+y^2}\right)+\arctan \left(\frac{y}{x}\right).$ Find the biggest possible region $G\subset \mathbb{C}$ such that $10g:G\to \mathbb{C}$ is a branch of the logarithm. Is this the same as the natural domain of $10g$ ? Hint: range $(\arctan )\in (-\pi /2,\pi /2)$ so this function is going to give incorrect angles in certain quadrants. Does the domain of $1\mathrm{Og}$ contain the imaginary axis?