(Solved): Let \( A \) and \( B \) be nonempty sets. We use the notation \( A^{B} \) to denote the set of all ...

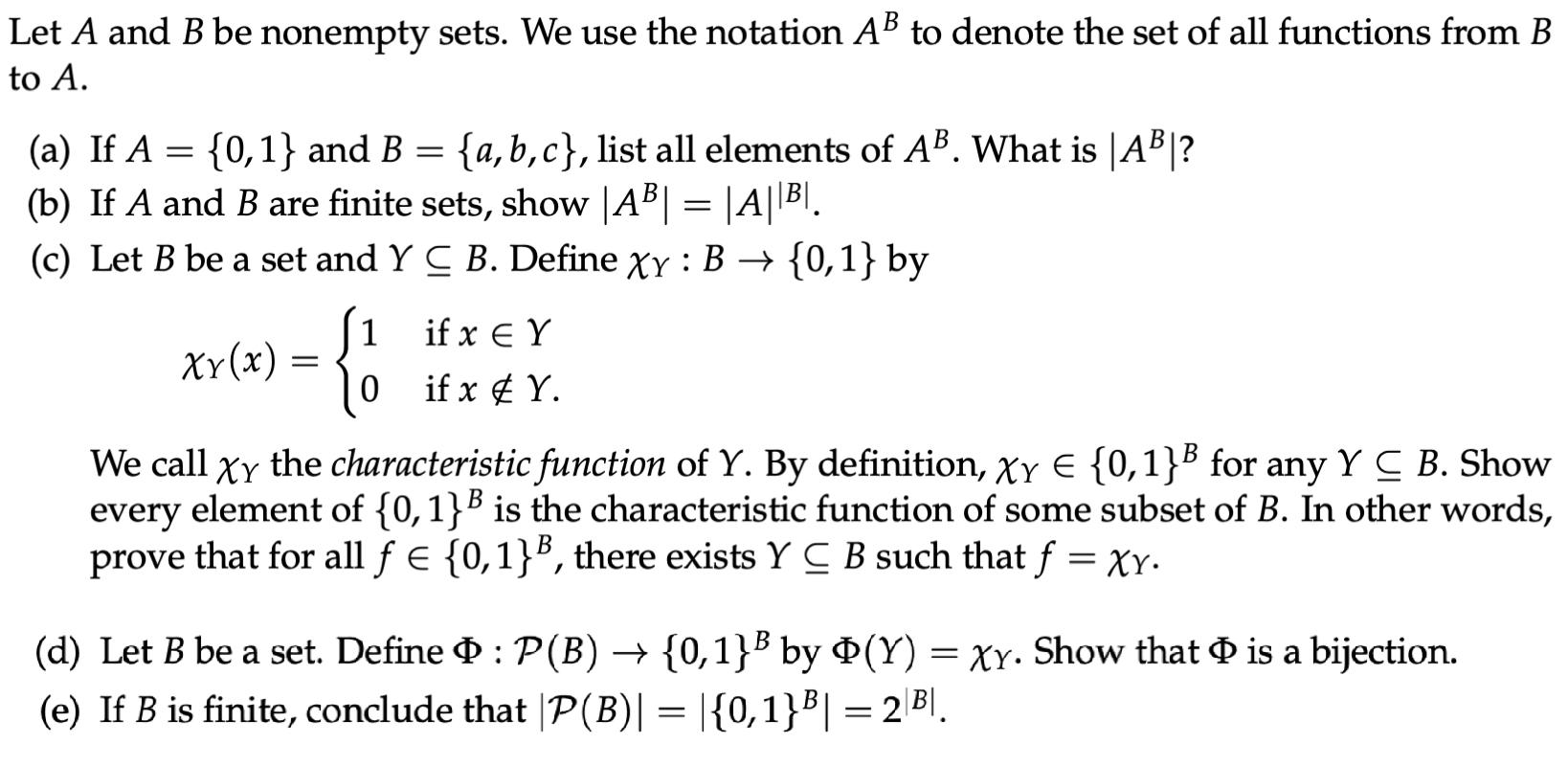
Let \( A \) and \( B \) be nonempty sets. We use the notation \( A^{B} \) to denote the set of all functions from \( B \) to \( A \) (a) If \( A=\{0,1\} \) and \( B=\{a, b, c\} \), list all elements of \( A^{B} \). What is \( \left|A^{B}\right| \) ? (b) If \( A \) and \( B \) are finite sets, show \( \left|A^{B}\right|=|A|^{|B|} \). (c) Let \( B \) be a set and \( Y \subseteq B \). Define \( \chi_{Y}: B \rightarrow\{0,1\} \) by \[ \chi_{Y}(x)=\left\{\begin{array}{ll} 1 & \text { if } x \in Y \\ 0 & \text { if } x \notin Y \end{array}\right. \] We call \( \chi_{Y} \) the characteristic function of \( Y \). By definition, \( \chi_{Y} \in\{0,1\}^{B} \) for any \( Y \subseteq B \). Show every element of \( \{0,1\}^{B} \) is the characteristic function of some subset of \( B \). In other words, prove that for all \( f \in\{0,1\}^{B} \), there exists \( Y \subseteq B \) such that \( f=\chi_{Y} \). (d) Let \( B \) be a set. Define \( \Phi: \mathcal{P}(B) \rightarrow\{0,1\}^{B} \) by \( \Phi(Y)=\chi_{Y} \). Show that \( \Phi \) is a bijection. (e) If \( B \) is finite, conclude that \( |\mathcal{P}(B)|=\left|\{0,1\}^{B}\right|=2^{|B|} \).