(Solved): Learning Goal: To show how a propagating triangle electromagnetic wave can satisfy Maxweli's equati ...

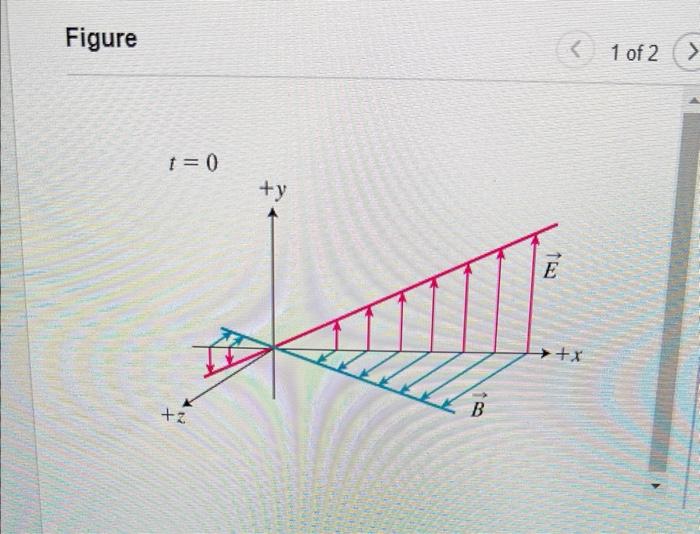
Learning Goal: To show how a propagating triangle electromagnetic wave can satisfy Maxweli's equations if the wave travels at speed \( c \). Light, radiant heat (infrared radiation). X rays, and radio waves are all examples of traveling electromagnetic waves. Electromagnetic waves consist of mutually compatible combinations of electric and magnetic fields ("mutually compatible" in the sense that changes in the electric field generate the magnetic field, and vice versa). The simplest form for a traveling electromagnetic wave is a plane wave. One particularly simple form for a plane wave is known as a "triangle wave, " in which the electric and magnetic fields are linear in position and time (rather than sinusoidal) In this problem we will investigate a triangle wave traveling in the \( x \) direction whose electric field is in the \( y \) direction. This wave is linearly polarized along the \( y \) axis; in other words, the electric field is always directed along the \( y \) axis. Its electric and magnetic fields are given by the following expressions: \[ E_{y}(x, t)=E_{0}(x-v t) / a \text { and } B_{2}(x, t)=B_{0}(x-v t) / a \text {. } \] where \( E_{0}, B_{0} \), and \( a \) are constants. The constant \( a \), which has dimensions of length, is introduced so that the constants \( E_{0} \) and \( B_{0} \) have dimensions of electric and magnetic field respectively. This wave is pictured in the figure at time \( t=0 \). (Figure.1) Note that we have only drawn the field vectors along the \( x \) axis. In fact, this idealized wave fills all space, but the field vectors only vary in the \( x \) direction. Wo arnart thic wava in eaticho Mavwafre areaatione. 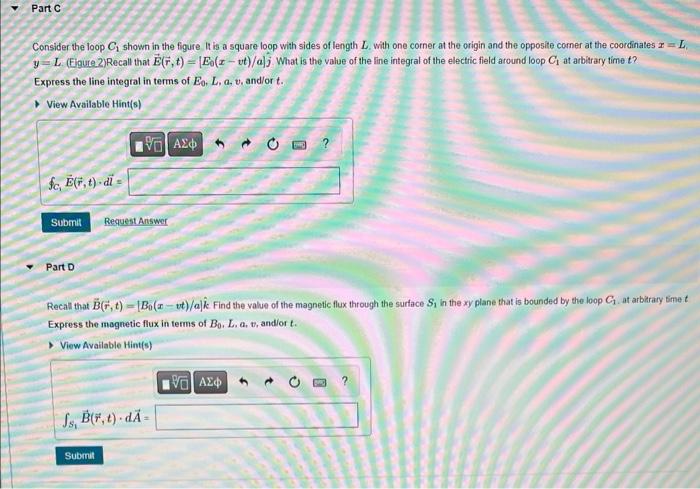For it in do en Consider the loop \( C_{1} \) shown in the figure \( I t \) is a square loop with sides of length \( L \), with one corner at the origin and the opposite corner at the coordinates \( x=L \). \( y=L \). (Eigure 2) Recall that \( \vec{E}(\vec{r}, t)=\left[E_{0}(x-v t) / a\right] j \). What is the value of the line integral of the electric field around loop \( C_{1} \) at arbitrary time \( t \) ? Express the line integral in terms of \( E_{0}, L, a, v \), and/or \( t \). Part D Recall that \( \ddot{B}(\vec{r}, t)=\left[B_{0}(x-v t) / a \mid \hat{k}\right. \). Find the value of the magnetic flux through the surface \( S_{1} \) in the xy plane that is bounded by the loop \( C_{1} \). at arbirary sme \( t \) Express the magnetic flux in terms of \( B_{0}, L, a, v \), andilor \( t \). Figure Use the Ampère-Maxwell law to find a new relationship between \( E_{0} \) and \( B_{0} \) Express \( E_{0} \) in terms of \( B_{0}, \mu 20, \varepsilon_{0} \), and other quantities given in the introduction: Part H Finally we are ready to show that the electric and magnetic fields given in the introduction describe an electromagnetic wave propagating at the speed of light if the electric and magnetic fields are to be self-consistent, they must obiey all of Maxwells equations Uaing one of Manwells equations, Faraday's law, we found a certain relationship between \( E_{0} \) and \( B_{0} \). You derived this in Part \( E \) Using another of Maxwells equations, the Ampere-Maxwell law, we found what appears fo be a diferent relationship between \( \mathrm{E}_{0} \) and \( B_{0} \).You derived this in Part I if the results of Parts \( \mathrm{E} \) and 1 are to apree. what does this imply that the speed of propagation \( e \) must be? Express \( v \) in terms of only 110 and \( 60 . \)