(Solved): Lab 4 Population Ecology Lab Adapted from biologyjunction.com and General Ecology Labs, Brown et. ...

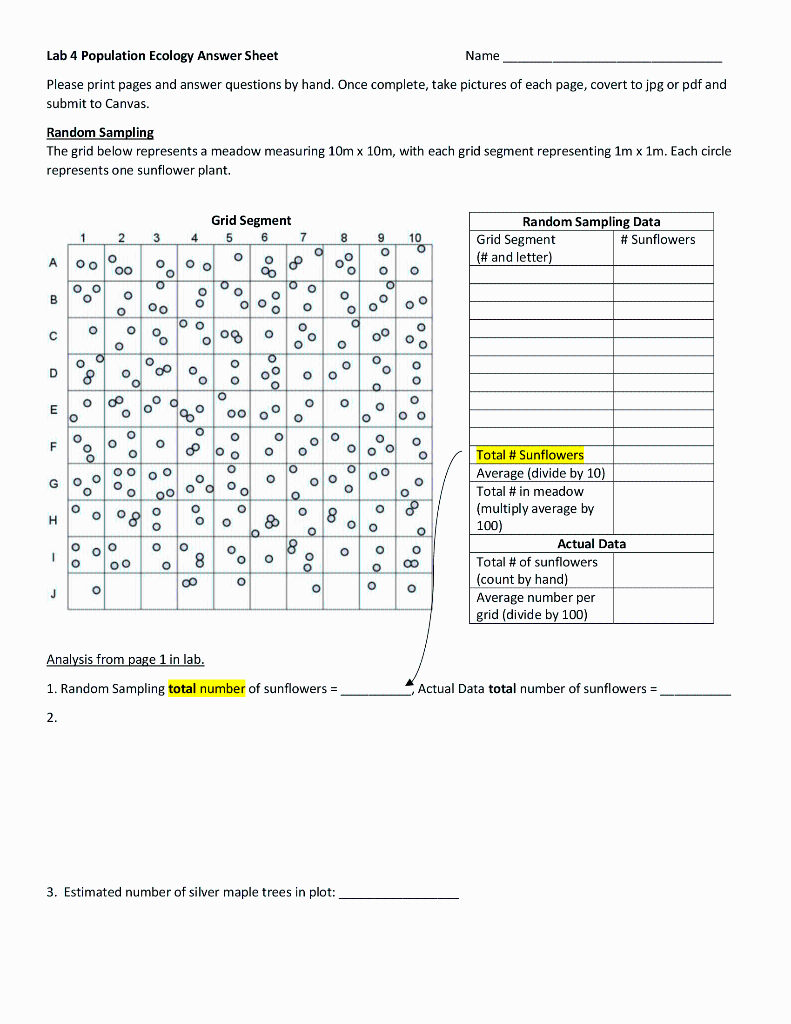
Lab 4 Population Ecology Lab Adapted from biologyjunction.com and General Ecology Labs, Brown et. al. *All answers will be recorded on Lab 4 Population Ecology Answer Sheet in Module Week \( 3 . \) Introduction Because it is difficult and time consuming to measure populations across entire areas, ecologists sometimes use random sampling techniques to calculate the estimated size of a population. Random sampling ensures each member of the population is equally likely to be included, a necessary component of the technique. Because areas of said population can be quite large and therfore unmanageable or impossible to cover and to ensure unbiased sampling, ecologists use quadrants and random number tables while sampling. Quadrants are small, equally sized plots placed randomly over a study site or area. Typically, preliminary studies are conducted to determine the optimal quadrant size, shape and number of quadrants. To ensure randomness of samples, we will mimic a random number table by using pairs of numbers as \( x \) and \( y \) coordinates while randomly pulling pieces of paper from containers. Random Sampling a. Cut a sheet of paper into 20 slips, each approximately \( 4 \mathrm{~cm} \times 4 \mathrm{~cm} \). b. Number 10 of the slips from 1 to 10 and put in a small container, bag, or envelope. c. Label the remaining slips A through \( \mathrm{J} \) and place in a second container, bag, or envelope. d. Randomly remove one slip from each container and write down the number letter combination on Random Sampling Data table on answer sheet. e. Find the Grid Segment (see answer sheet) that matches the combination and count the number of sunflower plants in that grid segment. Record this number in the data table on the answer sheet. f. Return each slip to its appropriate container. g. Repeat steps 4 - 6 until you have data for 10 different grid segments, completing the data table. h. Find the total number of sunflower plants for the 10 samples and record in data table. i. To find the average number of sunflowers per grid segment, divide the estimated total number of sunflowers by \( 10 . \) Record in data table. j. To calculate the estimated total sunflower population in the meadow based on your sample, multiply the average number (value of number 9 ) by 100 (total number of grid segments) and record. k. Now count all the sunflower plants on the grid by hand and record under Actual Data. Divide this value by 100 to calculate the average number of sunflower plants per grid. Analysis (answer questions \( 1-3 \) on page 1 of answer sheet) 1. Compare the total number of sunflowers from sampling data and actual count. 2. How close are random sampling values compared to actual number of sunflowers? 3. In a forest that measures 5 miles by 5 miles, random samples were taken to count the number of silver maple trees in the forest. The number of trees counted in sampled plots are listed in the grid shown below. (There is no need to fill in data in the blank cells.) Determine how many silver maple trees are in this forest using the random sampling technique. Please be sure to show your work. (Be mindful of the total number of plots.) Mark and Recapture Sometimes ecologists use the mark and recapture method when it is impossible to count every individual. The idea is to capture and leave some sort of marking on organisms before releasing them back into the original population. Different species are marked in a yariety of ways and include but are not limited to: collars placed on large mammals, notched fins on fish, photographs of dorsal fins on dolphins, paint markings on shells, and non-toxic dyes injected under skin of amphibians. After a period of time, the ecologist returns to the same sight for a second sampling where they will take note of how many captured are already marked. The assumption is that the proportion of marked individuals recaptured in the second sample represents the proportion of marked individuals in the population as a whole. This can be expressed mathematically using the Lincoln-Peterson Index equation below. (' Letters used below to represent variables are different than those presented in lecture, although they represent the same values.) \[ \mathbf{N}=\left\langle\mathrm{M}^{*} \mathrm{C}\right] / \mathbf{R} \] N = estimated \( \underline{\text { Number of population size }} \) \( M= \) number of individuals Marked and released c. - total number captured the seond time (with and without a mark) R- number of individuals Resaptured (those with a mark\} Practicing Mark and Recapture - Please answer all questions on page \( \mathbf{Z} \) of answer sheet. In a small population, you are more likely to recapture marked indiwiduals, whereas in a larec population, you are less likely. Understand that the smaller the number of recaptures \( \{\mathrm{R}\} \), the larger the estimate of population size. This makes geod biolagical sense because if the population is large, the marked animals you release into the wild will be mixing with a greater number of unmarked animals, so you will recapture a lower percentage of them in your second sample. Let us practice with sample problems. You hawe been tasked with determining how many Ferrell cats are liwing in Hart Park. On a Friday morning you pack up traps and collars and head to the park. With lots of patience, you end up capturing and placing collars on 33 cats before relcasing them back into the area from which you captured them. You return scuen days later to repcat the process and end up capturing 41 cats. Of these, 19 are wearing your collars. Now that you have collected the necessary data, you are ready to use the Lincoln-Peterson Index equation to estimate the size of the Ferrell cat population. 4. Fill in data on answer sheet for following wariables \( M, C \), and \( R . \) 5. Showing your work, calculate the estimated population size of Ferrell cats in Hart Park. Because you hawe had nothing, else to do during shelter in place, you've decided to take up pardening. You spend your hard-earned money and buy plants at your local nursery and plant them in your backyard. After a few days, you go outside in the morning to admire your work and sec that many of your plants are being caten by snails. You do some research and learn that the snails come out after the sprinklers have gone on and spend the rest of the day hiding in the vegetation to stay cool. There is no way of counting all the snails, but you need to know how much snail bait to buv. You decide to use the Lincoln-Peterson Index calculation to estimate the number of snails in the vard. 6. Give a detailed description of how you might mark and recapture snails in your yard. (You may use an illustration if you'd like.) 7. Following mark and recapture, you have the following data: \( \mathrm{M}=36, \mathrm{C}=29 \), and \( \mathrm{R}=11 \), About how many 5 ails are liwing in vour vard? 2 Lab 4 Population Ecology Answer Sheet Name Please print pages and answer questions by hand. Once complete, take pictures of each page, covert to .jpg or pdf and submit to Canvas. Random Sampling The grid below represents a meadow measuring \( 10 \mathrm{~m} \times 10 \mathrm{~m} \), with each grid segment representing \( 1 \mathrm{~m} \times 1 \mathrm{~m} \). Each circle represents one sunflower plant. Analysis from page 1 in lab. 1. Random Sampling total number of sunflowers = Actual Data total number of sunflowers \( = \) \( 2 . \) 3. Estimated number of silver maple trees in plot: Mark and Recapture 4. \( \mathrm{M}= \) \( \mathrm{C}= \) \( \mathbf{R} \) 5. Estimated population size of Ferrell cats in Hart Park: (please show your work!). \( 6 . \) 7. Estimated population size of snails in your yard: \{please show your work\}.