(Solved): Help please? Find the length of one arch of the cycloid x=r(sin()),y=r(1cos()) Solution ...

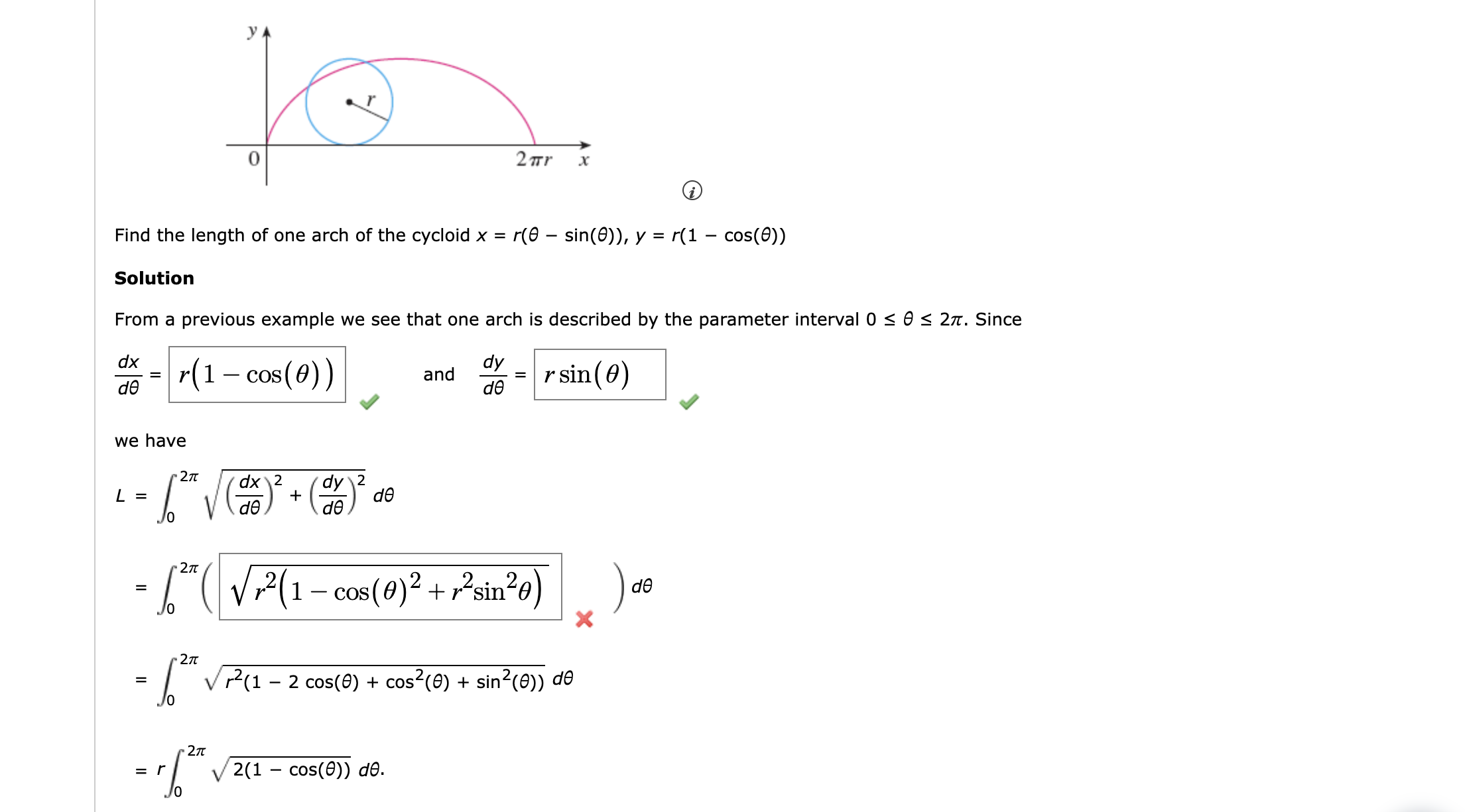
Find the length of one arch of the cycloid $x=r(\theta -\sin (\theta )),y=r(1-\cos (\theta ))$ Solution From a previous example we see that one arch is described by the parameter interval $0\le \theta \le 2\pi$. Since $\frac{dx}{d\theta }=r(1-\cos (\theta ))\text{ and }\frac{dy}{d\theta }=$ we have $\begin{array}{rl}L& ={\int }_0^{2\pi }\sqrt{{\left(\frac{dx}{d\theta }\right)}^2+{\left(\frac{dy}{d\theta }\right)}^2}d\theta \\ & ={\int }_0^{2\pi }\left(\sqrt{r^2\left(1-\cos (\theta {)}^2+r^2{\sin }^2\theta \right)}\right)d\theta \\ & ={\int }_0^{2\pi }\sqrt{r^2\left(1-2\cos (\theta )+{\cos }^2(\theta )+{\sin }^2(\theta )\right)}d\theta \\ & =r{\int }_0^{2\pi }\sqrt{2(1-\cos (\theta ))}d\theta .\end{array}$ $=r{\int }_0^{2\pi }\sqrt{2(1-\cos (\theta ))}d\theta$ 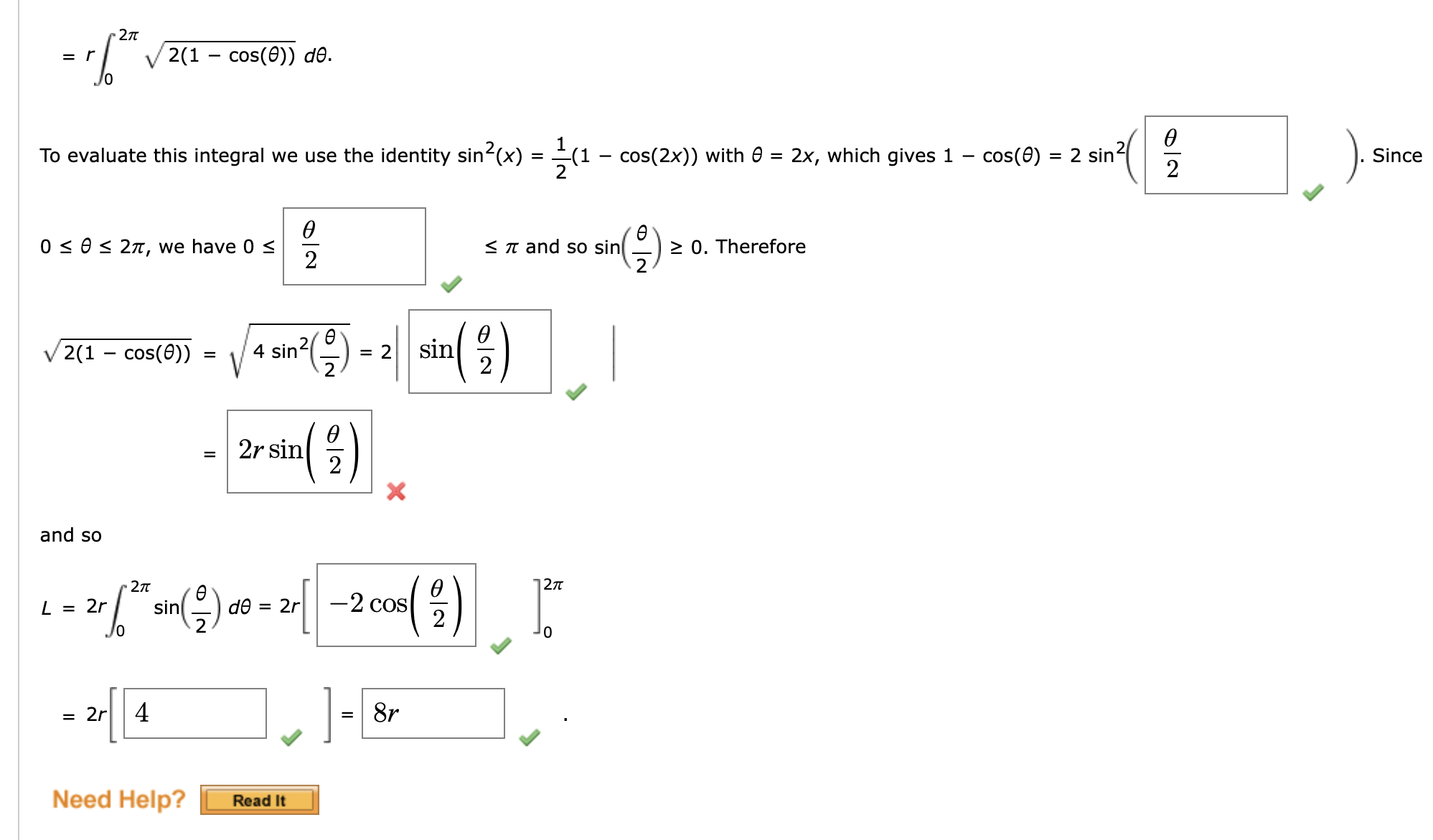To evaluate this integral we use the identity ${\sin }^2(x)=\frac{1}{2}(1-\cos (2x))$ with $\theta =2x$, which gives $1-\cos (\theta )=2\sin 2($ $0\le \theta \le 2\pi$, we have $0\le$ $\begin{array}{rl}\sqrt{2(1-\cos (\theta ))}& =\sqrt{4{\sin }^2\left(\frac{\theta }{2}\right)}=2\\ & =2r\sin \left(\frac{\theta }{2}\right)\end{array}$ and so $\begin{array}{rl}L& =2r{\int }_0^{2\pi }\sin \left(\frac{\theta }{2}\right)d\theta =2r[\\ & =2r[\end{array}$ $\le \pi$ and so $\sin \left(\frac{\theta }{2}\right)\ge 0$. Therefore ). Since