(Solved): First, lets consider a laminar flow of water in the system of pipes defined in the figure. There is ...
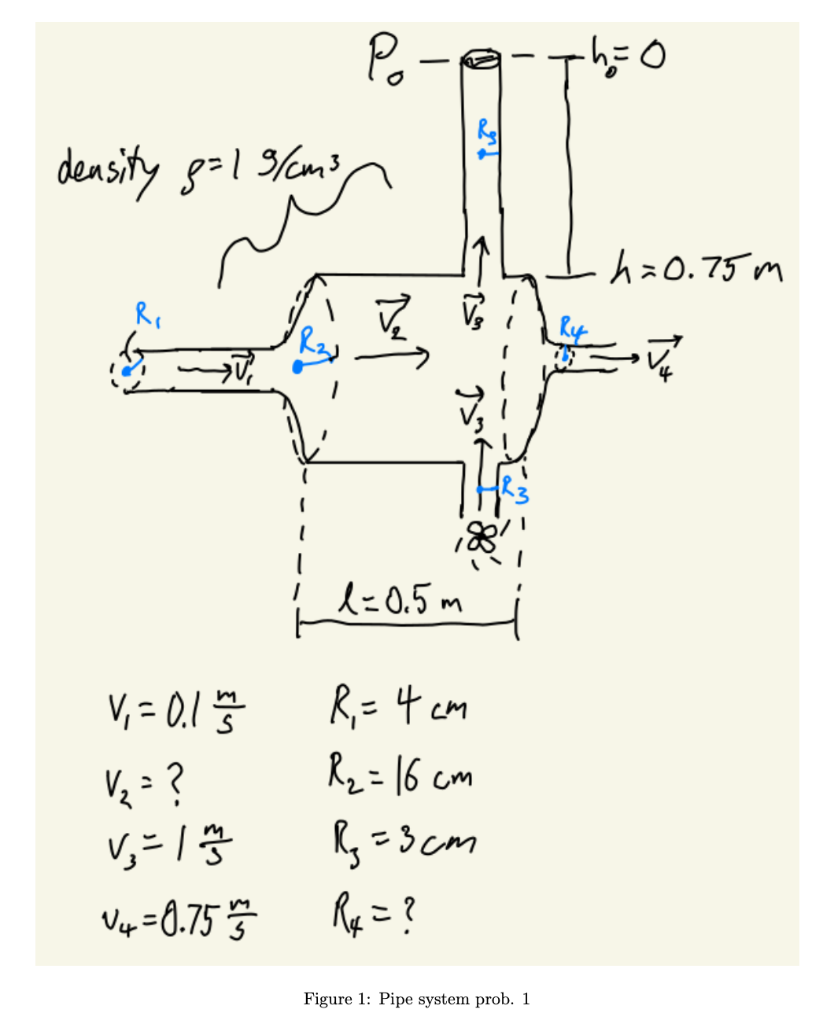
First, lets consider a laminar flow of water in the system of pipes defined in the figure. There is some mechanism pumping water (at constant power) towards the right through the pipe of radius R1, into the larger chamber which has radius R2, and out of pipe with radius R4. Additionally, there is a fan pumping a column of water (at constant power) in the pipe with radius R3 through the larger chamber and back out. Assume that the flow of water through both directions is conserved separately for both directions (can apply Bernoulli’s law for each direction). In the figure, we are given values for velocities v1, v3, & v4 and radii R1, R2, & R3.
a) Find v2 and R4 using the given values and the continuity of the fluid flow.
b) Find the pressure as a function of depth in the vertical column of water assuming that the vertical and
horizontal flows are perfectly perpendicular (i.e. pass through one another) and that the ambient pressure
is 1 atm.
Hint: when calculating the contribution to the pressure, we need to treat the horizontal flow in a piecewise
fashion that only contributes where the horizontal flow intersects the vertical flow inside the chamber. It may
be useful to first define the functions vvertical(h) and vhorizontal(h).
c) Now introduce some viscosity to our system defined by ?w = 10?3 Pa ·s and use the flow rate Q =
10?4 m3/s to determine the pressure difference between each side of the chamber of length l = 0.75 m (assume
the fan is turned off/vertical pipes are sealed such that there is no vertical flow for this part).
Expert Answer
Answer:Fluids are the name given to a substance that beigns to flow when force is appiled to it. Liquids and gasses are fluids. Fluids do not have the