(Solved): Find the steady-state vector for the transition matrix. 01010110751100 ...

Expert Answer

---

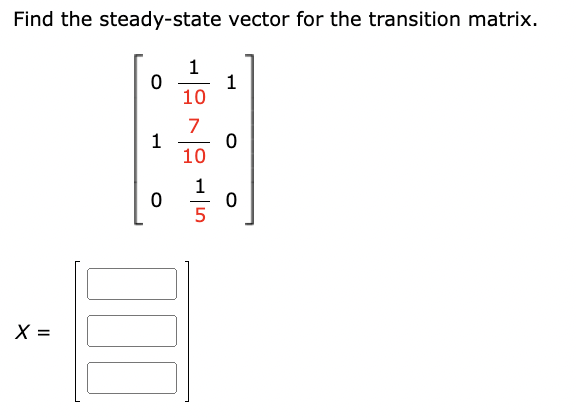
Given transition Matrix
Let p = transition Matrix
V = vector

Let X=V=   

Where X+Y+Z = 1


In the context of Markov chains, a steady-state vector (also known as a stationary vector or invariant distribution) of a transition matrix is a probability vector that remains unchanged after multiple iterations of the matrix. A transition matrix is a square matrix that describes the probabilities of transitioning between a set of states. Each row of the matrix corresponds to a starting state, while each column corresponds to an ending state. The entries in the matrix represent the probabilities of moving from the starting state to the ending state.