(Solved): Find the damping ratio and natural frequency for each second-order system and show that the value ...

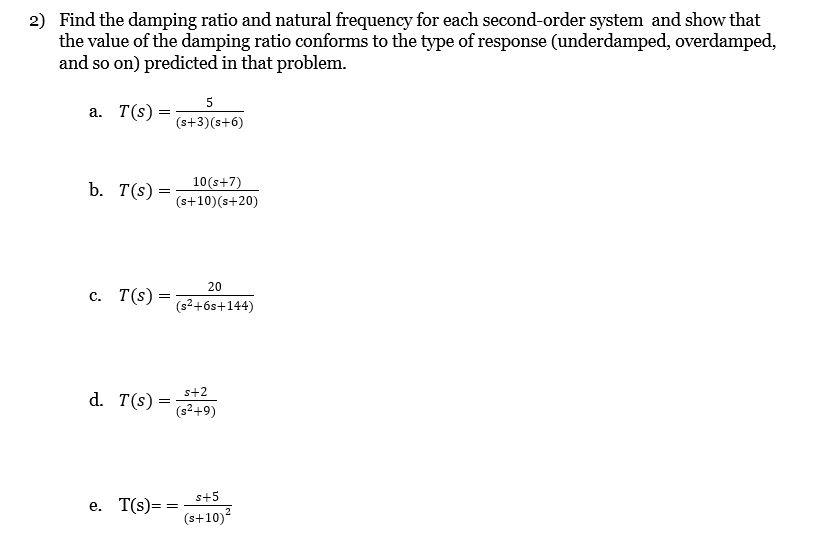
Find the damping ratio and natural frequency for each second-order system and show that the value of the damping ratio conforms to the type of response (underdamped, overdamped, and so on) predicted in that problem. a. \( \quad T(s)=\frac{5}{(s+3)(s+6)} \) b. \( T(s)=\frac{10(s+7)}{(s+10)(s+20)} \) c. \( T(s)=\frac{20}{\left(s^{2}+6 s+144\right)} \) d. \( T(s)=\frac{s+2}{\left(s^{2}+9\right)} \) e. \( \mathrm{T}(\mathrm{s})==\frac{s+5}{(s+10)^{2}} \)