(Solved): During our introduction of the Navier-Stokes equation, the velocity profile for Poiseuille flow, or ...

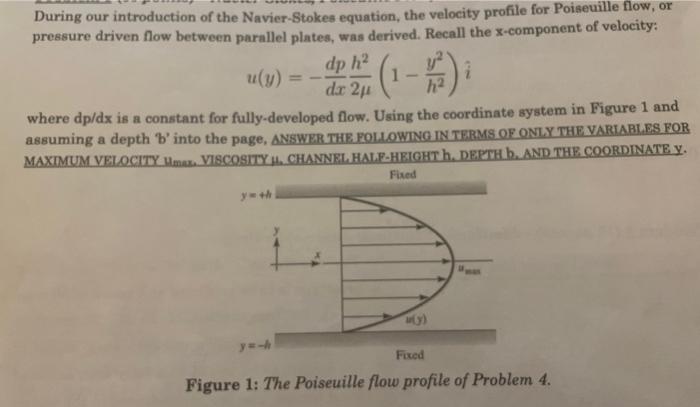
During our introduction of the Navier-Stokes equation, the velocity profile for Poiseuille flow, or pressure driven flow between parallel plates, was derived. Recall the x-component of velocity: \[ u(y)=-\frac{d p}{d x} \frac{h^{2}}{2 \mu}\left(1-\frac{y^{2}}{h^{2}}\right) \hat{i} \] where \( \mathrm{dp} / \mathrm{dx} \) is a constant for fully-developed flow. Using the coordinate system in Figure 1 and assuming a depth ' \( b \) ' into the page, ANSWRR THE FOL LWINO IN TFRMS OF ONLY THE VARIABLES EOR Figure 1: The Poiseuille flow profile of Problem 4. 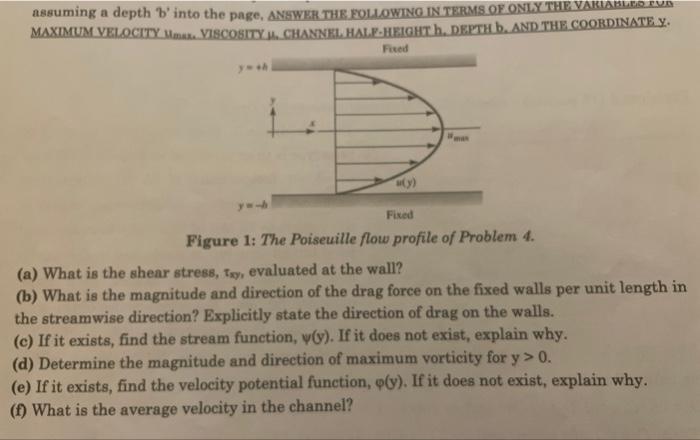Figure 1: The Poiseuille flow profile of Problem 4. (a) What is the shear stress, \( \tau_{x} \), evaluated at the wall? (b) What is the magnitude and direction of the drag force on the fixed walls per unit length in the streamwise direction? Explicitly state the direction of drag on the walls. (c) If it exists, find the stream function, \( v(y) \). If it does not exist, explain why. (d) Determine the magnitude and direction of maximum vorticity for \( y>0 \). (e) If it exists, find the velocity potential function, \( \varphi(y) \). If it does not exist, explain why. (f) What is the average velocity in the channel?