(Solved): Derive shear stress equation of body fluid in two parallel plates given in the below figure. (Assump ...

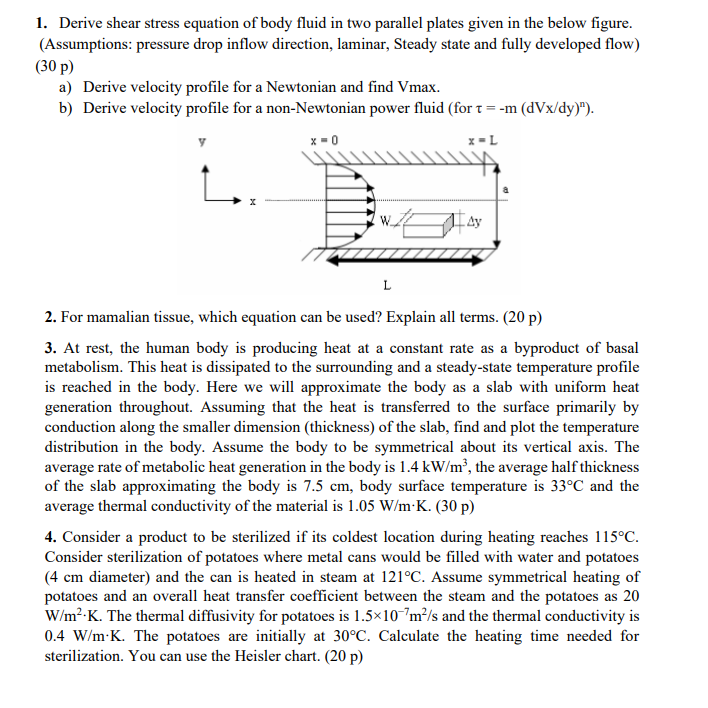
Derive shear stress equation of body fluid in two parallel plates given in the below figure. (Assumptions: pressure drop inflow direction, laminar, Steady state and fully developed flow)

a) Derive velocity profile for a Newtonian and find Vmax.

b) Derive velocity profile for a non-Newtonian power fluid (for τ = -m (dVx/dy)n ).

2. For mamalian tissue, which equation can be used? Explain all terms.

3. At rest, the human body is producing heat at a constant rate as a byproduct of basal metabolism. This heat is dissipated to the surrounding and a steady-state temperature profile is reached in the body. Here we will approximate the body as a slab with uniform heat generation throughout. Assuming that the heat is transferred to the surface primarily by conduction along the smaller dimension (thickness) of the slab, find and plot the temperature distribution in the body. Assume the body to be symmetrical about its vertical axis. The average rate of metabolic heat generation in the body is 1.4 kW/m3 , the average half thickness of the slab approximating the body is 7.5 cm, body surface temperature is 33°C and the average thermal conductivity of the material is 1.05 W/m·K.

4. Consider a product to be sterilized if its coldest location during heating reaches 115°C. Consider sterilization of potatoes where metal cans would be filled with water and potatoes (4 cm diameter) and the can is heated in steam at 121°C. Assume symmetrical heating of potatoes and an overall heat transfer coefficient between the steam and the potatoes as 20 W/m2 ·K. The thermal diffusivity for potatoes is 1.5×10−7m2 /s and the thermal conductivity is 0.4 W/m·K. The potatoes are initially at 30°C. Calculate the heating time needed for sterilization. You can use the Heisler chart.