(Solved): Consider the flash tank shown in the figure. Here, z(t),y(t) and x(t) are the mole fractions of the ...

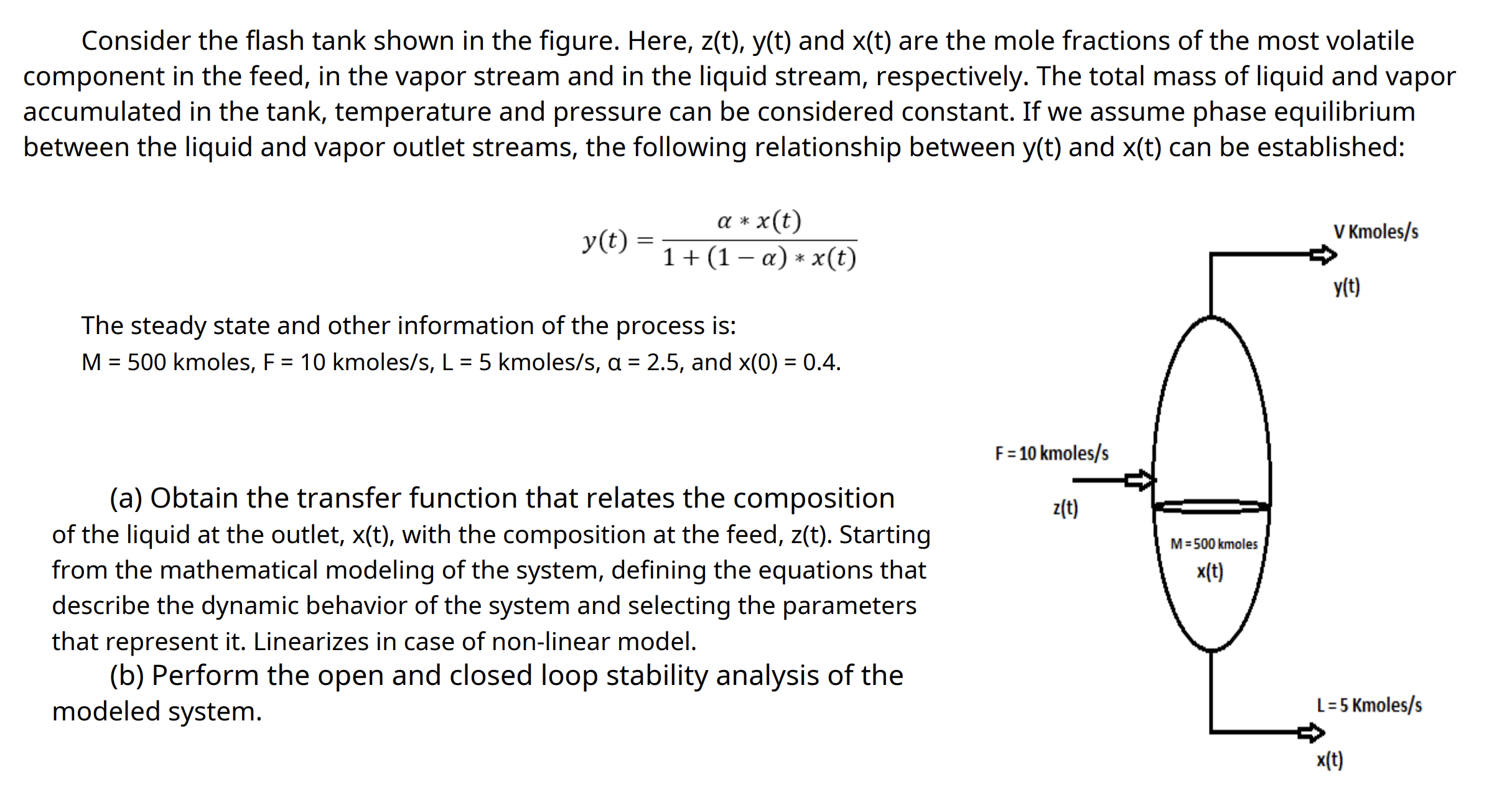
Consider the flash tank shown in the figure. Here, $\mathrm{z}(\mathrm{t}),\mathrm{y}(\mathrm{t})$ and $\mathrm{x}(\mathrm{t})$ are the mole fractions of the most volatile component in the feed, in the vapor stream and in the liquid stream, respectively. The total mass of liquid and vapor accumulated in the tank, temperature and pressure can be considered constant. If we assume phase equilibrium between the liquid and vapor outlet streams, the following relationship between $y(t)$ and $x(t)$ can be established: $y(t)=\frac{\alpha \ast x(t)}{1+(1-\alpha )\ast x(t)}$ The steady state and other information of the process is: $M=500\text{ kmoles, }F=10\text{ kmoles }/\mathrm{s},L=5\text{ kmoles }/\mathrm{s},a=2.5\text{, and }x(0)=0.4\text{. }$ (a) Obtain the transfer function that relates the composition of the liquid at the outlet, $x(t)$, with the composition at the feed, $z(t)$. Starting from the mathematical modeling of the system, defining the equations that describe the dynamic behavior of the system and selecting the parameters that represent it. Linearizes in case of non-linear model. (b) Perform the open and closed loop stability analysis of the modeled system.