(Solved): Consider the case of a skier shown here on an incline. (See section 5.6 of Openstax University Phy ...

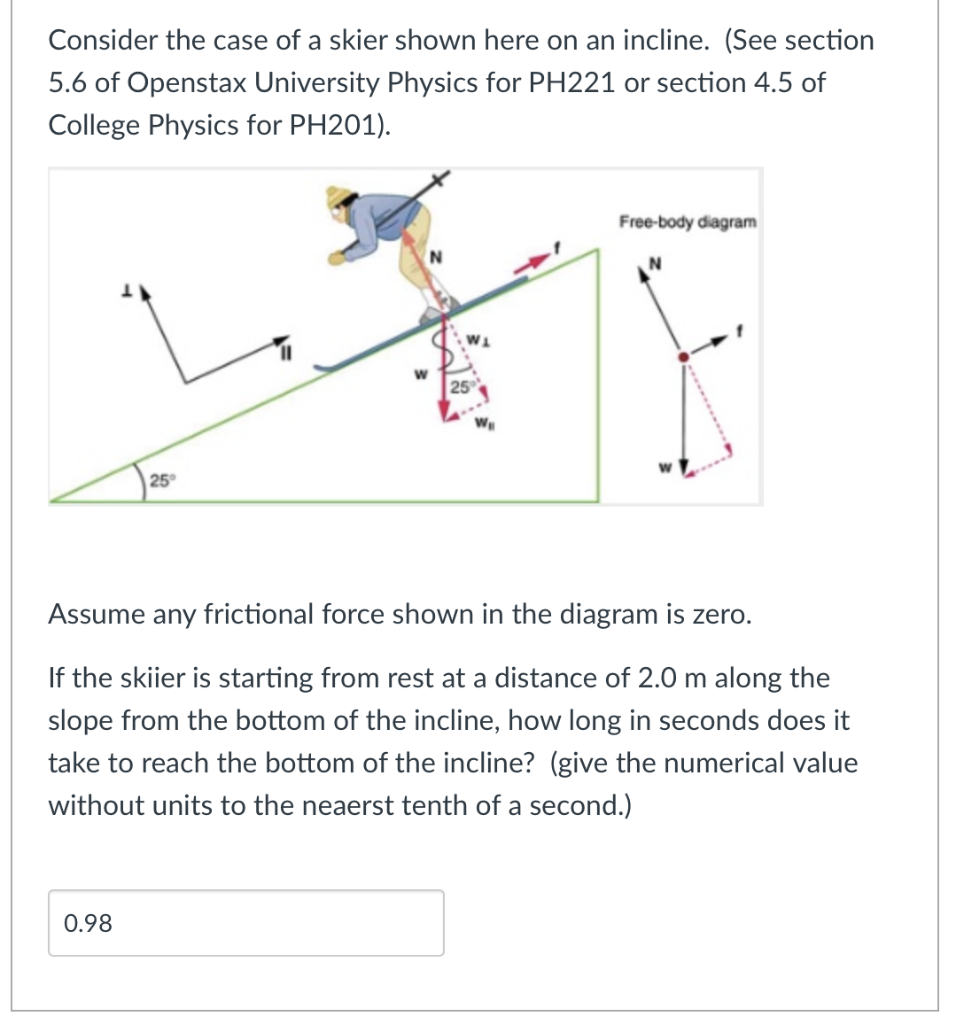
Consider the case of a skier shown here on an incline. (See section 5.6 of Openstax University Physics for \( \mathrm{PH} 221 \) or section \( 4.5 \) of College Physics for \( \mathrm{PH} 201 \) ). Assume any frictional force shown in the diagram is zero. If the skiier is starting from rest at a distance of \( 2.0 \mathrm{~m} \) along the slope from the bottom of the incline, how long in seconds does it take to reach the bottom of the incline? (give the numerical value without units to the neaerst tenth of a second.) Given the same conditions as stated in the previous question, what is the speed of the skier in \( \mathrm{m} / \mathrm{s} \) when reaching the bottom of the slope? (Give the numerical value to the nearest \( 1 / 10 \) of a m/s, as a positive number, even though the direction down the slope may often be chosen as a negative direction.) Considering the skiier again in the above question, suppose the frictional force is non-zero, and consider that we take the direction down the slope as a negative direction, such that any acceleration in that direction is negative. You can say that: The acceleration in this case will be more negative compared to the case with constant acceleration The acceleration in this case will be less negative compared to the case with zero friction The acceleration will not be constant as the skiier moves The friction will make the acceleration zero Suppose the same skier here is at the bottom of the slope, facing uphill (with skis pointing upslope.) Also suppose the skier is given a big push so as to start motion up the slope, with friction acting in the opposite direction as shown in the first question above. Again considering that we take the direction down the slope as negative, we can say that the acceleration will not be constant as the skier moves up the hill the friction will cause the skier to move at constant speed the acceleration is more negative compared to the case with no friction the acceleration is less negative compared to the case of zero friction