(Solved): Consider the aircraft pitch dynamic system indicated below. Also consider the McDonnell Douglas F-4 ...

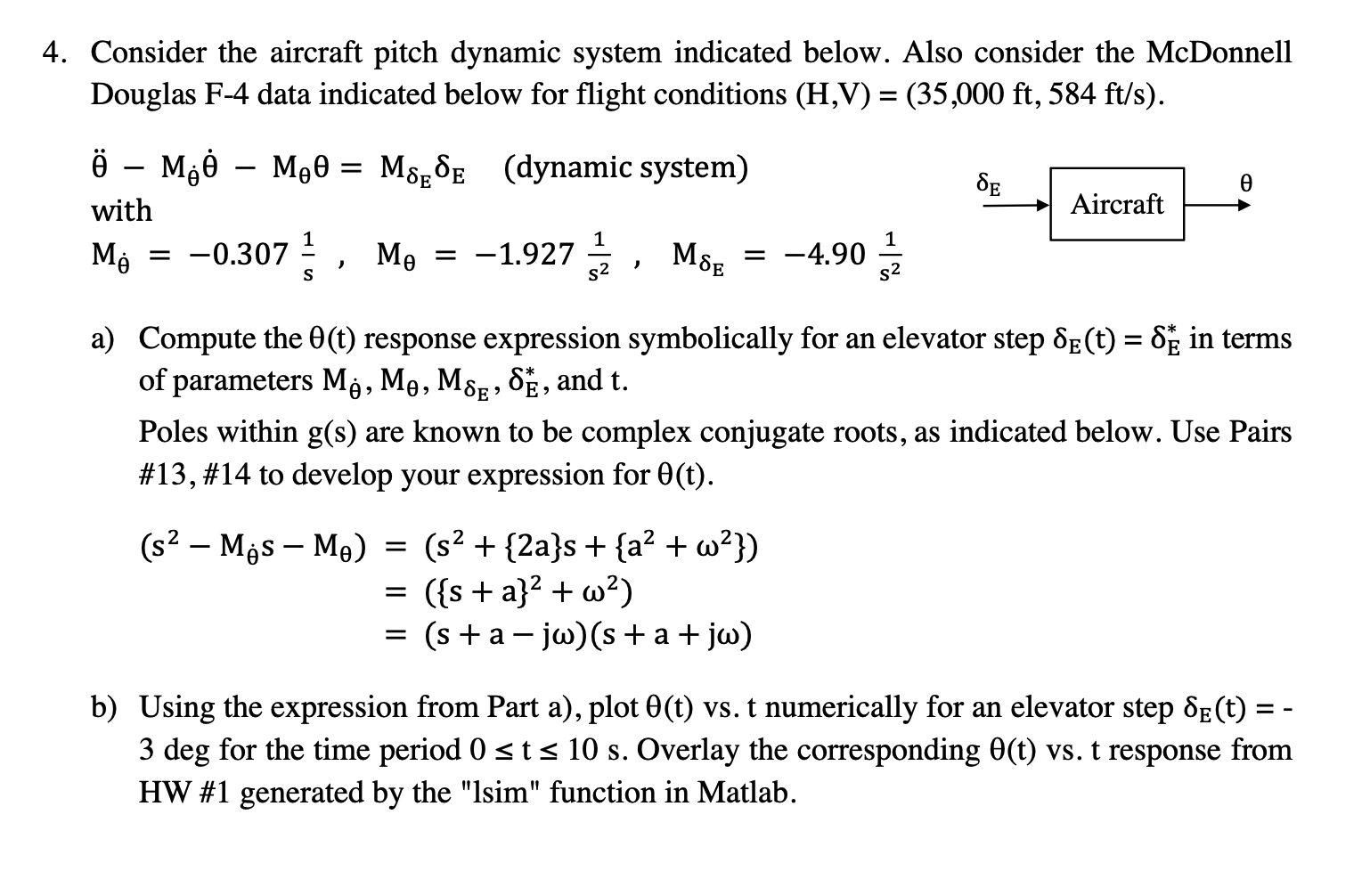
Consider the aircraft pitch dynamic system indicated below. Also consider the McDonnell Douglas F-4 data indicated below for flight conditions $(\mathrm{H},\mathrm{V})=(35,000\mathrm{ft},584\mathrm{ft}/\mathrm{s})$. $\ddot{\theta }-{\mathrm{M}}_{\dot{\theta }}\dot{\theta }-{\mathrm{M}}_{\theta }\theta ={\mathrm{M}}_{{\delta }_{\mathrm{E}}}{\delta }_{\mathrm{E}}$ (dynamic system) with ${\mathrm{M}}_{\dot{\theta }}=-0.307\frac{1}{\mathrm{s}},{\mathrm{M}}_{\theta }=-1.927\frac{1}{{\mathrm{s}}^2},{\mathrm{M}}_{{\delta }_{\mathrm{E}}}=-4.90\frac{1}{{\mathrm{s}}^2}$ a) Compute the $\theta (\mathrm{t})$ response expression symbolically for an elevator step ${\delta }_{\mathrm{E}}(\mathrm{t})={\delta }_{\mathrm{E}}^{\ast }$ in terms of parameters ${\mathrm{M}}_{\dot{\theta }},{\mathrm{M}}_{\theta },{\mathrm{M}}_{{\delta }_{\mathrm{E}}},{\delta }_{\mathrm{E}}^{\ast }$, and $\mathrm{t}$. Poles within $\mathrm{g}(\mathrm{s})$ are known to be complex conjugate roots, as indicated below. Use Pairs $\#13$, \#14 to develop your expression for $\theta (t)$. $\begin{array}{rl}\left(s^2-M_{\dot{\theta }}s-M_{\theta }\right)& =\left(s^2+\{2a\}s+\left\{a^2+{\omega }^2\right\}\right)\\ & =\left(\{s+a{\}}^2+{\omega }^2\right)\\ & =(s+a-j\omega )(s+a+j\omega )\end{array}$ b) Using the expression from Part a), plot $\theta (t)$ vs. $t$ numerically for an elevator step ${\delta }_E(t)=-$ $3\mathrm{deg}$ for the time period $0\le \mathrm{t}\le 10\mathrm{s}$. Overlay the corresponding $\theta (\mathrm{t})$ vs. $t$ response from HW \#1 generated by the "lsim" function in Matlab.