Consider steady one-dimensional heat conduction in a pin fin of constant diameter D with constant thermal conductivity k and surface emissivity epsi . The fin is losing heat by convection to the ambient air at T_( infty ) with a convection coefficient of h, and by radiation to the surrounding surfaces at an average temperature of T_(surr ). The no

Question

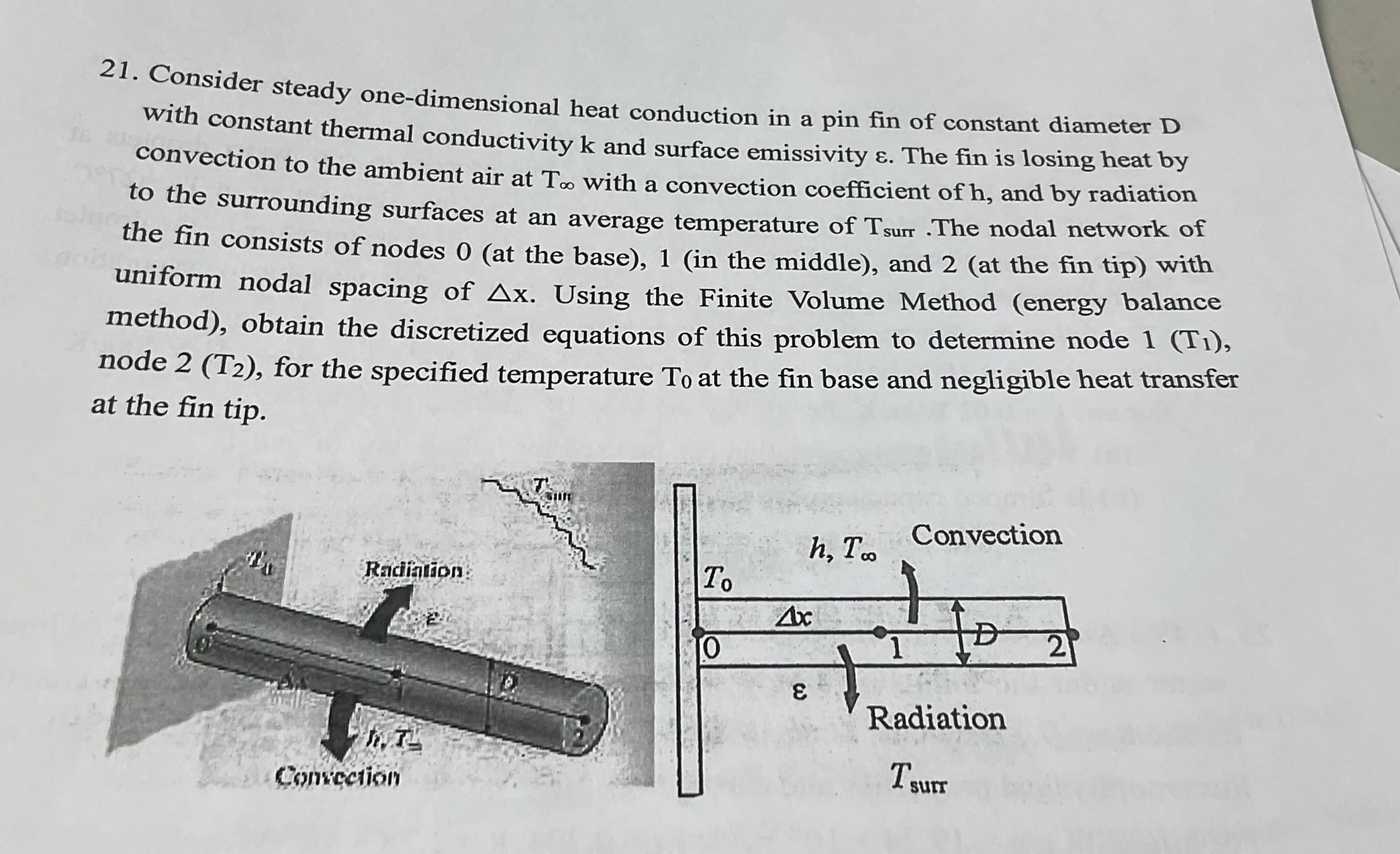
Consider steady one-dimensional heat conduction in a pin fin of constant diameter

D

with constant thermal conductivity k and surface emissivity

\epsi

. The fin is losing heat by convection to the ambient air at

T_(\infty )

with a convection coefficient of

h

, and by radiation to the surrounding surfaces at an average temperature of

T_(surr )

. The nodal network of the fin consists of nodes 0 (at the base), 1 (in the middle), and 2 (at the fin tip) with uniform nodal spacing of

\Delta x

. Using the Finite Volume Method (energy balance method), obtain the discretized equations of this problem to determine node

1(T_(1))

, node

2(T_(2))

, for the specified temperature

T_(0)

at the fin base and negligible heat transfer at the fin tip.

Accepted Answer

Expert Answer to - Consider steady one-dimensional heat conduction in a pin fin of constant diameter D with constant th

Answer

Solution for - Consider steady one-dimensional heat conduction in a pin fin of constant diameter D with constant th

Answer

This an additional answer to - Consider steady one-dimensional heat conduction in a pin fin of constant diameter D with constant th

(Solved): Consider steady one-dimensional heat conduction in a pin fin of constant diameter D with constant th ...