(Solved): Consider an X-ray diffraction experiment using a crystalline powder in the cubic system. Monochrom ...

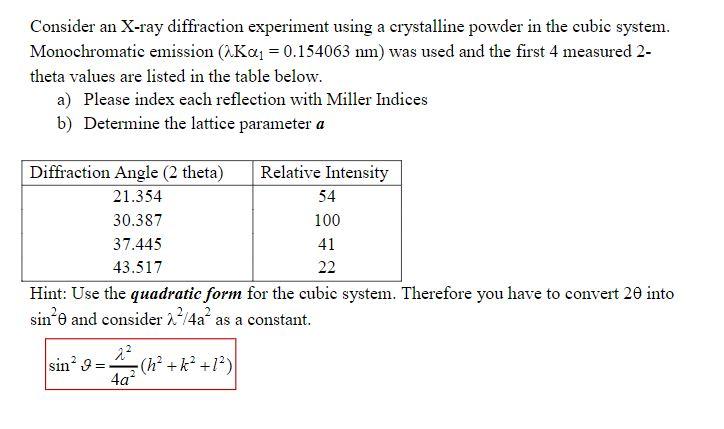
Consider an X-ray diffraction experiment using a crystalline powder in the cubic system. Monochromatic emission \( \left(\lambda \mathrm{K} \alpha_{1}=0.154063 \mathrm{~nm}\right) \) was used and the first 4 measured \( 2- \) theta values are listed in the table below. a) Please index each reflection with Miller Indices b) Determine the lattice parameter \( \boldsymbol{a} \) Hint: Use the quadratic form for the cubic system. Therefore you have to convert \( 2 \theta \) into \( \sin ^{2} \theta \) and consider \( \lambda^{2} / 4 a^{2} \) as a constant. \[ \sin ^{2} \vartheta=\frac{\lambda^{2}}{4 a^{2}}\left(h^{2}+k^{2}+l^{2}\right) \]