(Solved): Consider a cylindrical vessel of radius R which contains water and rotates steadily at angular velo ...

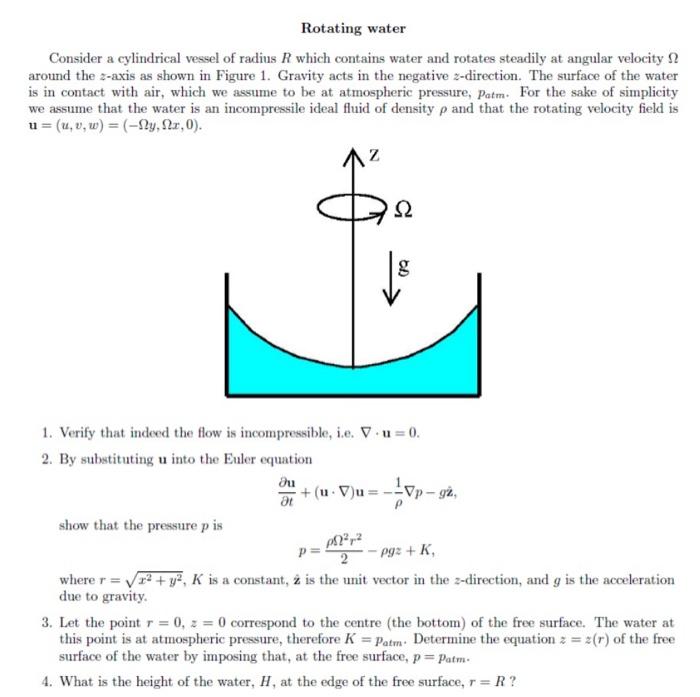
Consider a cylindrical vessel of radius $R$ which contains water and rotates steadily at angular velocity $\Omega$ around the $z$-axis as shown in Figure 1 . Gravity acts in the negative $z$-direction. The surface of the water is in contact with air, which we assume to be at atmospheric pressure, $p_{atm}$. For the sake of simplicity we assume that the water is an incompressile ideal fluid of density $\rho$ and that the rotating velocity field is $\mathbf{u}=(u,v,w)=(-\Omega y,\Omega x,0)$. 1. Verify that indeed the flow is incompressible, i.e. $\nabla \cdot \mathbf{u}=0$. 2. By substituting $\mathbf{u}$ into the Euler equation $\frac{\partial \mathbf{u}}{\partial t}+(\mathbf{u}\cdot \nabla )\mathbf{u}=-\frac{1}{\rho }\nabla p-g\hat{\mathbf{z}},$ show that the pressure $p$ is $p=\frac{\rho {\Omega }^2{r}^2}{2}-\rho gz+K,$ where $r=\sqrt{x^2+y^2},K$ is a constant, $\mathbf{z}$ is the unit vector in the $z$-direction, and $g$ is the acceleration due to gravity. 3. Let the point $r=0,z=0$ correspond to the centre (the bottom) of the free surface. The water at this point is at atmospheric pressure, therefore $K=p_{\mathrm{atm}}$. Determine the equation $z=z(r)$ of the free surface of the water by imposing that, at the free surface, $p=p_{\text{atm }}$. 4. What is the height of the water, $H$, at the edge of the free surface, $r=R$ ?