(Solved): Can someone help me answer the following multiple choice with equations and text given? Given equ ...

Can someone help me answer the following multiple choice with equations and text given?

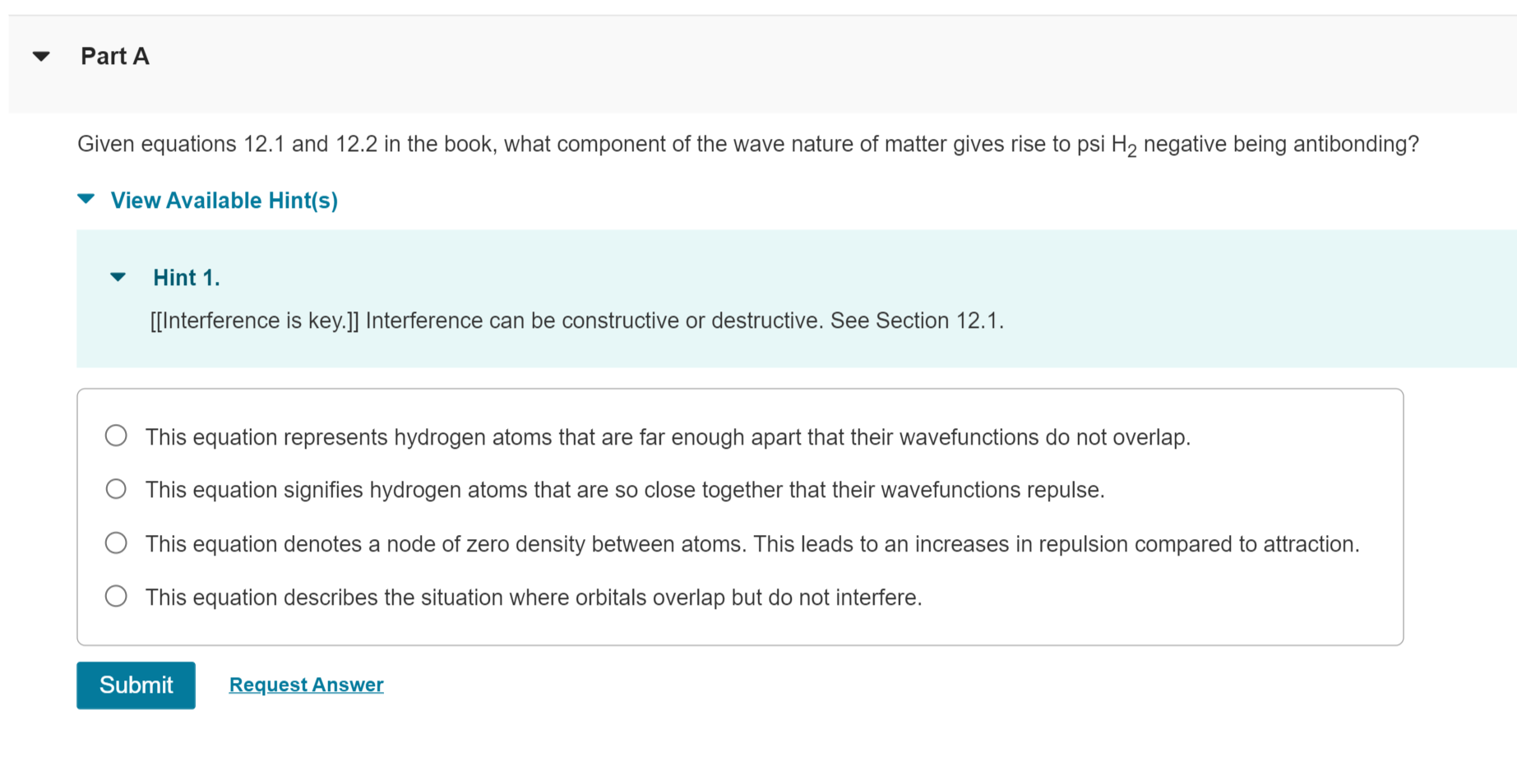
Given equations \( 12.1 \) and \( 12.2 \) in the book, what component of the wave nature of matter gives rise to \( \mathrm{psi}_{2} \) negative being antibonding? View Available Hint(s) Hint 1. [[Interference is key.]] Interference can be constructive or destructive. See Section 12.1. This equation represents hydrogen atoms that are far enough apart that their wavefunctions do not overlap. This equation signifies hydrogen atoms that are so close together that their wavefunctions repulse. This equation denotes a node of zero density between atoms. This leads to an increases in repulsion compared to attraction. This equation describes the situation where orbitals overlap but do not interfere. 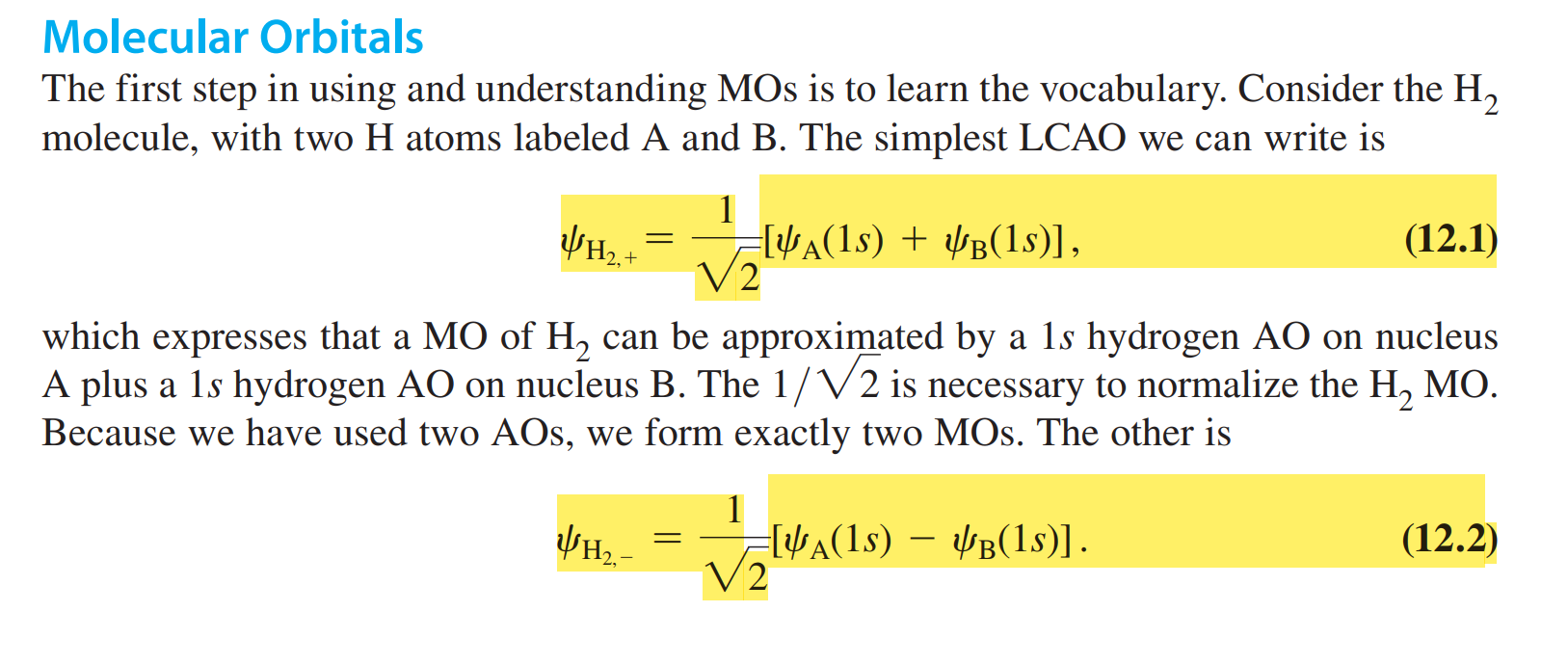Molecular Orbitals The first step in using and understanding MOs is to learn the vocabulary. Consider the \( \mathrm{H}_{2} \) molecule, with two \( \mathrm{H} \) atoms labeled A and B. The simplest LCAO we can write is \[ \psi_{\mathrm{H}_{2,+}}=\frac{1}{\sqrt{2}}\left[\psi_{\mathrm{A}}(1 s)+\psi_{\mathrm{B}}(1 s)\right], \] which expresses that a \( \mathrm{MO} \) of \( \mathrm{H}_{2} \) can be approximated by a \( 1 s \) hydrogen \( \mathrm{AO} \) on nucleus A plus a \( 1 s \) hydrogen \( \mathrm{AO} \) on nucleus \( \mathrm{B} \). The \( 1 / \sqrt{2} \) is necessary to normalize the \( \mathrm{H}_{2} \) MO. Because we have used two AOs, we form exactly two MOs. The other is \[ \psi_{\mathrm{H}_{2,-}}=\frac{1}{\sqrt{2}}\left[\psi_{\mathrm{A}}(1 s)-\psi_{\mathrm{B}}(1 s)\right] \] We can calculate the electron distributions corresponding to these MOs by squaring them, and we can calculate their energy levels by using the Hamiltonian operator for an \( \mathrm{H}_{2} \) molecule. Equations \( 12.1 \) and \( 12.2 \) give approximations to the wavefunctions of \( \mathrm{H}_{2} \). To calculate reasonable values for the electronic energies of \( \mathrm{H}_{2} \), much more complicated wavefunctions would be needed. Nevertheless, MOs that are LCAOs are useful concepts to understand qualitatively electron distributions in molecules. Show that the molecular wavefunction proposed in Eq. \( 12.1 \) is normalized, taking the atomic wavefunctions of distinct nuclei to be orthogonal, \( \int \psi_{A}^{*} \psi_{B}=\int \psi_{B}^{*} \psi_{A}=0 \) and the individual wavefunctions are also normalized. SOLUTION Form the product \( \psi^{*} \psi \) and then evaluate the integral over all space. A common notational shortcut is to write \( d \tau \) as a generic symbol for all three variables of integration since we have not specified the coordinate system (cartesion, polar, other): \[ \begin{aligned} \int \frac{1}{\sqrt{2}} & {\left[\psi_{A}^{*}(1 s)+\psi_{B}^{*}(1 s)\right] \frac{1}{\sqrt{2}}\left[\psi_{A}(1 s)+\psi_{B}(1 s)\right] d \tau } \\ &=\frac{1}{2} \int\left[\psi_{A}^{*}(1 s) \psi_{A}(1 s)+\psi_{A}^{*}(1 s) \psi_{B}(1 s)+\psi_{B}^{*}(1 s) \psi_{A}(1 s)+\psi_{B}^{*}(1 s) \psi_{B}(1 s)\right] d \tau \\ &=\frac{1}{2}[1+0+0+1]=1 \end{aligned} \] Practice: Now show that Eq. \( 12.2 \) is also a normalized molecular wavefunction. "Caution: in the LCAO-MO treatment of \( \mathrm{H}_{2} \), Eqs. \( 12.1 \) and \( 12.2 \) would be modified, as the separate atomic orbitals are not orthogonal, and indeed define the degree of overlap of the separate atomic orbitals (see question 12.12). Equations \( 12.1 \) and \( 12.2 \) are examples of sigma \( (\sigma) \) and sigma star \( \left(\sigma^{*}\right) \mathrm{MOs} \), respectively, where the names indicate symmetry properties. A \( \sigma \) bonding molecular orbital has cylindrical symmetry of the electron density around a line joining the nuclei and has no node between the nuclei. Bonding orbitals are stabilized by having electron density enhanced between the two positively charged nuclei. A \( \sigma^{*} \) antibonding molecular orbital also has cylindrical symmetry around the line joining the nuclei but has a node between the nuclei. Figures \( 12.1 \) and \( 12.2 \) illustrate \( \sigma \) and a \( \sigma^{*} \) MOs constructed for the \( \mathrm{H}_{2} \) molecule. The energy calculated for a \( \sigma \mathrm{MO} \) is less (more negative) than the sum of the energies for the two AOs; the \( \sigma^{*} \) MO energy is greater than the sum of the two AOs.