(Solved): (Algebraic properties of the dot product) Assume all vectors are in R3. Let a and b be fixed vector ...

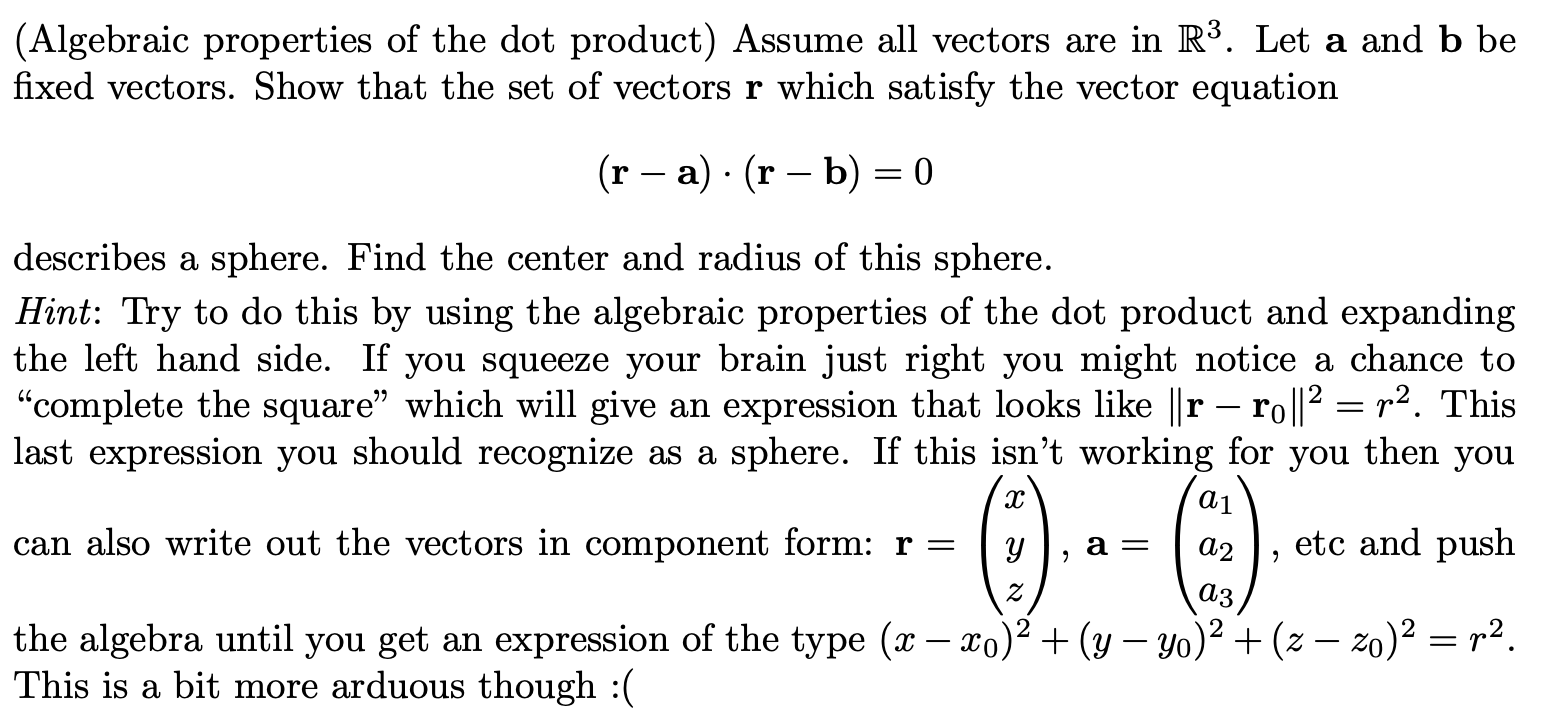
(Algebraic properties of the dot product) Assume all vectors are in ${\mathbb{R}}^3$. Let $\mathbf{a}$ and $\mathbf{b}$ be fixed vectors. Show that the set of vectors $\mathbf{r}$ which satisfy the vector equation $(\mathbf{r}-\mathbf{a})\cdot (\mathbf{r}-\mathbf{b})=0$ describes a sphere. Find the center and radius of this sphere. Hint: Try to do this by using the algebraic properties of the dot product and expanding the left hand side. If you squeeze your brain just right you might notice a chance to "complete the square" which will give an expression that looks like ${\Vert \mathbf{r}-{\mathbf{r}}_0\Vert }^2=r^2$. This last expression you should recognize as a sphere. If this isn't working for you then you can also write out the vectors in component form: $\mathbf{r}=\left(\begin{array}{l}x\\ y\\ z\end{array}\right),\mathbf{a}=\left(\begin{array}{l}{a}_1\\ a_2\\ a_3\end{array}\right)$, etc and push the algebra until you get an expression of the type ${\left(x-x_0\right)}^2+{\left(y-y_0\right)}^2+{\left(z-z_0\right)}^2=r^2$. This is a bit more arduous though :(